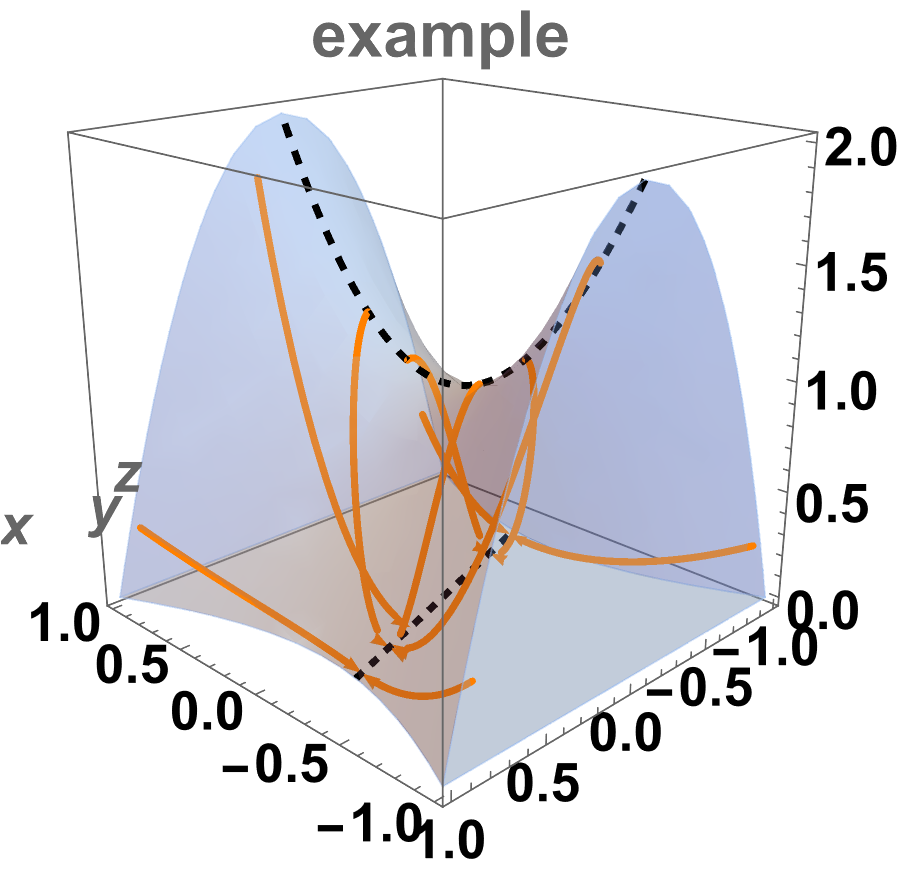
I am using the StreamPlot3D function from Wolfram Mathematica for the following system

1. $x'=\left(-x^2-1\right) (3 x+y)+3 x z$
2. $y'= 3 y z-x y (3 x+y)$
3. $z'= z (6 (z-1)-2 x (3 x+y)),$

with the following restriction $-x^2+2y^2+z=1.$

I am using the RegionFunction option of StreamPlot3D to plot on the inside of the restriction, that is $-x^2+2y^2+z\leq 1$ or RegionFunction -> Function[{x, y, z}, -x^2 + 2 y^2 + z <= 1]

Is there a way to plot the lines on the surface of the restriction i.e. the hyperbolic paraboloid?

I don't see any way to do it in the documentation or in the help for the StreamPlot3D function. I tried defining the region function to ==1 but it does not work. The closest thing i have seen is this link: Mapping StreamPlot onto spherical surfaces

But they go from a 2D system to a 3D projection on a sphere, I do have a 2D version of this system solving the restriction for z and I have 2D plots for this but I cannot define the quadric surface properly to make it work like in that example.

Thanks in advance for the help.