Return to Revisions

You may use the NDSolve Components and Data Structures tutorial to control the memory usage and save down the state of intermediate runs.

Initialise the NDSolve`StateData for the complete range you need to create solutions. Below is done for 0 <= t <= 30 and I will iterate in chunks of 10.

ndsStateData = First@NDSolve`ProcessEquations[
    {
     D[u[t, x], t] == D[u[t, x], x, x],
     u[0, x] == 0,
     u[t, 0] == Sin[t],
     u[t, 5] == 0
     },
    u,
    {t, 0, 30}, {x, 0, 5}
    ];

Next I iterate in 3 chunks of 10. ndsStateData can be saved down after the current chunk's solution is extracted with NDSolve`ProcessSolutions. Below I reassign ndsStateData with the reinitialised NDSolve`StateData instead.

sols = {};
Module[{step = #},
    NDSolve`Iterate[ndsStateData, {(step - 1)*10, step 10}];
    AppendTo[sols, u /. NDSolve`ProcessSolutions[ndsStateData]];
    ndsStateData =
     First@NDSolve`Reinitialize[ndsStateData, {u[step 10, x] == sols[[step]][step 10, x]}];
    ] & /@ Range@3;

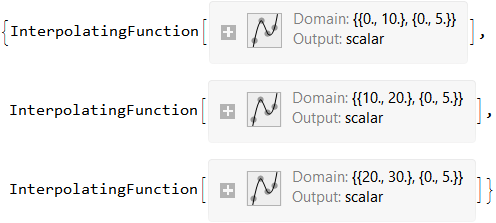
sols contains the 3 solutions over each chunk. Notice the difference in the domains.

sols

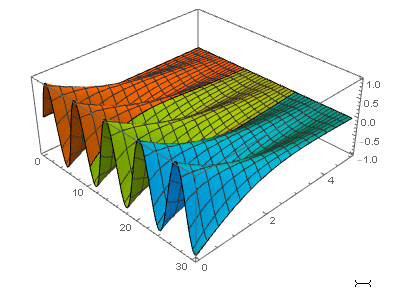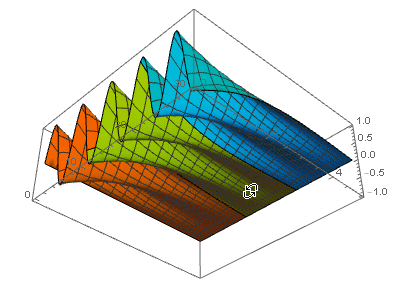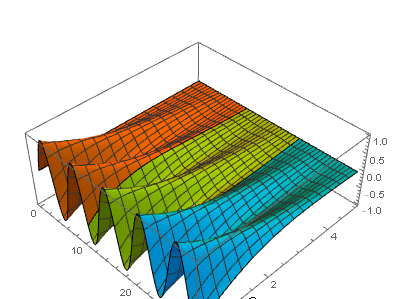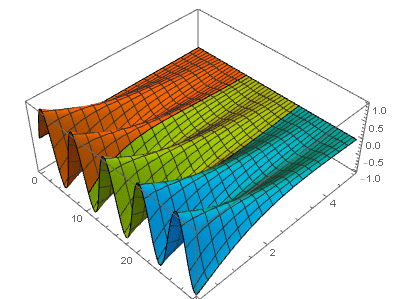
Plotting the solutions.

Plot3D[sols[[#]][t, x], {t, (# - 1) 10, # 10}, {x, 0, 5},
    PlotRange -> Full,
    PlotStyle -> ColorData[109][#]
    ] & /@ Range@3 // Show[#, PlotRange -> All] &

Hope this helps.