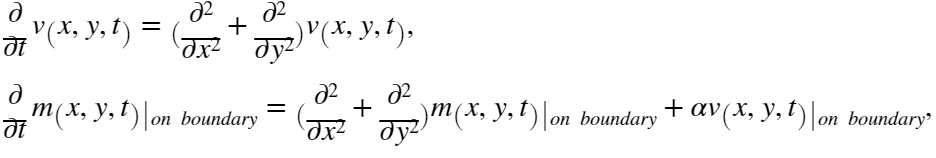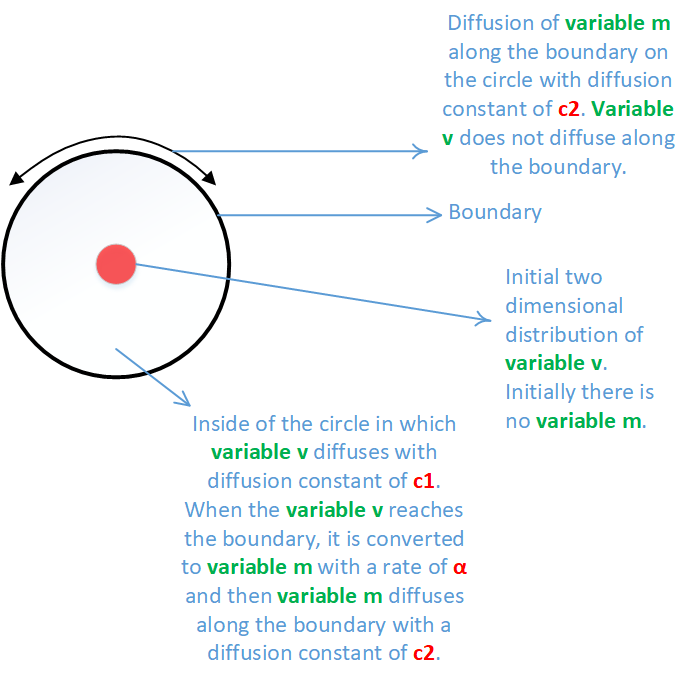
I want to solve two coupled partial differential equations on two dimension. There are two variables v and m. The geometry is a disk. The variable v diffuses inside the disk until it reaches the boundary and then it converts to variable m. Variable m then diffuses on the boundary, on the edge of the disk. Variable m does not exist inside the disk, it only exists on the boundary. In the diagram below you see the summary of the problem:

I use the set of equations below to define the problem:

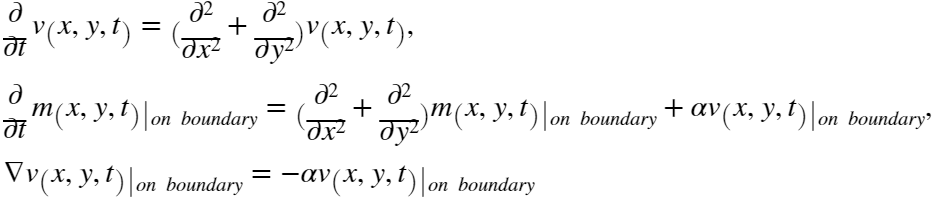
The first equation describes the diffusion of variable v inside the disk. The second equation describes the conversion of variable v to variable m (the term alpha*v(x,y,t)) and the diffusion of variable m on the edge of the disk. The last equation is the boundary condition at the boundary of the disk which accounts for the conversion of variable v to variable m. On the left ∇ is the gradient operator which indicates the flux of variable v on the boundary.

My problem is that how I should tell Mathematica that in the system of equations below (also shown above before) the first equation applies to the disk and the second equation applies to the edge of the disk (the boundary)? The way I solved it below, the value of variable m is calculated on the entire of the disk which is not desired. m has value only on the boundary while it diffuses there.

Here is the code in Mathematica:

alpha = 1.0;
geometry = Disk[];

sol = NDSolveValue[{D[v[x, y, t], t] == 
     D[v[x, y, t], x, x] + D[v[x, y, t], y, y] + 
      NeumannValue[-1*alpha*v[x, y, t], x^2 + y^2 == 1],
    D[m[x, y, t], t] == 
     D[m[x, y, t], x, x] + D[m[x, y, t], y, y] + alpha*v[x, y, t], 
    m[x, y, 0] == 0, v[x, y, 0] == Exp[-((x^2 + y^2)/0.01)]}, {v, 
    m}, {x, y} \[Element] geometry, {t, 0, 10}];

v = sol[[1]];
m = sol[[2]];

ContourPlot[v[x, y, 1], {x, y} \[Element] geometry, PlotRange -> All, 
 PlotLegends -> Automatic]

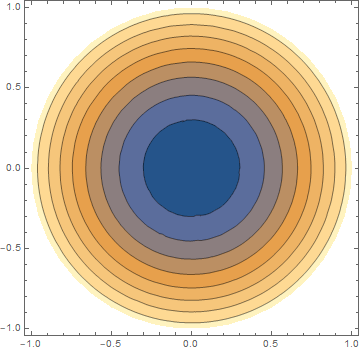
ContourPlot[m[x, y, 10], {x, y} \[Element] geometry, PlotRange -> All,
  PlotLegends -> Automatic]

Adding DirichletCondition[m[x, y, t] == 0, x^2 + y^2 < 1] to enforce the value of m inside the geometry (here the disk) gives this error:

NDSolveValue::bcnop: No places were found on the boundary where x^2+y^2<1 was True, so DirichletCondition[m==0,x^2+y^2<1] will effectively be ignored.