Fancy 3D Graphics

Question

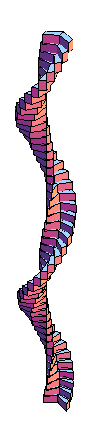
I define following functions:

helix[a_, b_][t_] := {a*Cos[t], a*Sin[t], b*t}
listept = Table[helix[0.35, 0.35][t], {t, 0, 4 Pi, .25}];
bezierint = Graphics3D[Cuboid[{#, # + 0.1}] & /@ listept]
tube = Graphics3D[{Opacity[0.25], RGBColor[1, 3, 0], 
 Cylinder[{{0, 0, 0}, {0, 0, 1.55 Pi}}, 0.75]}]
Show[{bezierint, tube}]

and now I would like to substitute each ordinary Cuboid defined in bezierint with fancy Cuboid defined as follow:

box1 = GeometricTransformation[Cuboid[{0, 0, 0}], ShearingMatrix[Pi/4, {1,0, 0}, {-1, 1, 0}]]
box2 = GeometricTransformation[Cuboid[{1, 1, 0}], 
 ShearingMatrix[-Pi/4, {1, 0, 0}, {-1, 1, 0}]]
Graphics3D@{box1, box2}

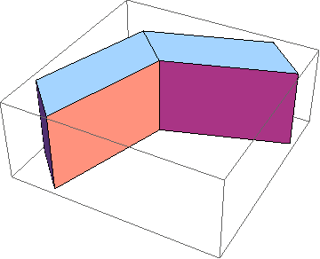
simulateneously mentaining the repeatability of the pattern.

I have used many approaches but up to now all were unsuccesful. Did anyone had to deal before with such task?

Answer 1

Perhaps:

gt  = GeometricTransformation;
box = gt[{box1, box2}, ScalingTransform[.1 {1, 1, 1}]];
cc[{x_, y_, z_}] := gt[gt[box, RotationTransform[{{1, 1, 0}, {x, y, 0}}]], 
                       TranslationTransform[{0, 0, z}]]

Graphics3D[cc /@ listept]

---

Edit

A small generalization, just for fun:

gt = GeometricTransformation;
box = gt[{box1, box2}, ScalingTransform[.1 {1, 1, 1}]];
cg[{x_, y_, z_}, pat_] := gt[gt[box, RotationTransform[{{1, 1, 0}, pat[x, y, z]}]], 
                            TranslationTransform[{0, 0, z}]]
gf[pat_] := Graphics3D[cg[#, pat] & /@ listept]

gf /@ {Function[{a, b, c}, {a, b, 0}], 
       Function[{a, b, c}, {a, b, Sin@c}], 
       Function[{a, b, c}, {a, -b, c^2}],
       Function[{a, b, c}, {a^2, b, 0}]}