How to speed up the plotting of B-spline curve?

Question

Three months ago, I asked a quesion about B-Spline basis function here, Today, I used this function to plot B-spline curve.

The definition of $N_{i,p}$

  NBSpline[i_Integer, 0, knots_?(VectorQ[#, NumericQ] && OrderedQ[#] &),u_] /;
   i <= Length[knots] - 2 :=
  Piecewise[
   {{1, knots[[i + 1]] <= u < knots[[i + 2]]},
    {0, u < knots[[i + 1]] || u >= knots[[i + 2]]}}]

  coeff[u_, i_, j_, knots_] /; knots[[i]] == knots[[j]] := 0;
  coeff[u_, i_, j_, knots_] := (u - knots[[i]])/(knots[[j]] - knots[[i]])

  NBSpline[i_Integer, p_Integer, knots_?(VectorQ[#, NumericQ] && OrderedQ[#] &), 
    u_] /;p > 0 && i + p <= Length[knots] - 2 :=
   Module[{init, res},
    init = Table[NBSpline[j, 0, knots, u], {j, i, i + p}];
    res = First@
    Nest[
     Dot @@@
      (Thread@
        {Partition[#, 2, 1],
         With[{m = p + 2 - Length@#},
          Table[
           {coeff[u, k + 1, k + m + 1, knots],
            coeff[u, k + m + 2, k + 2, knots]}, {k, i, i + Length@# - 2}]]}) &,
    init, p]
  ]

---

The definition of B-Spline curve

$$\overset{\rightharpoonup }{C}(u)=\sum _{i=0}^n N_{i,p}(u) \overset{\rightharpoonup }{P}_i \text{ }\qquad (a\leq u\leq b)$$

where, $P_i$ is the control point, the $N_ {i, p} (u)$ are the pth - degree Bspline basis functions defined on the nonperiodic (and nonuniform) knot vector

knots= $\{\underbrace {a,\cdots ,a}_{p+1},u_{p+1},\cdots u_{m-p-1},\underbrace {b,\cdots,b}_{p+1}\}$

---

Trail 1

(Update) with george2079's solution

 BSplinePlot1[pts : {{_, _} ..}, knots_, opts : OptionsPattern[Plot]] :=
 Module[{p = Length@First@Split[knots] - 1, a, b},
  {a, b} = {First[knots], Last[knots]};
  ParametricPlot[
  Evaluate@
  Simplify@
    Total@ 
     MapIndexed[
      NBSpline[First@#2 - 1, p, knots, u] #1 &, pts], {u, a, b}, opts
 ]
]

Test1

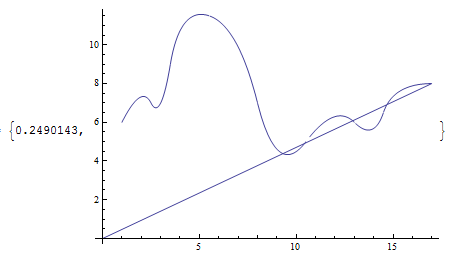
 pts3 = {{1, 6}, {2, 8}, {3, 6}, {4, 12}, {7, 11}, {9, 3}, {12, 7}, {14, 5}, {15, 8}, {17, 8}};
 knots3= {0, 0, 0, 1/8, 2/8, 3/8, 4/8, 5/8, 6/8, 7/8, 1, 1, 1};

 BSplinePlot1[pts3, knots3, ImageSize -> 600]

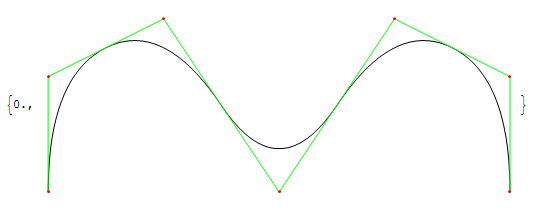
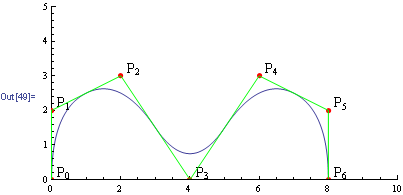
 Graphics[{BSplineCurve[pts, SplineKnots -> knots], Green, Line[pts], 
  Red, Point[pts]}] // AbsoluteTiming

---

Update

• Is there any method to speed up the calculation of NBSPline?

  See george2079's solution and my answer

• How to deal with the problem of discontinuity shown in the first graph?

  Add the option PlotPoints

Answer 1

The plot is sped up substantially if you use Evaluate:

  ParametricPlot[
      Evaluate[ Total@MapIndexed[NBSpline[First@#2 - 1, p, knots, u] #1 &, pts]] ,
           {u, a, b}, opts]

(I only looked a trial 1 , but I think your other try have the same issue )

It helps a little more if you remove the Simplify from NBSpline and simplify the whole thing:

 ParametricPlot[
       Evaluate[Total@
             MapIndexed[NBSpline[First@#2 - 1, p, knots, u] #1 &, pts] // 
                  Simplify], {u, a, b}, opts]

Your original form is a sum of piecewise expressions. The outer Simplify condenses the whole thing into a single piecewise.

The gaps seem to relate the nature of the discontinuity in derivatives of the bspline w/ respect to its parameter at the knots, which evidently fools Parametric Plot into thinking there is an actual discontinuity.

The gaps close with PlotPoints -> 1000 , though if you look at the graphics produced you'll see you still have separate Lines for each portion. I don't think there is anything to do about that except not use ParametricPlot.

You might try doing away with ParametricPlot and doing Graphics@Line@Table .., which may speed it up as well.

Answer 2

Re: your error

BSplinePlot2[pts : {{_, _} ..}, knots_, opts : OptionsPattern[{Plot, BSplinePlot}]] := 
 Module[{p = Length@First@Split[knots] - 1, a, b}, 
  {a, b} = {First[knots], Last[knots]};
  With[{a1 = a, b1 = b}, 
   ParametricPlot[
    Total@MapIndexed[NBSpline[First@#2 - 1, p, knots, u] #1 &, pts], {u, a1, b1},
    Evaluate[Sequence @@ FilterRules[{opts}, Options[Plot]]], 
    Epilog -> If[OptionValue[ShowPoints], 
                Join[Text @@@ (Thread@{Table[ Style[Subscript["P", i], 14], {i, 0, Length@pts - 1}], 
                     # + {.4, .2} & /@ pts}), {Red,PointSize[0.015],Point[pts], Green, Line[pts]}]]]]]

pts = {{0, 0}, {0, 2}, {2, 3}, {4, 0}, {6, 3}, {8, 2}, {8, 0}};
knots = {0, 0, 0, 1/5, 2/5, 3/5, 4/5, 1, 1, 1}; 
BSplinePlot2[pts, knots, PlotRange -> {{0, 10}, {0, 5}}, ShowPoints -> True]