Problem with Neumann condition in quarter disc

Question

Bug introduced in 10.0 and fixed in 10.2

---

So I'm following the available examples in version 10 for FEM, The plane stress operator is shown as this

op0 = {Inactive[
       Div][({{0, -((Y ν)/(1 - ν^2))}, {-((Y (1 - ν))/(
           2 (1 - ν^2))), 0}}.Inactive[Grad][
         v[x, y], {x, y}]), {x, y}] + 
     Inactive[
       Div][({{-(Y/(1 - ν^2)), 
          0}, {0, -((Y (1 - ν))/(2 (1 - ν^2)))}}.Inactive[
          Grad][u[x, y], {x, y}]), {x, y}], 
    Inactive[
       Div][({{0, -((Y (1 - ν))/(2 (1 - ν^2)))}, {-((
           Y ν)/(1 - ν^2)), 0}}.Inactive[Grad][
         u[x, y], {x, y}]), {x, y}] + 
     Inactive[
       Div][({{-((Y (1 - ν))/(2 (1 - ν^2))), 
          0}, {0, -(Y/(1 - ν^2))}}.Inactive[Grad][
         v[x, y], {x, y}]), {x, y}]}

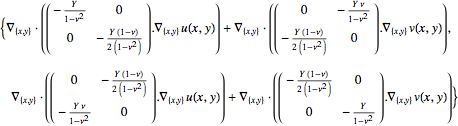
 op = op0 /. {Y -> 10^3, ν -> 33/100};

My goal is to model a disc with pressure in the outer surface. I placed a boundary condition that reduced the computational domain to the upper quarter of the plane:

Subscript[Γ, D] = { 
  DirichletCondition[{u[x, y] == 0.}, x == 0], 
  DirichletCondition[{ v[x, y] == 0.0}, y == 0]}

ℛ = 
  ParametricRegion[{{s, t}, 
    Sqrt[s^2 + t^2] <= 10.0 && 0 <= t <= 10.0 && 0 <= s <= 10.0}, {s, 
    t}];

dd = DiscretizeRegion[ℛ]

At this point I get as expected, a mesh in the upper quarter plane of the disc of radius 10.0

Now I try to solve the system applying some Neumann condition in the outer radius, but apparently something about it is failing it:

{uif, vif} = 
  NDSolveValue[{op == {
       NeumannValue[-1.3*(x/10.0), Sqrt[x^2 + y^2] >= 9.9], 
       NeumannValue[-1.3*(y/10.0), Sqrt[x^2 + y^2] >= 9.9]}, 
    Subscript[Γ, D]}, {u, v}, Element[{x, y}, dd]];

I get

Power::infy: Infinite expression 1/Sqrt[0.] encountered. >>
Infinity::indet:Indeterminate expression 0. ComplexInfinity encountered. >>
CompiledFunction::cfta: Argument {Boole[-9.9>=Indeterminate],0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,0,<<14>>} at position 1 should be a rank 1 tensor of machine-size integers. >>
CompiledFunction::cfta: Argument {Boole[-9.9>=Indeterminate],0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,0,<<14>>} at position 1 should be a rank 1 tensor of machine-size integers. >>`

Answer 1

Apparently NeumannValue doesn't like the square root, replacing it with the squared expression works correctly:

NeumannValue[1000.3* (x/10.0), x^2 + y^2 >= 99.9]