Graph and Markov Chain

Question

I have the following code:

proc = DiscreteMarkovProcess[1, {{0.6, 0.4}, {0.3, 0.7}}]

Graph[{"A", "E"}, proc, GraphStyle -> "DiagramBlue"]

How can I 'force' to show the percentage values (0.6, 0.4 etc) on each arrow? I can see the values when I mouse hover it. Thank you.

Answer 1

proc = DiscreteMarkovProcess[1, {{0.6, 0.4}, {0.3, 0.7}}]
h = Graph[{"A", "E"}, proc, GraphStyle -> "DiagramBlue"]
ToExpression@StringReplace[ToString@FullForm@h, "Tooltip" :> ".1"]

Answer 2

Update: Getting EdgeLabels from proc and using them directly in Graph:

Graph[vl = {"A", "E"}, proc, GraphStyle -> "DiagramBlue", 
 EdgeLabels -> Flatten[MapIndexed[DirectedEdge @@ (#2 /. MapIndexed[#2[[1]]
   -> # &, vl]) -> # &, proc[[2]], {2}]]]

Using MarkovProcessProperties[proc, "TransitionMatrix"] in place of proc[[2]] gives the same result.

---

Original answer:

proc = DiscreteMarkovProcess[1, {{0.6, 0.4}, {0.3, 0.7}}];
g = Graph[{"A", "E"}, proc, GraphStyle -> "DiagramBlue"];

SetProperty[g, Sequence @@ (AbsoluteOptions[g, EdgeLabels] /. Tooltip -> 1/2)]
(* or Tooltip -> Automatic *)

Few more alternatives -- all give the same picture

g1 = Graph[{"A", "E"}, proc, GraphStyle -> "DiagramBlue", 
           EdgeLabels -> {e_ :> PropertyValue[{g1, e}, "Probability"]}]

Or

g2 = Graph[{"A", "E"}, proc];
edgeprobs = (Join @@ {# -> PropertyValue[{g2, #}, "Probability"]} & /@ EdgeList[g2]); 
Graph[{"A", "E"}, proc, GraphStyle -> "DiagramBlue",  EdgeLabels -> edgeprobs]

Or

g3 = Fold[SetProperty[{#, #2},
                EdgeLabels -> PropertyValue[{#, #2}, "Probability"]] &,
          g3, EdgeList[g3]]

An alternative way to get the probabilities associated with edges:

props = Properties /. AbsoluteOptions @ g
(* {"E" \[DirectedEdge] "E" -> {"Probability" -> 0.7}, 
    "E" \[DirectedEdge] "A" -> {"Probability" -> 0.3}, 
    "A" \[DirectedEdge] "E" -> {"Probability" -> 0.4}, 
    "A" \[DirectedEdge] "A" -> {"Probability" -> 0.6}} *)

props /. {"Probability" -> a_} :> a
(* {"E" \[DirectedEdge] "E" -> 0.7, 
    "E" \[DirectedEdge] "A" -> 0.3, 
    "A" \[DirectedEdge] "E" -> 0.4, 
    "A" \[DirectedEdge] "A" -> 0.6} *)

Answer 3

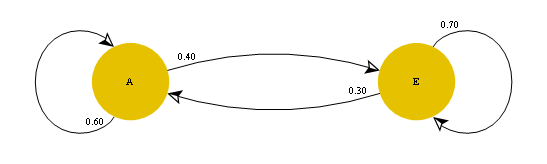
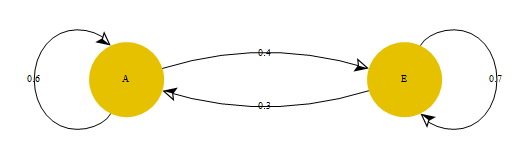
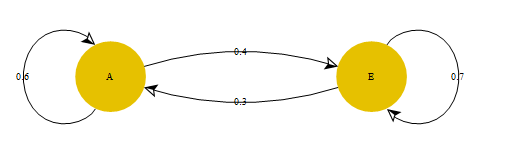
From the documentation for DiscreteMarkovProcess, you can come up with this:

Graph[proc, GraphStyle -> "DiagramBlue", 
 EdgeLabels -> 
  With[{sm = MarkovProcessProperties[proc, "TransitionMatrix"]}, 
   Flatten@Table[DirectedEdge[i, j] -> sm[[i, j]], {i, 2}, {j, 2}]],
 VertexLabels -> {1 -> "A", 2 -> "E"}
]

I took the liberty of changing how the vertices are labeled (otherwise the edge label commands would become unnecessarily further complicated).

Considering that's how it's done in the documentation, I suspect this is the cleanest way to do it canonically. There may be a better hack that might pick apart the Markov process data structure and piece together a graph, but this is how Mathematica devs seem to have intended it.

SECOND VERSION

Based on comments following the question, perhaps a different approach would help.

This time I will try to be "constructive" in some sense. Let's start with the probabilities:

proc = {{0.6, 0.4}, {0.3, 0.7}};

Now, you want to create your edge labels as a table. Every individual edge label will be of the form:

DirectedEdge[2,2] -> 0.7

Since that is the (2,2) entry in your matrix of probabilities. To construct that table:

edge = Table[DirectedEdge[i, j] -> prob[[i, j]],
 {i, 1, Length[prob]}, {j, 1, Length[prob[[i]]]}];

Here we use Length[prob] etc. to make sure our indices are right, but you can also just do:

edge = Table[DirectedEdge[i, j] -> prob[[i, j]], {i, 1, 2}, {j, 1, 2}];

That list, however, is still in an array/matrix format. So we flatten it:

edge = Flatten[edge];

Finally, we need some vertex labels to go with it:

vert = {1 -> "A", 2 -> "E"};

And now we are done:

proc = DiscreteMarkovProcess[1, prob];
Graph[proc, VertexLabels -> vert, EdgeLabels -> edge, GraphStyle -> "DiagramBlue"]