How to plot some special coordinate lines?

Question

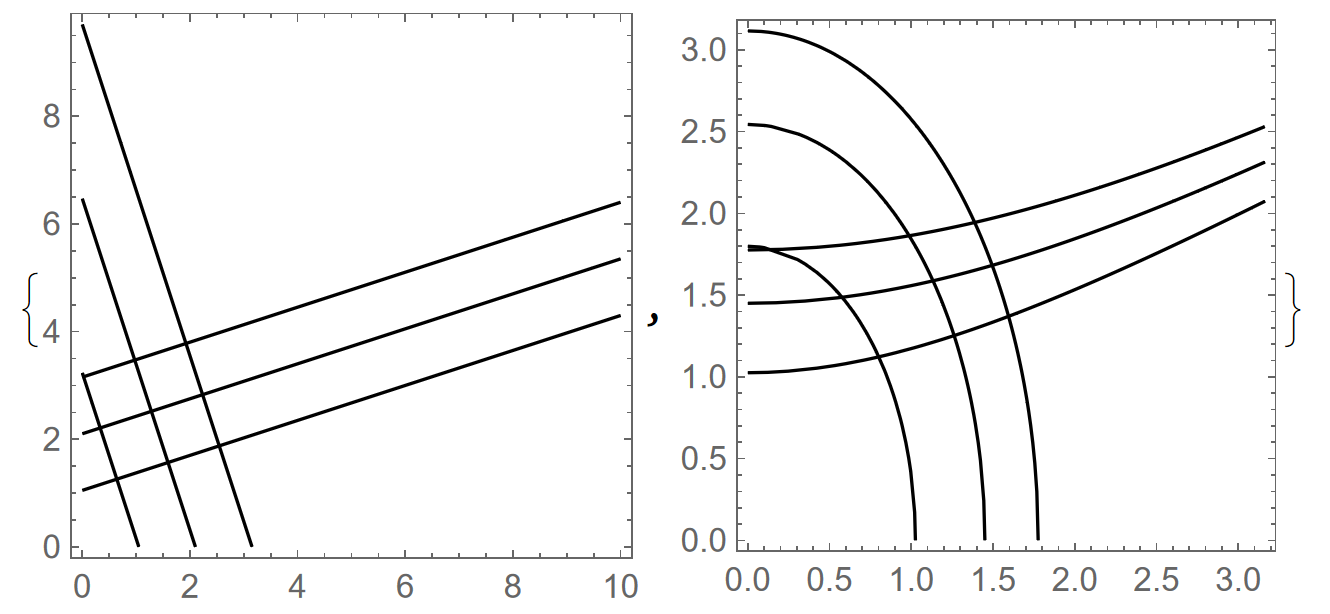
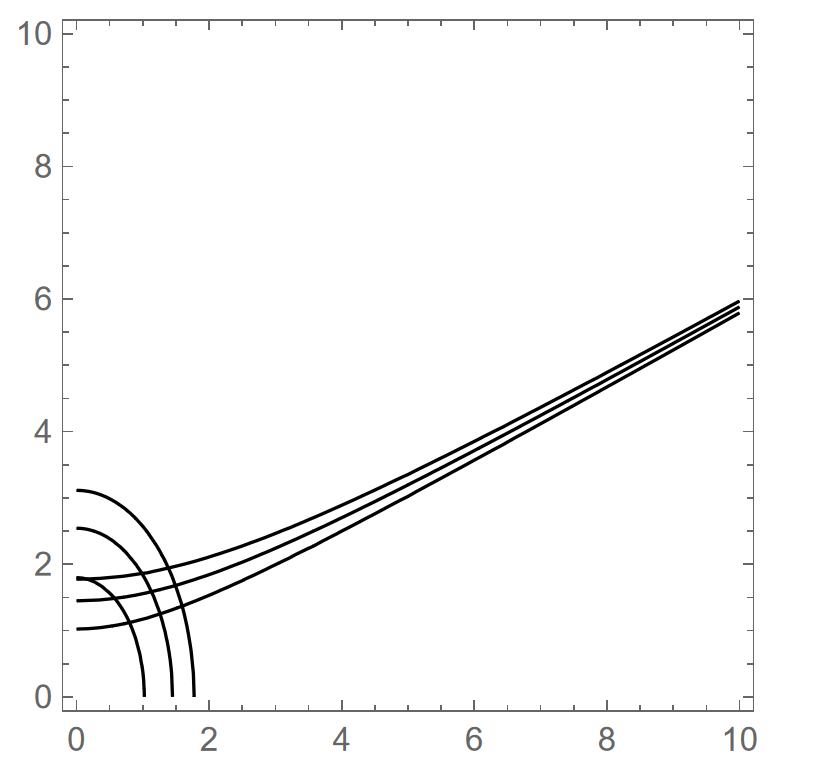
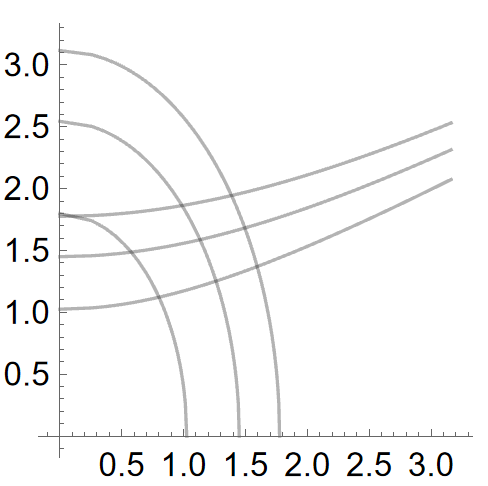
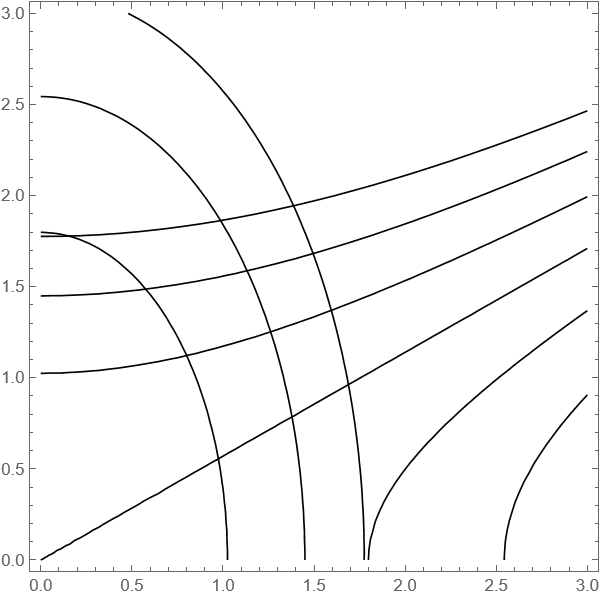
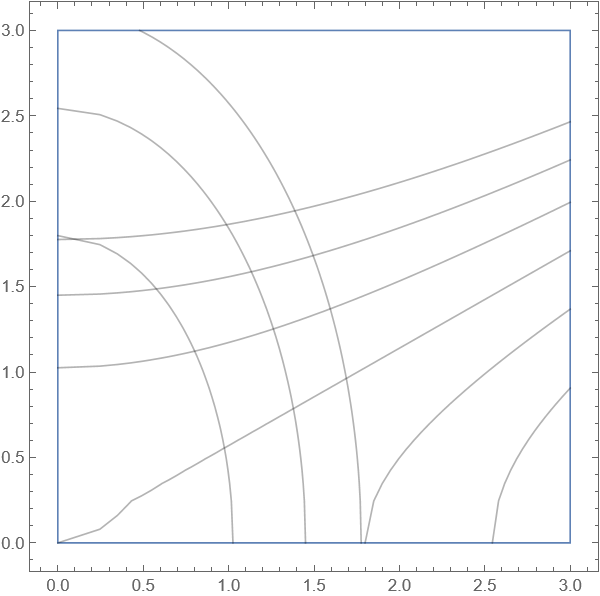
Let $$\eta=x \cos a+y \sin a,\quad\zeta=-x \sin a+y \cos a,\quad 0<a<\pi/2$$

For fixed $a$, the coordinate lines for $\eta=1,2,3, etc$ are the parallel straight lines going from down-right to up-left in x-y plane. The coordinate lines for $\zeta=-3,-2,-1,0,1,2,3, etc$ are the parallel straight lines going from down-left to up-right in x-y plne.

Supposed that I am only interested in those lines in the first quadrant of x-y plane: $(x>0,y>0)$.

Let $(x_1=\sqrt{x},x_2=\sqrt{y}$. How those lines will look like in the first quadrant of the $x_1-x_2$ plane?

Thanks a lot!

Answer 1

Clear["Global`*"];
a = .3*2 π;
η = x*Cos[a] + y*Sin[a];
ζ = -x*Sin[a] + y*Cos[a];
g = Show[
   ContourPlot[η, {x, 0, 10}, {y, 0, 10}, Contours -> {1, 2, 3}, 
    ContourShading -> None], 
   ContourPlot[ζ, {x, 0, 10}, {y, 0, 10}, 
    Contours -> Range[-3, 3], ContourShading -> None], 
   PlotRange -> All, AspectRatio -> Automatic];
{g, g /. {x_Real, y_Real} :> {Sqrt[x], Sqrt[y]}}

• or

a = .3*2 π;
Show[ContourPlot[
  x*Cos[a] + y*Sin[a] /. {x -> x^2, y -> y^2} // Evaluate, {x, 0, 
   10}, {y, 0, 10}, Contours -> {1, 2, 3}, ContourShading -> None], 
 ContourPlot[-x*Sin[a] + y*Cos[a] /. {x -> x^2, y -> y^2} // 
   Evaluate, {x, 0, 10}, {y, 0, 10}, 
  Contours -> {-3, -2, -1, 0, 1, 2, 3}, ContourShading -> None]]

• or

a = .3*2 π;
η = x*Cos[a] + y*Sin[a];
ζ = -x*Sin[a] + y*Cos[a]; 
ParametricPlot[{Sqrt[x], Sqrt[y]}, {x,
   0, 10}, {y, 0, 10}, 
 MeshFunctions -> {Function[{x1, y1, x, y}, η], 
   Function[{x1, y1, x, y}, ζ]}, 
 Mesh -> {{1, 2, 3}, {-3, -2, -1, 0, 1, 2, 3}}, PlotPoints -> 150, 
 MaxRecursion -> 6, PlotStyle -> None, Frame -> False, 
 BoundaryStyle -> None]

Answer 2

This is an extended comment. ContourPlot seems to be best rendering compared with ParametricPlot:

m[a_] := {{Cos[a], Sin[a]}, {-Sin[a], Cos[a]}};
ContourPlot[
 m[0.3 2 Pi] . {u, v} /. {u -> x^2, v -> y^2}, {x, 0, 3}, {y, 0, 3}, 
 Contours -> Range[-3, 3], ContourShading -> None]
ParametricPlot[{Sqrt[x], Sqrt[y]}, {x, 0, 9}, {y, 0, 9}, 
 MeshFunctions -> {Function[{u, v, x, y}, First[m[0.3 
        2 Pi] . {x, y}]], Function[{u, v, x, y}, Last[m[0.3 
        2 Pi] . {x, y}]]}, Mesh -> {Range[-3, 3], Range[-3, 3]}, 
 PlotStyle -> None, PlotPoints -> 150, MaxRecursion -> 6]

Noting:

• coordinate transform is just a rotation
• I am using a used by @cvgmt for comparison (though using more contours/mesh values)