How can I draw the intersection of a plane with a dome tent?

Question

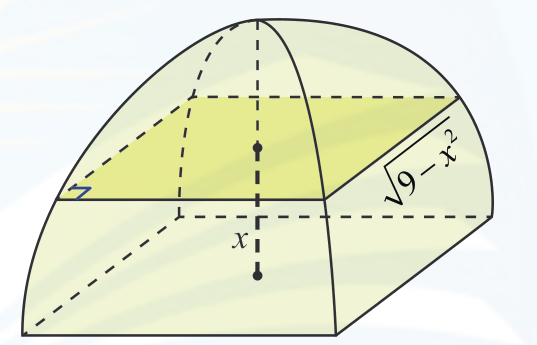
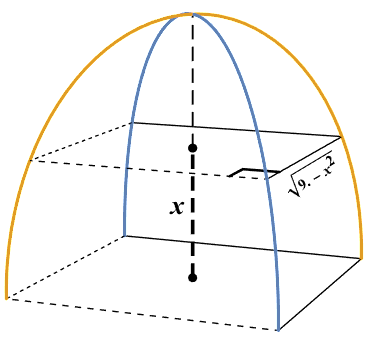
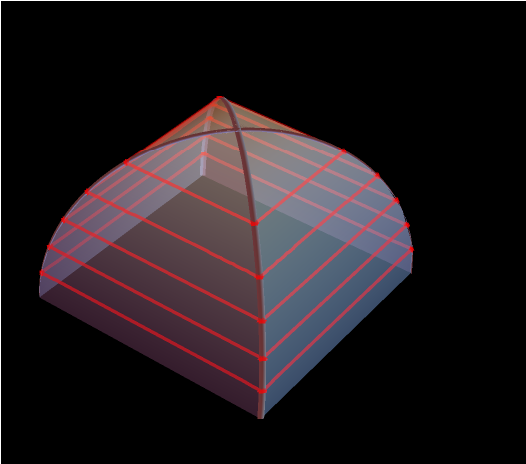
I am trying to draw this picture. I am sorry, I don't know how to start.

Answer 1

Edit

• For k=6 etc.

Clear["Global`*"];
k = 6;
L[θ_] := Sqrt[5^2 - θ^2]/(2   Sin[π/k]);
range = FunctionDomain[L[θ], θ];
pts[θ_] := 
  PadRight[#, 3, θ] & /@ CirclePoints[{L[θ], 0}, k];
lines = ParametricPlot3D[
   pts[x], {x, Mean[{range[[1]], range[[-1]]}], range[[-1]]}, 
   BoxRatios -> Automatic];
surface = 
  ParametricPlot3D[{1 - s, s} . # & /@ Partition[pts[x], 2, 1, 1] // 
    Evaluate, {x, Mean[{range[[1]], range[[-1]]}], range[[-1]]}, {s, 
    0, 1}, Boxed -> False, PlotStyle -> Opacity[.2], Axes -> False, 
   PlotPoints -> 60, MaxRecursion -> 0, Mesh -> None];
Show[lines, surface, 
 Graphics3D[{FaceForm[Directive@{Opacity[.5], Yellow}], 
   Polygon[pts[1.5]], FaceForm[], EdgeForm[Directive@{Orange, Thick}],
    Polygon[pts[0]]}], Boxed -> False, Axes -> False, 
 PlotRange -> All]

Edit

• The length of the square is Sqrt[3^2 - x^2] means that it's 4 points are squares[x] as below.(the height x is the parameter).

• For every 0<=x<=3, we join the 4 points squares[x] to build the surface.

Clear["Global`*"];
squares[x_] := {{-(Sqrt[3^2 - x^2]/2), -(Sqrt[3^2 - x^2]/2), 
    x}, {Sqrt[3^2 - x^2]/2, -(Sqrt[3^2 - x^2]/2), 
    x}, {(Sqrt[3^2 - x^2]/2), Sqrt[3^2 - x^2]/2, 
    x}, {-Sqrt[3^2 - x^2]/2, Sqrt[3^2 - x^2]/2, x}};
lines = ParametricPlot3D[squares[x], {x, 0, 3}, 
   BoxRatios -> Automatic];
surface = 
  ParametricPlot3D[{1 - s, s} . # & /@ 
    Partition[squares[x], 2, 1, 1], {x, 0, 3}, {s, 0, 1}, 
   Boxed -> False, Mesh -> None, PlotStyle -> Opacity[.2], 
   Axes -> False, PlotPoints -> 60, MaxRecursion -> 0];
Show[lines, surface, 
 Graphics3D[{FaceForm[Directive@{Opacity[.5], Yellow}], 
   Polygon[squares[1.5]], FaceForm[], 
   EdgeForm[Directive@{Orange, Thick}], Polygon[squares[0]]}], 
 Boxed -> False, Axes -> False]

• To calculate the volume,one way is using Integrate to accumulate the square area which length is Sqrt[3^2 - x^2].

Integrate[(Sqrt[3^2 - x^2])^2, {x, 0, 3}]

18

or build the solid by BoundaryMeshRegion(compare with https://mathematica.stackexchange.com/a/243985/72111)

k = 4; 
ptss = 
 Table[TranslationTransform[{0, 0, θ}]@*
    ScalingTransform[
     Sqrt[3^2 - θ^2]/(2 Sin[π/k])*{1, 1, 1}]@*
    RotationTransform[0, {0, 0, 1}] /@ (PadRight[#, 3] & /@ 
     CirclePoints[k]), {θ, Subdivide[3, 0, 10^3]}];
{m, n, p} = Dimensions[Rest@ptss];
bm = BoundaryMeshRegion[
  Join[Flatten[Rest@ptss, 1], 
   Union[First@ptss]], {Table[
    Polygon[{{#1[[1]], #2[[1]], #2[[2]]}, {#1[[2]], #1[[1]], \
#2[[2]]}}] & @@@ 
     Thread@{Partition[Range[1, n] + j*n, 2, 1, 1], 
       Partition[Range[1, n] + (j + 1)*n, 2, 1, 1]}, {j, 0, m - 2}], 
   Polygon[Range[1, n] + (m - 1)*n], 
   Polygon /@ (Append[#, n + (m - 1)*n + 1] & /@ 
      Partition[Range[1, n], 2, 1, 1])}]
bm//Volume

18.

Answer 2

A 1-1 copy:

dim = 1.5; \[Alpha] = 45 Degree; h = dim;

SetOptions[Graphics3D, 
 PlotRange -> {{-dim, dim}, {-dim, dim}, {-0.1, 2 dim}},
 Boxed -> False, BoxRatios -> {1, 1, 1}, ViewPoint -> {-5, -2, 1}, 
 ImageSize -> 220]; 

The graphics is a set of primitives, the data part of two ParametricPlot3D curves and a 2d-Epilog for directed Text. The elevated square is generated by a vertical lift mapped into the list of points on the floor.

Graphics3D[{
 {Line[dim {{-1, -1, 0}, {1, -1, 0}, {1, 1, 0}}]}, 
{Dashed, Line[dim {{1, 1, 0}, {-1, 1, 0}, {-1, -1, 0}}]},
{ParametricPlot3D[{{dim Sqrt[2] Cos[\[Phi]] Cos[\[Alpha]], 
   dim Sqrt[2] Cos[\[Phi]] Sin[\[Alpha]] , 
   3 Sin[\[Phi]]}, {dim Sqrt[2] Cos[\[Phi]] Cos[-\[Alpha]], 
   dim Sqrt[2] Cos[\[Phi]] Sin[-\[Alpha]] , 
   3 Sin[\[Phi]]}}, 
       {\[Phi],   0, \[Pi]}][[1]]}, 
 {Line[(# + {0, 0, h} &) /@ 
      (1/2 Sqrt[(2 dim)^2 -  dim^2] {{-1, -1, 0}, {1, -1, 0}, {1, 1, 0}} )]},
 {Dashed, Line[(# + {0, 0, h} &) /@ 
     (1/ 2 Sqrt[(2 dim)^2 -    dim^2] {{1, 1, 0}, {-1, 1, 0}, {-1, -1, 0}}) ]},
  {Thickness[0.007], Line[(# + {0, 0, h} &) /@ 
   (1/ 2 Sqrt[(2 dim)^2 -  dim^2] {{-0.7, -1, 0}, {-0.7, -0.7, 0}, {-1, -0.7, 0}}
      )]},
  {Thickness[0.01], Dashing[{0.04, 0.02}], Line[{{0, 0, 0}, {0, 0, h}}],
   Thickness[0.005],  Dashing[{0.04, 0.02}], Line[{{0, 0, h}, {0, 0, 3}}]},
 {Black, Ball[{0, 0, 0}, 0.05], Ball[{0, 0, h}, 0.05]},
  {Style[{Text[x, {0.3, 0.3, 0.75}] }, Bold, 16,  FontFamily -> "Times"]}}, 
  Epilog -> {Style[ Text[Sqrt[(2 dim)^2 - x^2], Scaled[{0.85, 0.48}], 
 Automatic, {1, 0.6}], Bold, 9, FontFamily -> "Times"]}]

The dashing line parts could be aligned with the ViewPoint

Answer 3

An approach:

f[s_, t_] := 
 s {0, 3 Cos[t], 3 Sin[t]} + (1 - s) {3 Cos[t], 0, 3 Sin[t]}
r = RotationMatrix[Pi/2, {0, 0, 1}]

ParametricPlot3D[{f[0, t], f[1, t], f[s, t], r . f[s, t]}, {t, 0, 
  Pi}, {s, 0, 1}, Mesh -> 5, MeshFunctions -> (#3 &), 
 MeshStyle -> {Red, Thick}, 
 PlotStyle -> {Directive[White, Thickness[0.1], Opacity[1]], 
   Directive[White, Thickness[0.1], Opacity[1]], LightBlue, 
   LightBlue}, Background -> Black, Boxed -> False, Axes -> False, 
 BaseStyle -> Opacity[0.5]]