I want to find the constant in front of the term of the integral that only contains $x$ in the following function: $$f(x) = \frac{(56-15 \sin x + \sin^2 x)^2}{6-5 \sin x + \sin^2 x}$$
This is my input in Mathematica:
Integrate[((56 - 15 Sin[x] + Sin[x]^2*x)^2)/(6 - 5 Sin[x] + Sin[x]^2), x]
And this is the output:
1/2 x (450 - 38 x + 13 x^2) - 5 (-2 - 6 x + x^2) Cos[x] -
1/4 x Cos[2 x] - (1/Sqrt[2])
I (1/2 (11 + 9 x)^2 Log[1 + I (3 - 2 Sqrt[2]) E^(I x)] -
1/2 (11 + 9 x)^2 Log[1 + I (3 + 2 Sqrt[2]) E^(I x)] -
9 I (11 + 9 x) PolyLog[2, I (-3 + 2 Sqrt[2]) E^(I x)] +
9 I (11 + 9 x) PolyLog[2, -I (3 + 2 Sqrt[2]) E^(I x)] +
81 PolyLog[3, I (-3 + 2 Sqrt[2]) E^(I x)] -
81 PolyLog[3, -I (3 + 2 Sqrt[2]) E^(I x)]) - (1/Sqrt[3])
4 I (-(13 + 2 x)^2 Log[(2 + Sqrt[3] + I E^(I x))/(
2 + Sqrt[3])] + (13 + 2 x)^2 Log[
1 - (I E^(I x))/(-2 + Sqrt[3])] -
4 I (13 + 2 x) PolyLog[2, (I E^(I x))/(-2 + Sqrt[3])] +
4 I (13 + 2 x) PolyLog[2, -((I E^(I x))/(2 + Sqrt[3]))] +
8 PolyLog[3, (I E^(I x))/(-2 + Sqrt[3])] -
8 PolyLog[3, -((I E^(I x))/(2 + Sqrt[3]))]) +
10 (-3 + x) Sin[x] - 1/8 (-1 + 2 x^2) Sin[2 x]
Therefore, the constant before the $x$-term is $450/2=225$, however that is incorrect. What am I doing wrong?

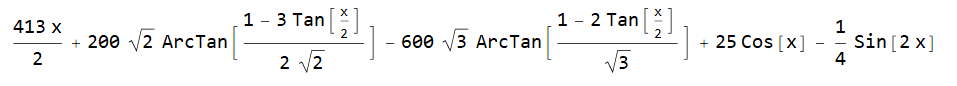
xmultiplyingSin[x]^2. $\endgroup$Coefficient[...,x]? $\endgroup$