I use Acegen for Finitie Element formulation that gives out residuals and tangents by taking in the field values as inputs. For this in my residual formulation I need to integrate a nonlinear term over the element Area(2D Element). For some reason, this doesn't give a result. I isolated the problem in a smaller version of the code as shown below:
SetOptions[EvaluationNotebook[]]
ClearAll["Global`*"]
<< AceGen`; NAME = "QIntegrate";
SMSInitialize[NAME, "Language" -> "Matlab", "Mode" -> "Debug"];
(*Initialization*)
SMSModule[NAME, Real[ y$$[2], ue$$[4], a$$, b$$, f$$, intf$$],
"Input" -> {y$$, ue$$, a$$},
"Output" -> {f$$, intf$$}];
(*copy Acegen variables to Mathematica variables*)
{y1,
y2} \[RightTee] SMSReal[Table[y$$[i], {i, 2}]];
ue \[DoubleRightTee] SMSReal[Table[ue$$[i], {i, 4}]];
ue1 \[DoubleRightTee] SMSReal[ue$$[1]];
ue2 \[DoubleRightTee] SMSReal[ue$$[2]];
ue3 \[DoubleRightTee] SMSReal[ue$$[3]];
ue4 \[DoubleRightTee] SMSReal[ue$$[4]];
a \[DoubleRightTee] SMSReal[a$$];
(*Shape Functions*)
sf \[DoubleRightTee] ConstantArray[1, 4];
sf[[1]] \[DoubleRightTee] 0.25*(1 - y1)*(1 - y2);
sf[[2]] \[DoubleRightTee] 0.25*(1 + y1)*(1 - y2);
sf[[3]] \[DoubleRightTee] 0.25*(1 + y1)*(1 + y2);
sf[[4]] \[DoubleRightTee] 0.25*(1 - y1)*(1 + y2);
(*Field value interpolated at gauss point*)
uGP \[DoubleRightTee] sf . ue;
(*Non-linear function definition*)
f \[DoubleRightTee] Exp[uGP];
(*Integrate the function over the area of the element after restoring y1 and y2 dependencies*)
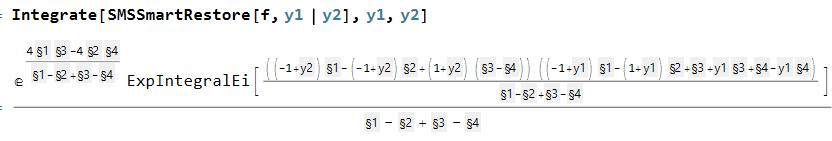
intf = Integrate[
SMSSmartRestore[f, y1 | y2], {y1, -1, 1}, {y2, -1, 1}];
(*export the output variables/copy mathematica variables to AceGen \
variables*)
SMSExport[f, f$$];
SMSExport[intf, intf$$];
SMSWrite[NAME, "LocalAuxiliaryVariables" -> True];
FilePrint[StringJoin[NAME, ".m"]]
This should technically run since the function is integrable. But the code doesnt give any result. Just says:
Expression contains part/parts that can not be numerically evaluated.
User subroutine: QIntegrate
Error in user input parameters for function: SMSExport
Input parameter: {LARGE EXPRESSION}
Parts that can not be evaluated: {Undefined}
Events: 0
Version: 7.505 Linux (16 Aug 22) (MMA 13.) Module: SMSExport
See also: Symbolic-Numeric Interface AceGen Troubleshooting Continue
I tried many things such as: defining the exponent as a polynomial function instead of a vector multiplication
uGP \[DoubleRightTee]
0.25*(1 - y1)*(1 - y2)*ue1 + 0.25*(1 + y1)*(1 - y2)*ue2 +
0.25*(1 + y1)*(1 + y2)*ue3 + 0.25*(1 - y1)*(1 + y2)*ue4;
using SMSPower instead
f \[DoubleRightTee] SMSPower[E, uGP];
It only gives a result for:
intf = Integrate[f, {y1, -1, 1}, {y2, -1, 1}];
but here it considers the exponent as independent of y1 and y2 and gives out wrong result.
or
f \[DoubleRightTee] Exp[a];
where a is an independent variable
but surprisingly f=sf works with array output
f \[DoubleRightTee] Exp[sf];
I'm not able to understand the issue. Please suggest a resolution to this. I'm not sure where the problem is occuring and my entire work depends on this. Any help is extremely appreciated. Thank You!!