There are two equations:
eq1 = x1^2/a^2 + y1^2/b^2 == 1;
eq2 = x2^2/a^2 + y2^2/b^2 == 1;
How to get this result by subtracting two equations?
the result
((x1 - x2) (x1 + x2))/a^2 + ((y1 - y2) (y1 + y2))/b^2 == 0
subtracting two equations?
You can use SubtractSides which is designed for this sort of operation.
ClearAll["Global`*"]
eq1 = x1^2/a^2 + y1^2/b^2 == 1
eq2 = x2^2/a^2 + y2^2/b^2 == 1
SubtractSides[eq1, eq2]
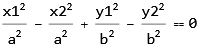
To simplify it more
SubtractSides[eq1, eq2] // Simplify
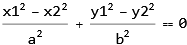
Without using this command, there are other ways to do this. See Subtracting equations from each other? for more options.
(eq1 - eq2 /. Equal -> List // Equal @@ # & //
Collect[#, {a, b}] &) /. (a_^2 - b_^2) :> (a + b) (a - b)
$$\frac{(\text{x1}-\text{x2}) (\text{x1}+\text{x2})}{a^2}+\frac{(\text{y1}-\text{y2}) (\text{y1}+\text{y2})}{b^2}$$
Try also this:
eq1 = x1^2/a^2 + y1^2/b^2 == 1;
eq2 = x2^2/a^2 + y2^2/b^2 == 1;
expr=Equal @@ MapThread[Subtract, {List @@ eq1, List @@ eq2}] // Simplify
(* (x1^2 - x2^2)/a^2 + (y1^2 - y2^2)/b^2 == 0 *)
If you need them factorized:
MapAt[Factor, expr, {{1, 1}, {1, 2}}]
(* ((x1 - x2) (x1 + x2))/a^2 + ((y1 - y2) (y1 + y2))/b^2 == 0 *)
Have fun!
Another way to do this is as follows:
FullSimplify[Thread[#[[1]] == #[[2]]] &@(#[[1]] - #[[2]] & /@
Transpose[Level[#, {1}] & /@ {eq1, eq2}])]
eq1 = x1^2/a^2 + y1^2/b^2 == 1;
eq2 = x2^2/a^2 + y2^2/b^2 == 1;
Original way
eq1 - eq2 // Thread[#, Equals] & // Simplify
(* (x1^2-x2^2)/a^2+(y1^2-y2^2)/b^2==0 *)
If you use EqualThread found at https://library.wolfram.com/infocenter/MathSource/4491, then it will be seamless.
eq1 - eq2//Simplify
to get the same answer.