Numerical Plot for an example

Question

I would like to compare the numerical and analytical solution with plot for this example but something is wrong I don't know how to fix it?!!

      Clear["Global`*"] 
      eqn = {2 s'[x] + 3 s[x]^2 - 3 f == 0};
      sol = NDSolveValue[{eqn /. {f -> 0.7}, s[0] == 1}, s[x], {x, 0, 8}];
      g[x_] := 1 - f/s[x]^2;
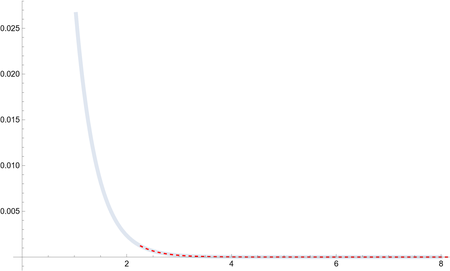
      pp1 = Plot[Evaluate[{g[x] /. {f -> 0.7}}], {x, 0, 8}]

     sol1 = DSolve[{2 w'[t] + 3 w[t]^2 - 3 p == 0, w[0] == 1}, w[t], t];
     p = 0.7;
     g1[t_] := 1 - p/w[t]^2;
     pp2 = Plot[g1[t] /. sol1, {t, 0, 15}, PlotStyle -> Dashed]
     Show[pp1, pp2]

Answer 1

When we use NDSolveValue, the result sol is the interpolation function. It is difference to NDSolve。

eqn = {2 s'[x] + 3 s[x]^2 - 3 f == 0};
sol = NDSolveValue[{eqn /. {f -> 0.7}, s[0] == 1}, s[x], {x, 0, 8}];
g[x_] = 1 - f/sol^2;
pp1 = Plot[Evaluate[{g[x] /. {f -> 0.7}}], {x, 0, 8}, 
   PlotStyle -> {Opacity[.2], AbsoluteThickness[5]}];
sol1 = DSolve[{2 w'[t] + 3 w[t]^2 - 3 p == 0, w[0] == 1}, w[t], t];
p = 0.7;
g1[t_] = 1 - p/w[t]^2;
pp2 = Plot[g1[t] /. sol1, {t, 0, 15}, PlotStyle -> {Dashed,Red}];
Show[pp1, pp2]