Borrowed Stolen Adapted from @J.M., Extruding along a path, to add end caps to make a closed polyhedron for 3D printing:
(*Pixar method;http://jcgt.org/published/0006/01/01/*)
orthogonalDirections[{p1_?VectorQ, p2_?VectorQ}] :=
Module[{s, w, w1, xx, yy, zz}, {xx, yy, zz} = Normalize[p2 - p1];
s = 2 UnitStep[zz] - 1; w = -1/(s + zz); w1 = xx yy w;
{{1 + s w xx^2, s w1, -s xx}, {w1, s + w yy^2, -yy}}]
orthogonalDirections[{p1_?VectorQ, p2_?VectorQ, p3_?VectorQ}] :=
Module[{d, u, v}, {u, v} = Normalize /@ {p3 - p2, p1 - p2};
If[Chop[Norm[u - v] Norm[u + v]] != 0, d = (u + v)/2;
Normalize /@ {d, Cross[u, d]}, orthogonalDirections[{p1, p2}]]]
extend[cs_, q_, d_, nrms_] :=
cs + Outer[Times,
First[
LinearSolve[Transpose[Prepend[-nrms, d]], q - Transpose[cs]]], d]
(*for custom cross-sections*)
crossSection[pointList_?MatrixQ, r_, csList_?MatrixQ] :=
Module[{p1, p2}, {p1, p2} = Take[pointList, 2];
(p1 + #) & /@ (r csList . orthogonalDirections[{p1, p2}])] /;
Last[Dimensions[pointList]] == 3 && Last[Dimensions[csList]] == 2
(*for circular cross-sections*)
crossSection[pointList_?MatrixQ, r_, n_Integer] :=
crossSection[pointList, r,
Composition[Through, {Cos, Sin}] /@ Range[0, 2 Pi, 2 Pi/n]]
(*approximate vertex normals,for a smooth appearance*)
vertNormals[vl_ /; ArrayQ[vl, 3, NumericQ]] :=
Block[{mdu, mdv, msh},
msh = ArrayPad[#, {{1, 1}, {1, 1}}, "Extrapolated",
InterpolationOrder -> 2] & /@ Transpose[vl, {2, 3, 1}];
mdu = ListCorrelate[{{1, 0, -1}}/2, #, {{-2, 1}, {2, -1}}, 0] & /@
msh;
mdv = ListCorrelate[{{-1}, {0}, {1}}/2, #, {{1, -2}, {-1, 2}},
0] & /@ msh;
MapThread[Composition[Normalize, Cross],
Transpose[{mdu, mdv}, {1, 4, 2, 3}], 2]]
MakePolygons // ClearAll;
MakePolygons[vl_ /; ArrayQ[vl, 3, NumericQ],
OptionsPattern[{"Normals" -> True, "Closed" -> False}]] :=
Module[{dims = Most[Dimensions[vl]]}, GraphicsComplex[Apply[Join, vl],
{If[TrueQ@OptionValue@"Closed",
Polygon[{Range@Length@First@vl,
Range[1 + Length@First@vl (Length@vl - 1),
Length@First@vl*Length@vl]}, VertexNormals -> None],
{}],
Polygon[
Flatten[
Apply[Join[Reverse[#1], #2] &,
Partition[
Partition[Range[Times @@ dims], Last[dims]], {2, 2}, {1,
1}], {2}], 1]]},
If[TrueQ[OptionValue["Normals"] /. Automatic -> True],
VertexNormals -> Apply[Join, vertNormals[vl]], Unevaluated[]]]]
TubePolygons // ClearAll;
TubePolygons // Options = {"Normals" -> True, "Scale" -> 1.,
"Closed" -> False};
TubePolygons[path_?MatrixQ, cs : (_Integer | _?MatrixQ),
OptionsPattern[]] :=
With[{p3 = PadRight[path, {Automatic, 3}]},
MakePolygons[
FoldList[
Function[{p, t},
extend[p, t[[2]], t[[2]] - t[[1]], orthogonalDirections[t]]],
crossSection[p3, OptionValue["Scale"], cs],
Partition[p3, 3, 1, {1, 2}, {}]],
"Normals" -> OptionValue["Normals"],
"Closed" -> OptionValue["Closed"]]]
OP's example:
path = First@
Cases[
ParametricPlot3D[{Cos[ωt], Sin[ωt], 0}, {ωt,
0, π/2}, ImageSize -> 500, SphericalRegion -> True,
PlotRange -> All], Line[l_] :> l, Infinity];
Graphics3D[{EdgeForm[],
TubePolygons[path, 30, "Normals" -> True, "Scale" -> 0.2,
"Closed" -> True]}, Axes -> True]
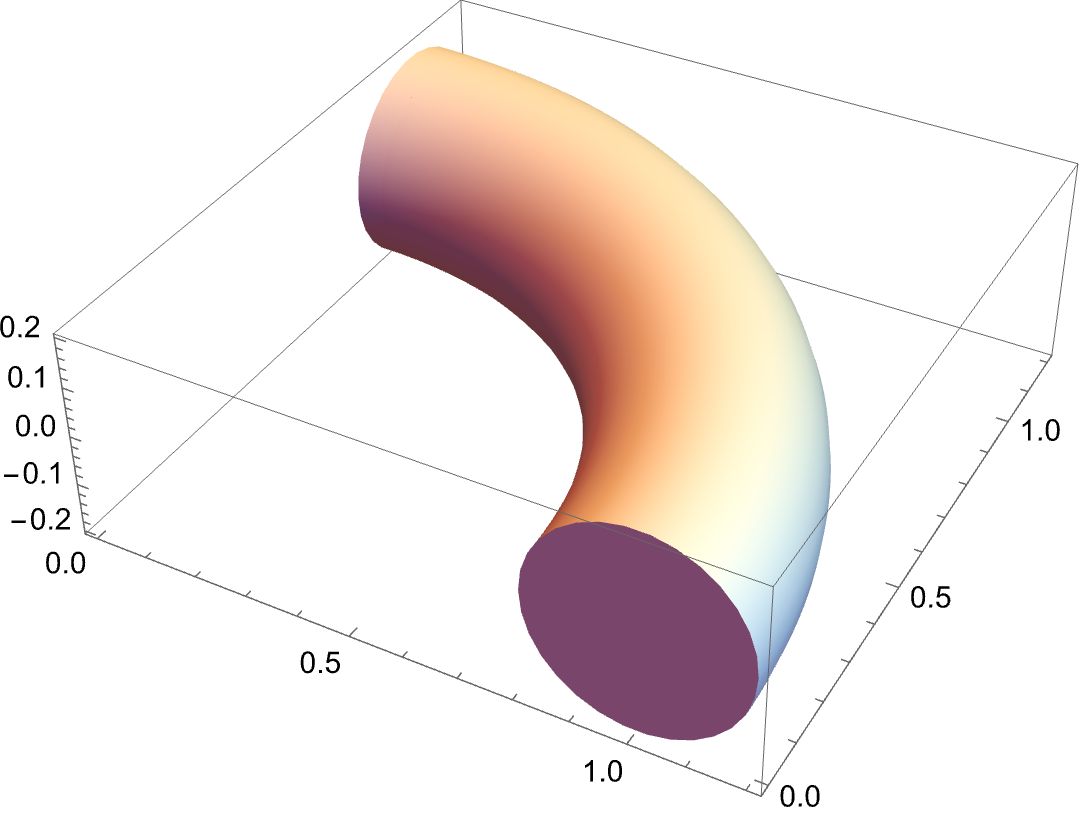


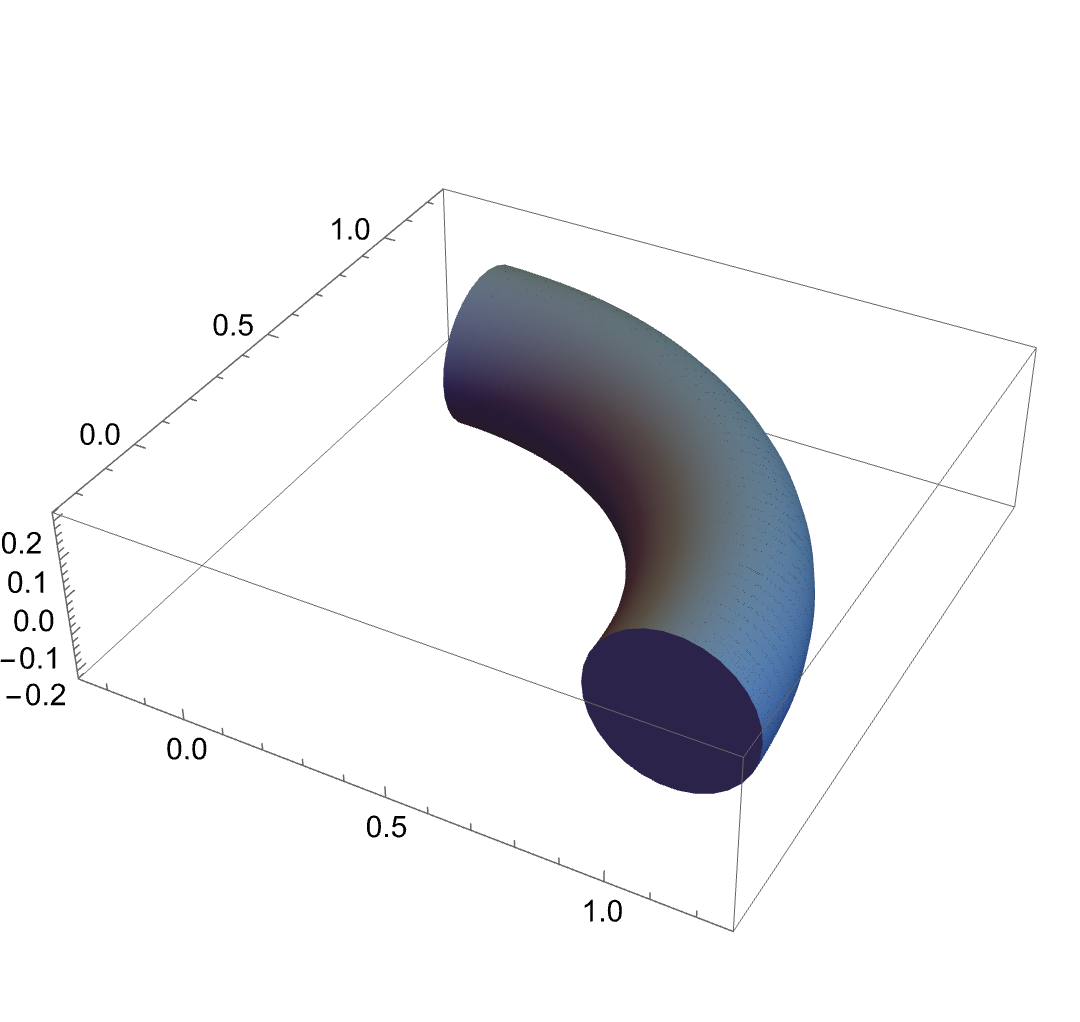
ParametricPlot3D[{Cos[\[Omega]t], Sin[\[Omega]t], 0} , {\[Omega]t, 0, \[Pi]/2} , ImageSize -> 500 , SphericalRegion -> True , PlotRange -> All , PlotStyle -> Directive[CapForm["Square"]] ] /. Line -> (Tube[#, 0.2] &)$\endgroup$DiscretizeGraphics[p1, MaxCellMeasure -> {"Area" -> 0.002}]$\endgroup$Mesh->20in the plot or experiment to find if it improves anything. Good luck. $\endgroup$