First of all, let us mention two syntax errors in your code.
First, the underscore has a special meaning in Mma. Therefore, notations like C_1 are illegal. Use C1 instead.
Second, the use of Solve requires that the Equal, rather than Set stays in the expression you want to be interpreted as an equation. Therefore, use == instead of =.
After fixing this, the answer to your question is not very difficult. I will do it starting with your original idea.
Here is your equation and its solution:
sol = Solve[ds^2 == -(C1 + A*t)^2 dt^2 + C2^2 dr^2, dr][[2, 1]]
(* dr -> Sqrt[ds^2 + C1^2 dt^2 + 2 A C1 dt^2 t + A^2 dt^2 t^2]/C2 *)
By default, Mma returns the solution in the form of a rule. Let us transform it into an equation:
eq1 = Equal @@ sol
(* dr == Sqrt[ds^2 + C1^2 dt^2 + 2 A C1 dt^2 t + A^2 dt^2 t^2]/C2 *)
Let us now divide the both parts of this equation by dt:
eq2 = Assuming[{dt != 0}, DivideSides[eq1, dt]]
(* dr/dt == Sqrt[ds^2 + C1^2 dt^2 + 2 A C1 dt^2 t + A^2 dt^2 t^2]/(C2 dt) *)
Now, let us bring dt under the square root:
eq3 = expr1 /. Sqrt[a_]/dt :> Sqrt[a/dt^2]
(* dr/dt == Sqrt[(
ds^2 + C1^2 dt^2 + 2 A C1 dt^2 t + A^2 dt^2 t^2)/dt^2]/C2 *)
and let us, further, expand the expression under the radical:
MapAt[Expand, expr2, {2, 2, 1}]
(* dr/dt == Sqrt[C1^2 + ds^2/dt^2 + 2 A C1 t + A^2 t^2]/C2 *)
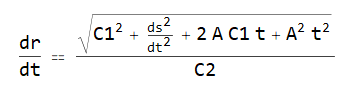
Done. Have fun!

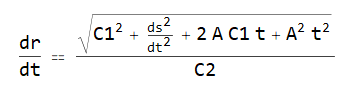
Solvefunction, not relativity or differentials. $\endgroup$Reduce[{q == dr/dt, ds^2 == -(C[1] + A*t)^2 dt^2 + C[2]^2 dr^2}, {q}, {dr, dt}]. However it does not directly reproduce your result. Are you sure of it? Could ds be zero? $\endgroup$