Ordered partition in a summation

Question

I need to ensure that the order is important and that $a_1a_2a_2$ is different from $a_2a_1a_2$ or as a matter of fact, any other possibility. In other words, $a_m$s are considered non-commutative. So how do I write this up in Mathematica, as I already figured out that IntegerPartitions is not something I'm looking for? The deal is with Ordered Partitions. $$\sum_{i_1+i_2+...+i_m=n}a_{i_1}a_{i_2}...a_{i_m}\Big\}$$ Thankful for your efforts.

Update

For more context, this question is part of another one:

Double summation involving partition of a number n as a condition

Answer 1

Something like this?

n = 4
times[x_] := x;
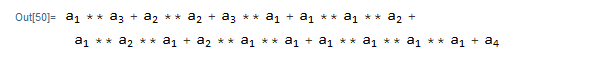
times[args__] := NonCommutativeMultiply[args];
Total[
 times @@@ Map[Subscript[a, #] &,
   Flatten[Permutations /@ IntegerPartitions[n], 1],
   {2}
  ]
]