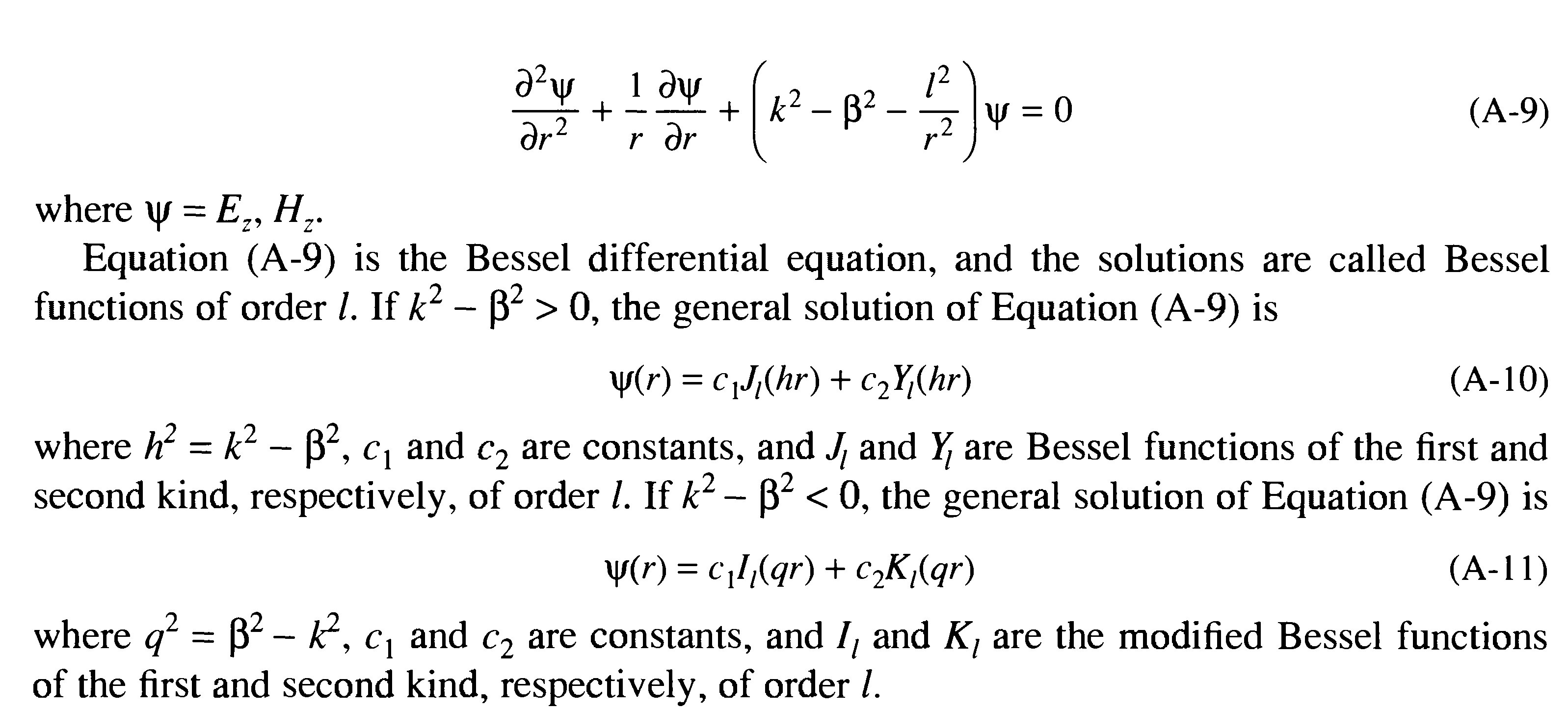I have been working on waveguide mode analysis using FEM in Mathematica for a week, but I haven't succeeded until now.
The optical fiber-like waveguide is featured with different refractive index in core and in clad, and the interface between the core and the clad should have the boundary condition of $D_⊥$ (the normal component of $\mathbf{D}$) and $\mathbf{E}_∥$ (the tangential component of $\mathbf{E}$) are continuous. But I don't know how to express this kind of boundary condition in Mma. I think this is of course different in Neumann, Dirichlet and Robin conditions.
The physical model is described below.
For Helmholtz equation for optical waveguide:
$$\nabla ^2 E(x,y,z)+\epsilon (\frac{2 \pi} {\lambda})^2 E(x, y, z)=0$$
Assuming that $$E(x,y,z)=E(x,y) e^{i \beta z}$$
We have
$$\nabla ^2 E(x,y)+\epsilon \left(\frac{2 \pi }{\lambda }\right)^2- \beta^2 E(x,y) = 0$$
The $\beta$ in this equation is to be solved and $\beta ^2$ can be considered as the eigen value of this eigen equation
$$\nabla ^2 E(x,y)+\epsilon \left(\frac{2 \pi }{\lambda }\right)^2 = \beta^2 E(x,y)$$
$\epsilon$ is different for core and cladding, i.e. , $\epsilon_\text{core}$ and $\epsilon_\text{clad}$, respectively.
The boundary conditions at the interface should be : (1) the tangential component of the $\mathbf{E}$, i.e. $\mathbf{E}_∥$, is continuous. (2) the normal component of the $\mathbf{D}$, i.e. $D_⊥$, is continuous, in which $\mathbf{D}=\epsilon \mathbf{E}$. In the cylindrical coordinates $(r, \theta, z)$, the boundary conditions at interface should be, $E_z$ and $E_\theta$ is continuous, and $D_r$ is continuous.
These conditions are my main concern when using FEM for the analysis of the eigenmode. Although they can be formulated easily in some special cases such as in rectangular or circular waveguide, but I'd like to try a more general form.
Here is my unsuccessful try.( Mma 12.0, Win 10)
To make the mesh points on the boundary, it can be used like this,
<< NDSolve`FEM`
r = 0.8;
outerCirclePoints =
With[{r = 2.},
Table[{r Cos[θ], r Sin[θ]}, {θ, Range[0, 2 π, 0.05 π] // Most}]]; (* the outer circle *)
innerCirclePoints =
With[{r = r},
Table[{r Cos[θ], r Sin[θ]}, {θ, Range[0, 2 π, 0.08 π] // Most}]]; (* the inner circle *)
bmesh = ToBoundaryMesh[
"Coordinates" -> Join[outerCirclePoints, innerCirclePoints],
"BoundaryElements" -> {LineElement[
Riffle[Range[Length@outerCirclePoints],
RotateLeft[Range[Length@outerCirclePoints], 1]] //
Partition[#, 2] &],
LineElement[
Riffle[Range[Length@outerCirclePoints + 1,
Length@Join[outerCirclePoints, innerCirclePoints]],
RotateLeft[
Range[Length@outerCirclePoints + 1,
Length@Join[outerCirclePoints,innerCirclePoints]],1]] //Partition[#,2] &]}];
mesh = ToElementMesh[bmesh];
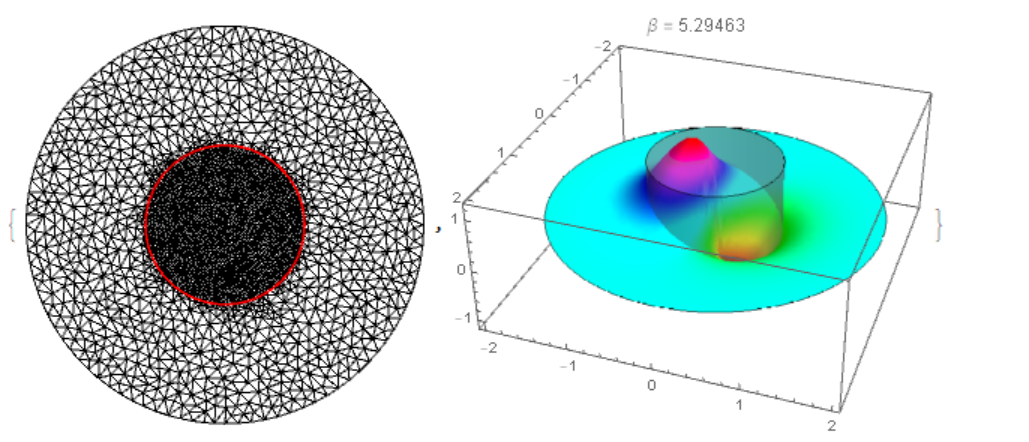
{bmesh["Wireframe"], mesh["Wireframe"]}
(* generate the boundary and element mesh, to make the mesh points \
on the outer and inner circles *)
glass = 1.45^2; air = 1.; k0 = (2 π)/1.55;
ϵ[x_, y_] := If[x^2 + y^2 <= r^2, glass, air]
helm = \!\(\*SubsuperscriptBox[\(∇\), \({x, y}\), \(2\)]\(u[x,y]\)\) + ϵ[x, y]*k0^2*u[x, y];
boundary = DirichletCondition[u[x, y] == 0., True];
(*region=ImplicitRegion[x^2+y^2≤2.^2,{x,y}];*)
{vals, funs} = NDEigensystem[{helm, boundary}, u[x, y], {x, y} ∈ mesh, 1,Method -> {"Eigensystem" -> {"FEAST","Interval" -> {k0^2, glass* k0^2}}}];
vals
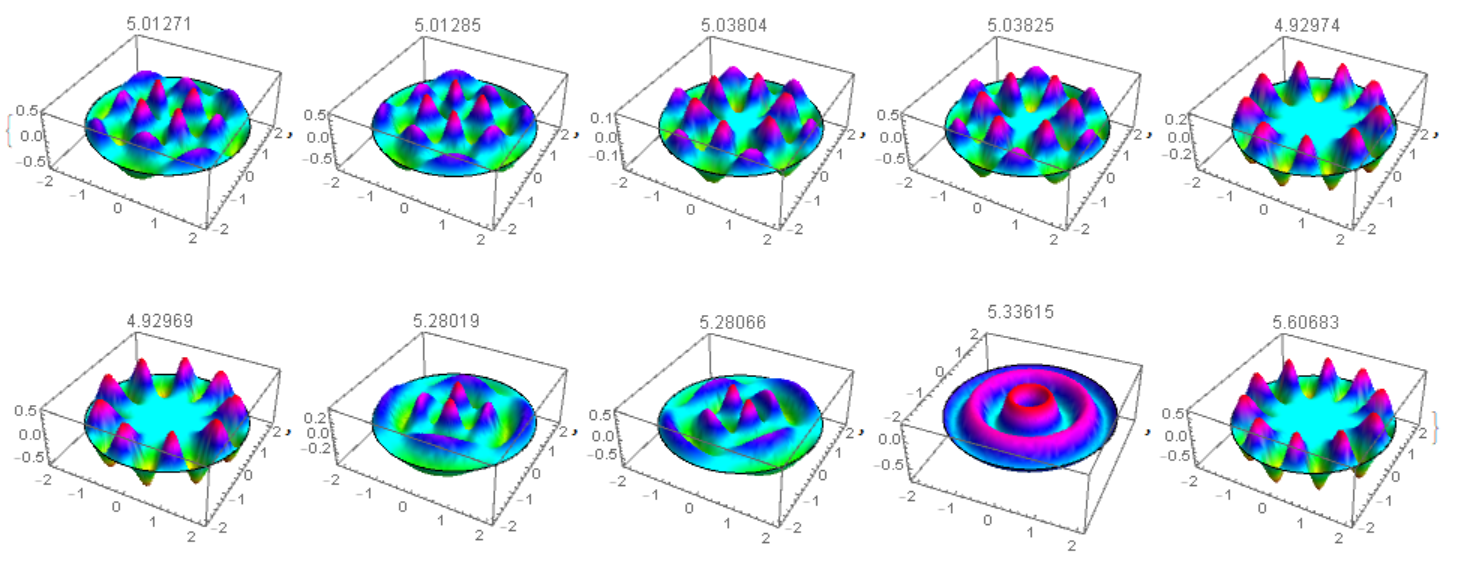
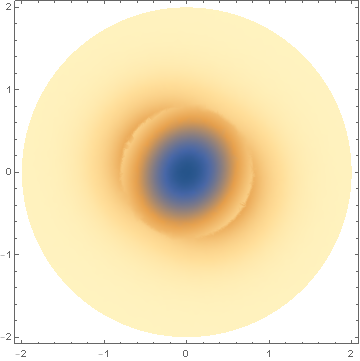
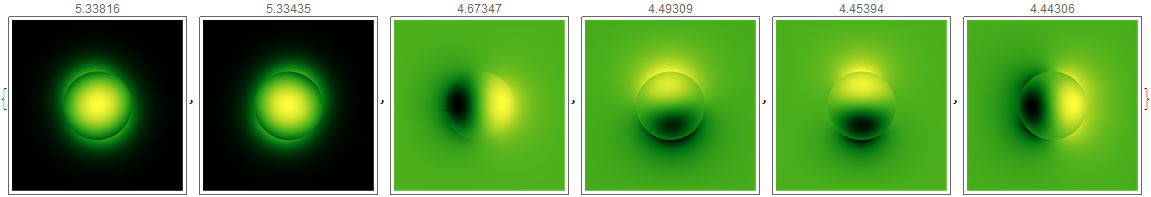
Table[Plot3D[funs[[i]], {x, y} ∈ mesh, PlotRange -> All,
PlotLabel -> vals[[i]]], {i, Length[vals]}]
Although the profile in the figure seems right, but the eigenvalue is not right, since I can check it using analytical solutions.
Edit 1
I notice their is a very closely related post here, where PML is employed. However, there are some bug there, and it couldnot properly run.
Are there some more examples? Thank you in advance.
Edit 2:
For analytical solution, I have to mention that it can be obtained by solving a transdental equation shown in the figure for a circular waveguide. The derivation of this equation can be found in papers, for example, https://www.osapublishing.org/oe/abstract.cfm?uri=oe-12-6-1025.
In addition, I can check the FEM results with other FEM tools like COMSOL.
Edit 3
I'm thankful to user21 for his kindness and patience. In this post, I will first give more information on the analytical solution, then I would present more clearly what I want to do with FEM.
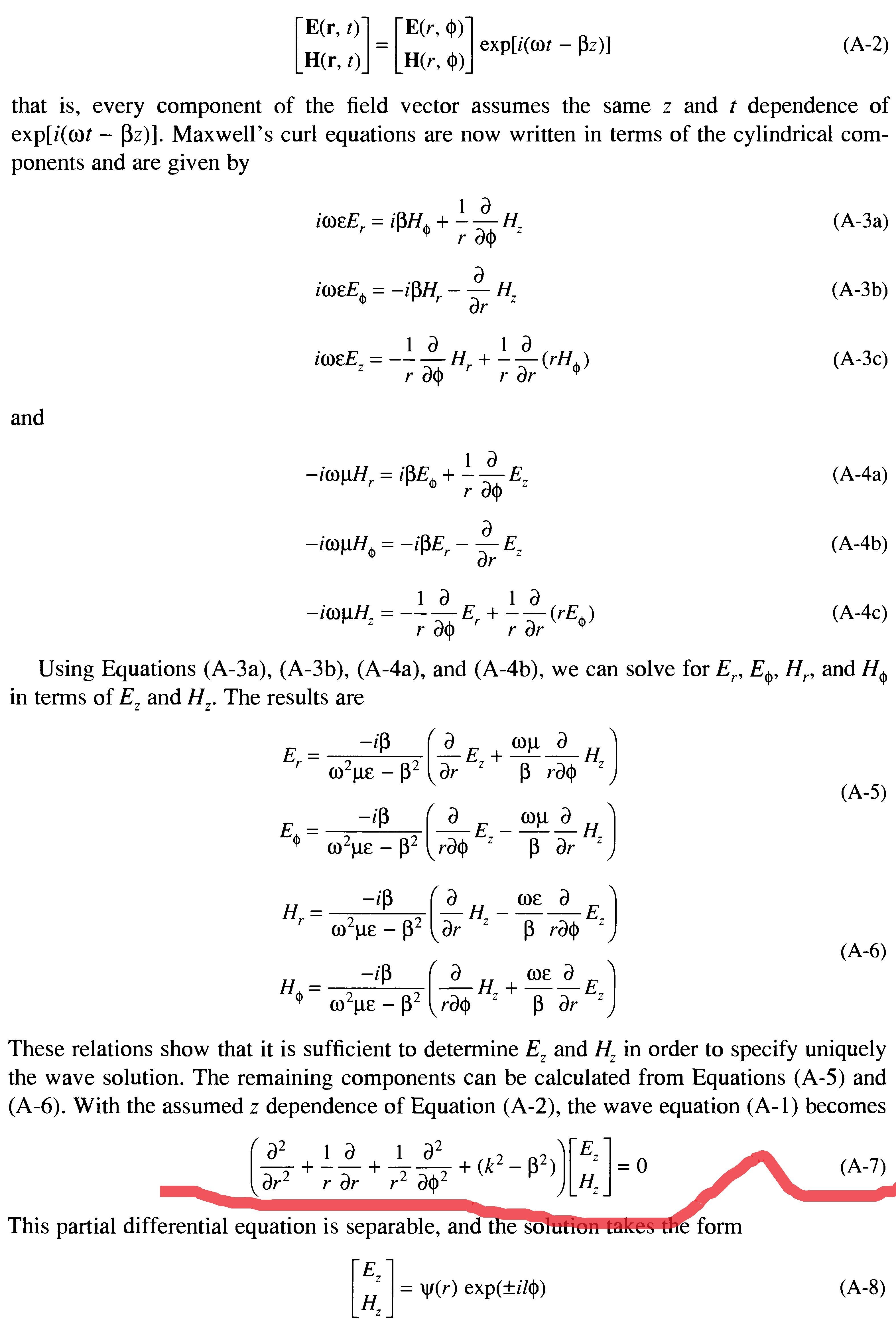
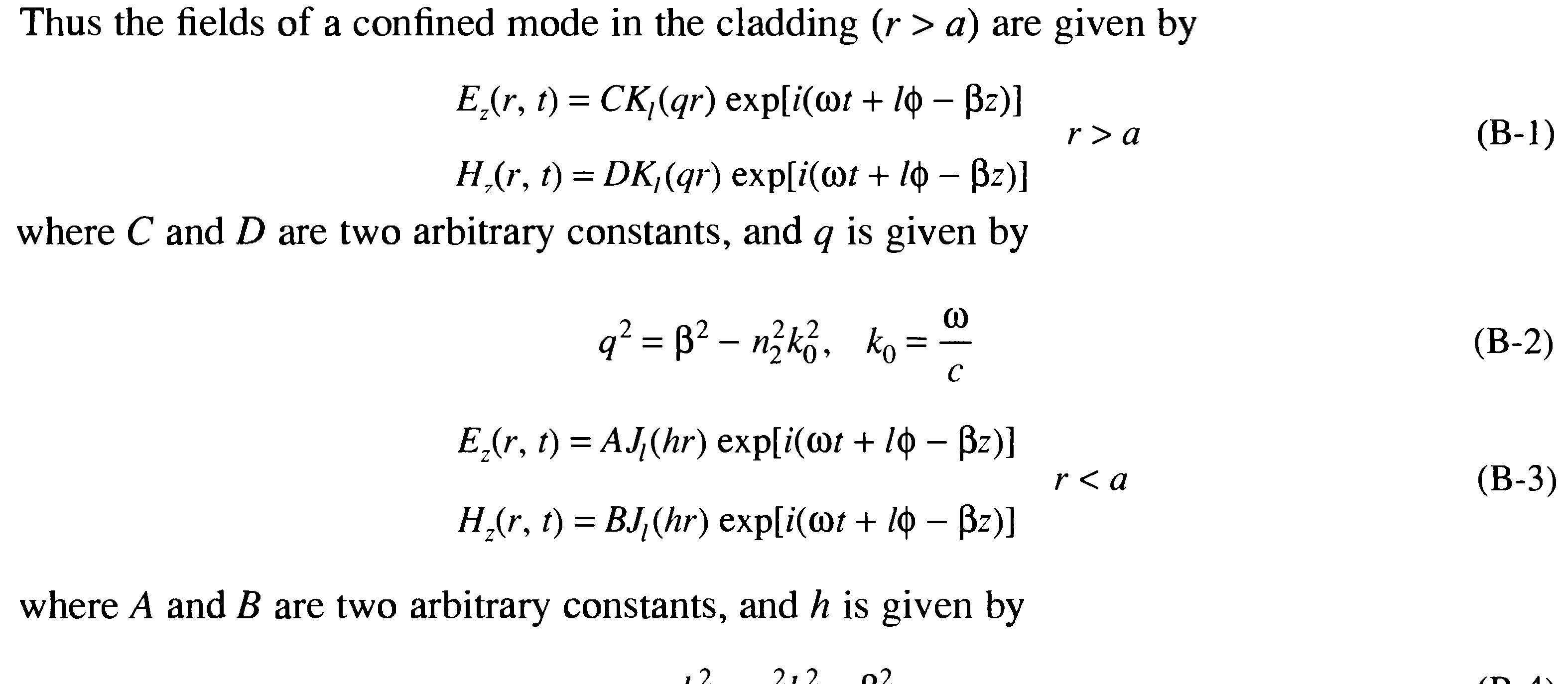
Since there are too many equations, I'd like to post the revelant contents from textbooks (the appendix of “Photonics”, written by A. Yariv) about the analytical approch to the eigenmodes of optical fibers. I have underlined the most important equations in the pictures to make these stuffs to be understand more easily.
Therefore, for circular optical fibers, I just need to solve the transcedental equation of (B-11), that was shown in the figure in my original post.
Since FEM is a more general way to cope with eigensystems with diverse operators and boundary conditions in arbitrary calculation region, now I'd like to use the FEM in mathematica to get the eigenvalue of $\beta ^2$ of $\nabla ^2 E(x,y)+\epsilon \left(\frac{2 \pi }{\lambda }\right)^2 = \beta^2 E(x,y)$, corresponding to the equation (A-7) underlined. Note that the eigenvalue is the square of the transcedental equation root in (B-11) underlined.
The codes in the newest post by user21 improves a lot compared in my original one, but I think it it still not right, since the calcutation result from COMSOL is exactly equal to the analytical solution.
Edit 4:
The following is the code for analytical solution.
λ = 1.55; ρ = 0.8; (* fiber core radius*)
ncore = 1.45;(*fiber core index*)
nclad = 1;(*fiber cladding index*)
s = 1;(*β for Subscript[HE, sm] or Subscript[EH, sm] mode*)
k = (2 π)/λ;
V = k ρ (ncore^2 - nclad^2)^(1/2); (*Print["V=",V];*)
U = ρ (k^2 ncore^2 - β^2)^(1/2); W = ρ (-k^2 nclad^2 + β^2)^(1/2);
Subscript[L, 1] = D[BesselJ[s, y], y]/(y BesselJ[s, y]) +
D[BesselK[s, z], z]/(z BesselK[s, z]);
Subscript[L, 2] = D[BesselJ[s, y], y]/(y BesselJ[s, y]) +
(nclad^2/ncore^2) (D[BesselK[s, z], z]/(z BesselK[s, z]));
lft = Subscript[L, 1 ] Subscript[L, 2] /. {y -> U, z -> W};
rght = ((s β)/(k ncore))^2 (V/(U W))^4;
bv = FindRoot[
lft == rght, {β,
Boole[V <= 2.405]*(k nclad + 10^-10) +
Boole[V > 2.405]*(k ncore - 10^-10), k nclad + 10^-10,
k ncore - 10^-10}];
Edit 5
Actually, some of the FEM model for fiber eigenmode analysis uses this expression to calculate the eigenfrequency of the modes. I'm sorry that I cann't understand this.
$\omega^2=\frac{\int\left[(\nabla\times H)^*{\varepsilon}(\nabla \times H)+\rho (\nabla \times H)^*(\nabla \times H)\right]\text{dx}\text{dy}}{\int \text{dxdy}H\mu H^*}$
I'm not sure it is an answer. But I'll post it here and waiting for elegant Mathematica codes.
All the above answers are very helpful. However, I'm afraid none of them is correct.
Here I find something that may be useful on the github. I hope this can work as a hint for elegant Mma codes.
I copied this theory part from here. It also included python codes.
I think models like this would be very helpful for non-expert users like me, and I don't know whether it is possible to include such a model in Mathematica, like in COMSOL. Actually, this is the real motivation for this question.
Theory (taken from the fenics book)
We are solving the Helmholtz equation:
$$\nabla \times \frac{1}{\mu_r}\nabla \times \boldsymbol{E} - k_0^2 \epsilon_r \boldsymbol{E}=0,$$ with the boundary condition of electric mirrors. $$k_0 = \frac{2\pi f_0}{c_0}$$
$$\epsilon_r = (n+\mathrm{i}k)^2$$
The functional is: $$F(\boldsymbol{E}) = \frac{1}{2} \int_\Omega \left[\frac{1}{\mu_r} \left(\nabla \times \boldsymbol{E}\right)\cdot \left(\nabla \times \boldsymbol{E}\right) - k^2_0 \epsilon_r \boldsymbol{E}\boldsymbol{E}\right]dx$$
In order to find it the vector is split in to the transverse and axial components.
$$\nabla = \nabla_t + \nabla_z,$$ with $$\nabla_t = \hat{e_x}\frac{\partial}{\partial x} + \hat{e_y}\frac{\partial}{\partial y}$$ and $$\nabla_z = \hat{e_z}\frac{\partial}{\partial z}$$ We also assume that the electric field can be split like: $$\boldsymbol{E}(x,y,z) = \left[E_t(x,y) + \hat{e_z} E_z(x,y) \right] e^{-\beta z}$$ where $\beta$ is the complex propagation constant, $$\boldsymbol{E}_t = \hat{e_x} E_x + \hat{e_y} E_y$$ and $$ \boldsymbol{E}_{axial} = \hat{e_z} E_z$$
By splitting the $\nabla$ and by substituting the above for the electric field the functional becomes: $$ F_{\beta}(\boldsymbol{E}) = \int_{\Omega} \frac{1}{\mu_r} \left(\nabla_t \times \boldsymbol{E}_{t,\beta}\right) \cdot \left(\nabla_t \times \boldsymbol{E}_{t,\beta}\right) -k^2_0 \epsilon_r E_{t,\beta}E_{t,\beta} \\ + \beta^2 \left[\frac{1}{\mu_r} \left(\nabla_t E_z+\beta E_{t,\gamma}\right)\left(\nabla_t E_z+\beta E_{t,\gamma}\right) - k^2_0 \epsilon_r E_z E_z dx \right]$$
Since the functional is built now the electric field components need to be discetized in order to be calculated. The axial component can be discretised by nodal basis functions (Lagrandge) but the transverse need to be by two dimensional curl-conforming vector basis functions(Nedelec).
The electric field in the nedelec space is described by: $$E_{t} = \sum_{i=1}^{N_{N}} (e_t)_i N_i$$
and the axial in lagrange elements as:
$$E_z = \sum_{i=1}^{N_{L}} (e_z)_i L_i$$ Where $N_N$ and $N_L$ are the number of nedelec and lagrange elements respectively. $N_i$, $L_i$ are the basis functions and $e_t$, $e_z$ are the equivalent coefficients.
While the cuttoff method is easier to implement it only prety much finds the cuttoff wavenumber (shown in fenics book and in other papers). This is not what is of interest. I am interested in calculating the propagation constants $\beta$ and by extension the effective indices and the Electric field that they equate to.
The matrix eqution to be solved is
$$\begin{bmatrix} A_{tt} & 0 \\ 0 & 0 \end{bmatrix}\begin{bmatrix} e_t \\ e_z \end{bmatrix} = -\beta^2 \begin{bmatrix} B_{tt} & B_{tz} \\ B_{zt} & B_{zz} \end{bmatrix}\begin{bmatrix} e_t \\ e_z \end{bmatrix} $$ Where $$A_{tt} = S_{tt} - k^2_0 T_{tt} = \int_{\Omega} \frac{1}{\mu_r} \left(\nabla_t \times N_i \right) \left(\nabla_t \times N_j \right)dx \\ - k^2_0 \int_{\omega} \epsilon_r N_i N_jdx$$
$$B_{zz} = S_{zz} - k^2_0 T_{zz} = \int_{\Omega} \frac{1}{\mu_r} \left(\nabla_t L_i \right) \left(\nabla_t L_j \right)dx \\ - k^2_0 \int_{\omega} \epsilon_r L_i L_j dx$$ $$ B_{tt} = \int_{\Omega} \frac{1}{\mu_r} N_i \cdot N_j dx$$$$ B_{tz} = \int_{\Omega} \frac{1}{\mu_r} N_i \cdot \nabla_t L_j dx$$$$ B_{zt} = \int_{\Omega} \frac{1}{\mu_r} \nabla_t L_i \cdot N_j dx$$
https://github.com/ibegleris/WaFEl/blob/master/Dispersion_analysis.ipynb