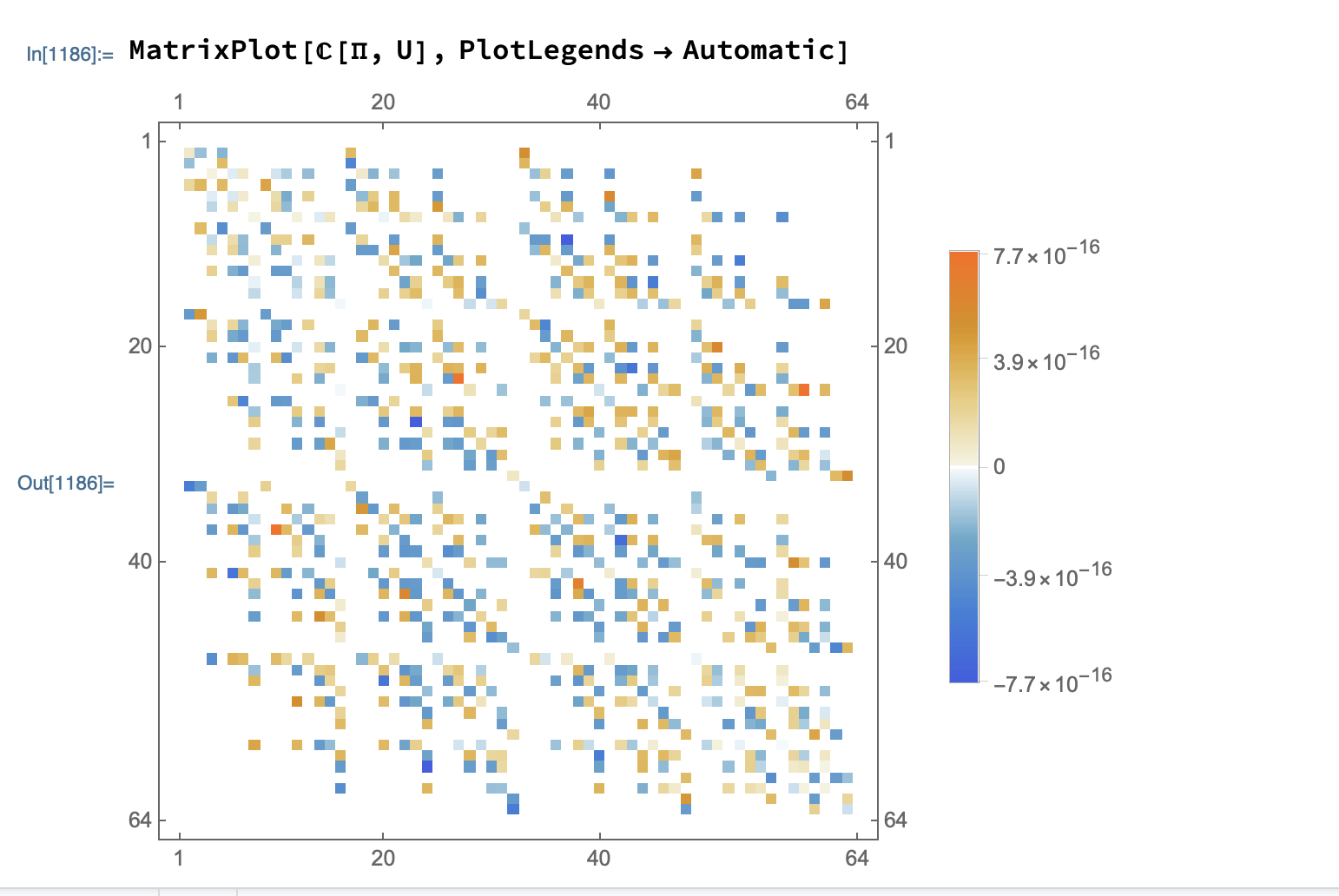
I've been doing some calcultations on mathematica which involve exponential of matrices. It's well known that if [A,B]=0 then [A,F(B)]=0 where A,B are matrices and [,] is their commutator. Nonetheless, I have a matrix A, which commutes with B and then I know that [A,MatrixExp[B]]=0, but Mathematica gives me a non-zero matrix... I've tried to chop it and it didn't work. So, what could be the source of this error? I checked for the commutator of [A,B] and Mathematica is indeed giving 0. Here is a picture of the matrix plot of the output:
I have a Hamiltonian which is parity invariant and the evolution operator U is not commuting with the parity operator (U=exp(-iHt)) and [Pi,H]=0.
Note: both operators have norm 1.
(Of course, this is just a sanity check of a much bigger calculation that is going wrong, I have a Hamiltonian which is parity invariant and the evolution operator U is not commuting with the parity operator).
Here are the two matrices:
A = SparseArray[{{1,1}->1,{2,33}->1,{3,17}->1,{4,49}->1,{5,9}->1,{6,41}->1,{7,25}->1,{8,57}->1,{9,5}->1,{10,37}->1,{11,21}->1,{12,53}->1,{13,13}->1,{14,45}->1,{15,29}->1,{16,61}->1,{17,3}->1,{18,35}->1,{19,19}->1,{20,51}->1,{21,11}->1,{22,43}->1,{23,27}->1,{24,59}->1,{25,7}->1,{26,39}->1,{27,23}->1,{28,55}->1,{29,15}->1,{30,47}->1,{31,31}->1,{32,63}->1,{33,2}->1,{34,34}->1,{35,18}->1,{36,50}->1,{37,10}->1,{38,42}->1,{39,26}->1,{40,58}->1,{41,6}->1,{42,38}->1,{43,22}->1,{44,54}->1,{45,14}->1,{46,46}->1,{47,30}->1,{48,62}->1,{49,4}->1,{50,36}->1,{51,20}->1,{52,52}->1,{53,12}->1,{54,44}->1,{55,28}->1,{56,60}->1,{57,8}->1,{58,40}->1,{59,24}->1,{60,56}->1,{61,16}->1,{62,48}->1,{63,32}->1,{64,64}->1}];
B = -I*SparseArray[{{1,1}->5,{2,2}->3,{2,3}->2,{3,2}->2,{3,3}->1,{3,5}->2,{4,4}->3,{4,6}->2,{5,3}->2,{5,5}->1,{5,9}->2,{6,4}->2,{6,6}->-1,{6,7}->2,{6,10}->2,{7,6}->2,{7,7}->1,{7,11}->2,{8,8}->3,{8,12}->2,{9,5}->2,{9,9}->1,{9,17}->2,{10,6}->2,{10,10}->-1,{10,11}->2,{10,18}->2,{11,7}->2,{11,10}->2,{11,11}->-3,{11,13}->2,{11,19}->2,{12,8}->2,{12,12}->-1,{12,14}->2,{12,20}->2,{13,11}->2,{13,13}->1,{13,21}->2,{14,12}->2,{14,14}->-1,{14,15}->2,{14,22}->2,{15,14}->2,{15,15}->1,{15,23}->2,{16,16}->3,{16,24}->2,{17,9}->2,{17,17}->1,{17,33}->2,{18,10}->2,{18,18}->-1,{18,19}->2,{18,34}->2,{19,11}->2,{19,18}->2,{19,19}->-3,{19,21}->2,{19,35}->2,{20,12}->2,{20,20}->-1,{20,22}->2,{20,36}->2,{21,13}->2,{21,19}->2,{21,21}->-3,{21,25}->2,{21,37}->2,{22,14}->2,{22,20}->2,{22,22}->-5,{22,23}->2,{22,26}->2,{22,38}->2,{23,15}->2,{23,22}->2,{23,23}->-3,{23,27}->2,{23,39}->2,{24,16}->2,{24,24}->-1,{24,28}->2,{24,40}->2,{25,21}->2,{25,25}->1,{25,41}->2,{26,22}->2,{26,26}->-1,{26,27}->2,{26,42}->2,{27,23}->2,{27,26}->2,{27,27}->-3,{27,29}->2,{27,43}->2,{28,24}->2,{28,28}->-1,{28,30}->2,{28,44}->2,{29,27}->2,{29,29}->1,{29,45}->2,{30,28}->2,{30,30}->-1,{30,31}->2,{30,46}->2,{31,30}->2,{31,31}->1,{31,47}->2,{32,32}->3,{32,48}->2,{33,17}->2,{33,33}->3,{34,18}->2,{34,34}->1,{34,35}->2,{35,19}->2,{35,34}->2,{35,35}->-1,{35,37}->2,{36,20}->2,{36,36}->1,{36,38}->2,{37,21}->2,{37,35}->2,{37,37}->-1,{37,41}->2,{38,22}->2,{38,36}->2,{38,38}->-3,{38,39}->2,{38,42}->2,{39,23}->2,{39,38}->2,{39,39}->-1,{39,43}->2,{40,24}->2,{40,40}->1,{40,44}->2,{41,25}->2,{41,37}->2,{41,41}->-1,{41,49}->2,{42,26}->2,{42,38}->2,{42,42}->-3,{42,43}->2,{42,50}->2,{43,27}->2,{43,39}->2,{43,42}->2,{43,43}->-5,{43,45}->2,{43,51}->2,{44,28}->2,{44,40}->2,{44,44}->-3,{44,46}->2,{44,52}->2,{45,29}->2,{45,43}->2,{45,45}->-1,{45,53}->2,{46,30}->2,{46,44}->2,{46,46}->-3,{46,47}->2,{46,54}->2,{47,31}->2,{47,46}->2,{47,47}->-1,{47,55}->2,{48,32}->2,{48,48}->1,{48,56}->2,{49,41}->2,{49,49}->3,{50,42}->2,{50,50}->1,{50,51}->2,{51,43}->2,{51,50}->2,{51,51}->-1,{51,53}->2,{52,44}->2,{52,52}->1,{52,54}->2,{53,45}->2,{53,51}->2,{53,53}->-1,{53,57}->2,{54,46}->2,{54,52}->2,{54,54}->-3,{54,55}->2,{54,58}->2,{55,47}->2,{55,54}->2,{55,55}->-1,{55,59}->2,{56,48}->2,{56,56}->1,{56,60}->2,{57,53}->2,{57,57}->3,{58,54}->2,{58,58}->1,{58,59}->2,{59,55}->2,{59,58}->2,{59,59}->-1,{59,61}->2,{60,56}->2,{60,60}->1,{60,62}->2,{61,59}->2,{61,61}->3,{62,60}->2,{62,62}->1,{62,63}->2,{63,62}->2,{63,63}->3,{64,64}->5}]/10;