I want to solve numerically the following differential equation
$$ y''(x) + \frac{3}{x}y'(x) = \frac{d U(y)}{y}\,,\qquad U(y) = \frac{1}{4}y(x)^2(y(x)-1)^2 -\frac{1}{400}y(x)^3 $$
in the region $x\in[0,+\infty]$ with the bounce boundary conditions
$$ y'(0) = 0\,,\qquad y(+\infty) = 0. $$
This problem is taken from Coleman's paper (click here) where he claims that such solution always exists, see page 4, right-column independently of the expression of the potential $U(y)$.
I want to find such solution for the potential that I have chosen.
This is a boundary-values problem and it is better to turn it into a initial-values problem. Let us then estimate the size of $y(0)$. It will become clear that I expect a flat direction around $x=0$, so substituting $y'(0)=0$ and $y''(0) = 0$ in the differential equation, I get an estimate for the initial condition $y(0) \sim 1$. This is just an estimate. I don't want the exact solution to satisfy $y''(0)=0$ identically, but I expect the second derivative to be small.
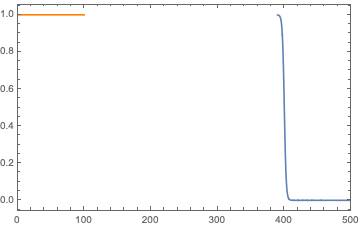
We can try to guess the form of the solution by making some approximations. Indeed, I can solve analytically the differential equation for big values of $x\geq R \gg 1$, by neglecting the $1/400y(x)^3$ term in the expression of $U(y)$, as $y(0) \sim 1$ and $y(+\infty) = 0$; then, by defining $U_0(y) = \frac{1}{4}y(x)^2(y(x)-1)^2$ we can solve
$$ y''(x)= \frac{d U_0(y)}{y}\, $$
from which we get
$$ y_R(x) = \frac{1}{2}\left(1-\text{tanh}\left[\frac{x-R}{2\sqrt{2}}\right]\right). $$
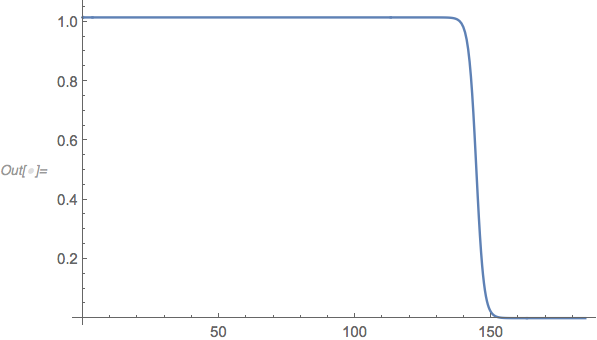
So, roughly the approximated solution is
$$ y(x) = \left\{\begin{array}{c c} 1 & x \ll R\\ y_R(x) & x \geq R \end{array}\right. $$ and it is shown in the following picture for $R=400$
So, I have tried to solve numerically this Cauchy problem without any approximation by setting a cut-off $R$ on the $x$ coordinates and using the following code
V[y_[x_]] = 1/4 (-1 + y[x])^2 y[x]^2 - 0.0025 y[x]^3
Psol = ParametricNDSolveValue[{D[y[x], {x, 2}] + 3/x D[y[x], x] == D[V[y[x]], y[x]], y'[10^-5] == 0, y[10^-5] == tp, WhenEvent[y[x] < 0 || y[x] > tp, "StopIntegration"]}, y, {x, 10^-5, R}, {tp, R}]
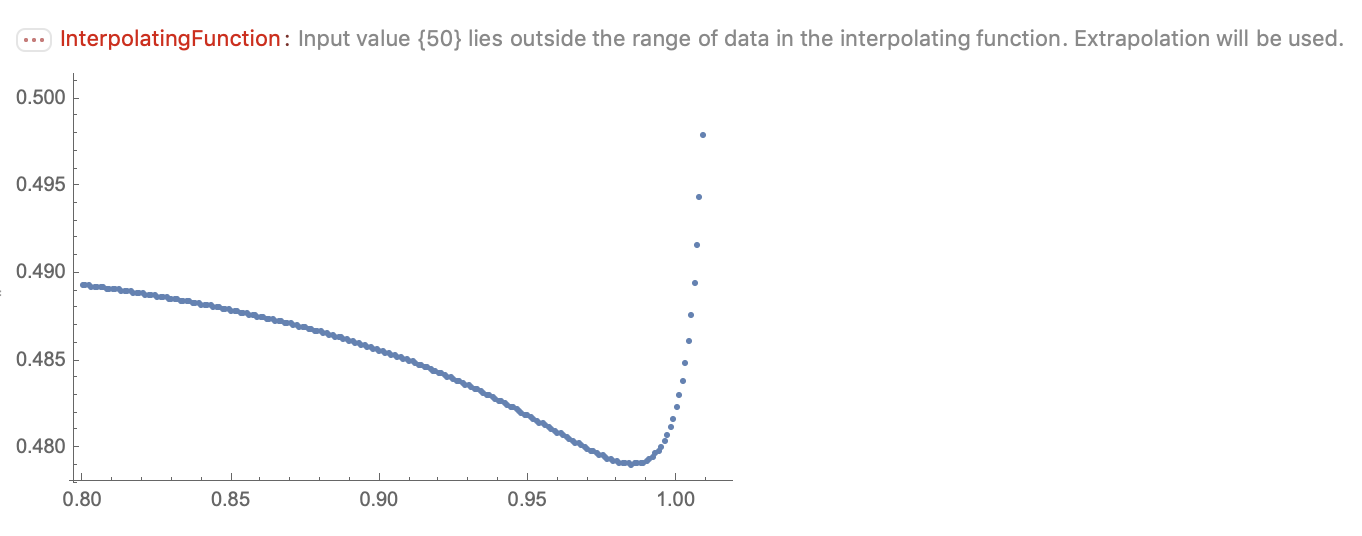
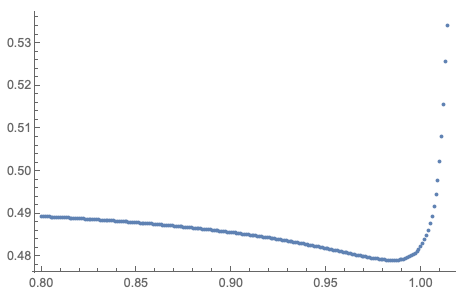
ListPlot[Table[{tp, Abs[Psol[tp, 50][50]]}, {tp, 0.8, 1.015, 0.001}]]
where the last line may be useful to determine the magnitude of tp such that I recover a vanishing asymptotic value. However, I get the following plot
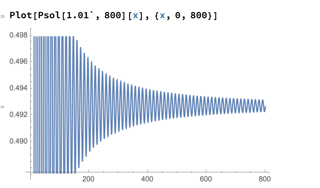
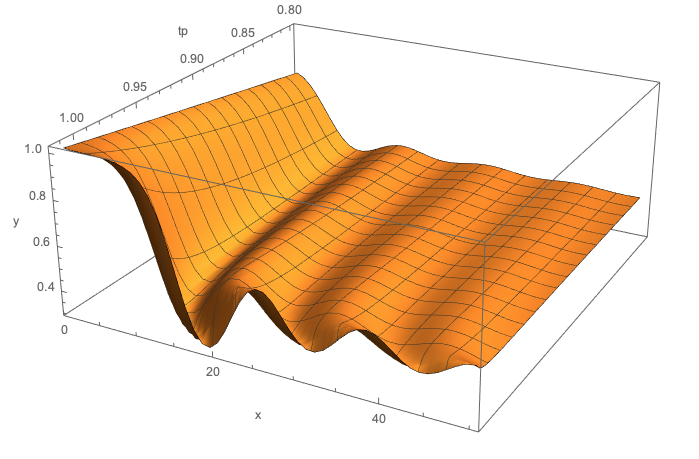
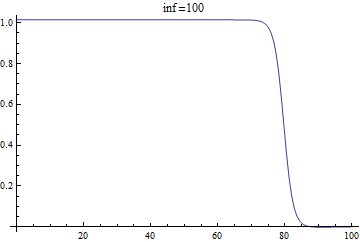
So, I guess the method is not suitable for this problem. In particular, the solution Mathematica finds does not respect the asymptotic boundary condition $y(+\infty) = 0$ at $x=50$, and moreover it starts oscillating after some time like in the following picture and does not go to zero at infinity
How can I get the numerical solution?

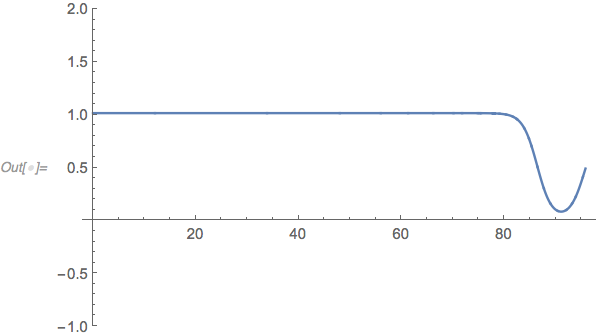

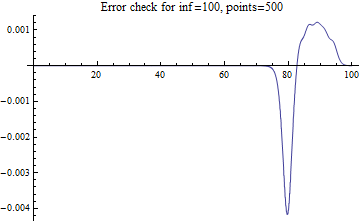
NDSolveis very robust, the result given by your code only suggests one thing in my view: there just exists no solution satisfying $y(0)∼1,y′(0)=0,y(+∞)=0$. $\endgroup$