I have a data set comprising of three columns:
Column A: Position in $x$ direction ($x_p$)
Column B: Position in $y$ direction ($y_p$)
Column C: Mass ($m_p$) at position $(x_p,y_p)$
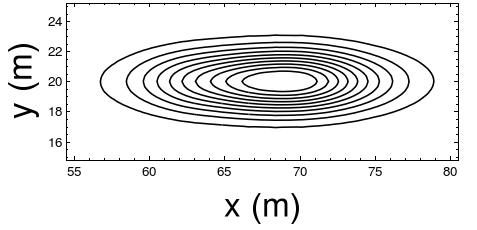
I am trying to represent this data in terms of PDF calculated via kernel density estimation. So far, I have been able to calculate the PDF using only two variables (i.e, position in $x$ and position in $y$) through the smooth kernel distribution functionality. The resulting PDF looks like this:
I would now like to include the third column into this PDF. So, mathematically I am interested in computing the following:
$$m(x,y)=\frac1{h^2}\sum_{p=1}^n m_p(x_p,y_p)K\left(\frac{x-x_p}{h},\frac{y-y_p}{h}\right)$$
where $p$ refers to the number of samples, $h$ is the bandwidth and $K$ is the smoothing kernel.
Could anyone please let me know if it possible to calculate such a PDF (or mass density here) using the SmoothKernelDensity functionality?
Any help would be much appreciated. Thank you.