I'm trying to solve 2 coupled nonlinear ODEs using NDSolve, but the solution fails when the parameter $\lambda$ increases. The equations are $$r^2 a''(r)= a(r) [a(r)-1] [a(r)-2]- r^2 [1-a(r)] h(r)^2,$$ $$\left(r^2 h'(r)\right)'= 2 [1-a(r)]^2 h(r)+ \lambda\,r^2 [h(r)^2-1]\, h(r).$$ And the boundary conditions are $$a(0)=h(0)=0,$$ $$a(\infty)= h(\infty)=1.$$ I've tried to solve it in the following way:
\[Lambda] = 0.1;
r1 = 10^(-6); r2 = 9.5;
eqn = {r^2*D[D[a[r], r], r] == a[r]*(a[r] - 1)*(a[r] - 2) - r^2*h[r]^2*(1 - a[r]),
D[r^2*D[h[r], r], r] == 2*h[r]*(1 - a[r])^2 + \[Lambda]*r^2*(h[r]^2 - 1)*h[r]};
bc = {a[r1] == 0, a[r2] == 1, h[r1] == 0, h[r2] == 1};
sol = NDSolveValue[{eqn, bc} /. {a -> (#1^2*g[#1] & ), h -> (#1*j[#1] & )}, {g, j}, {r, r1, r2},
Method -> {"ExplicitRungeKutta", "DifferenceOrder" -> 5}, WorkingPrecision -> MachinePrecision];
Plot[{1, 1 - r^2*sol[[1]][r], r*sol[[2]][r]}, {r, r1, r2}]
And, indeed, I get a solution.
This works for $\lambda=0$ and $\lambda=0.1$. However, I would like to get the solution for a larger range of $\lambda$, for example $\lambda=1$. When I try to use the code above and set $\lambda=1$ Mathematica gives me the following
NDSolveValue: At r == 1.2395178650126792`, step size is effectively zero; singularity or stiff system suspected.
NDSolveValue: The scaled boundary value residual error of 62.94578090521123` indicates that the boundary values are not satisfied to specified tolerances. Returning the best solution found.
As a consequence, the plot does not correspond to a solution.
So, my question is: How can I get a solution to these equations when $\lambda=1$, for example? Is it possible to calculate for larger values?
Besides that, how can I use the results of NDSolve in an integral of the form
$$E=4\pi \int_{0}^{\infty}dr\,\left[(a')^2 + \frac{1}{2}\frac{a^2(a-2)^2}{r^2}+ \frac{1}{2}r^2(h')^2 + h^2(a-1)^2 + \frac{\lambda}{4}r^2(h^2-1)^2 \right]?$$
I know this problem has a solution, because the equations are those of the 't Hooft-Polyakov monopole and the last integral is its mass as a function of $\lambda$. But, I'm new in Mathematica and I don't know how to correctly reproduce the solutions which are available at textbooks.
Any hint is valuable to me!
Thanks in advance.
***Update:***Just to make it clear, I would like to stress that I know this problem has been solved. The plots of the solution are available at textbooks on topological solitons, such as [Manton, Sutcliffe] and [Shnir]. What I'm trying to say is that I would like to understand how to obtain this solution in Mathematica. The question marked as a possible duplicate is the solution of the Abelian string (also ANO vortex), which is different. One can see this when looking, for example, into the kinetic part given in those terms with derivatives. Could you, please, give some hints on how I should improve my code?

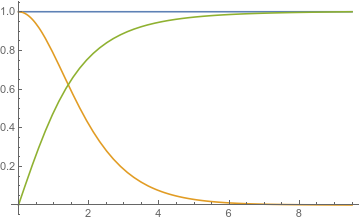
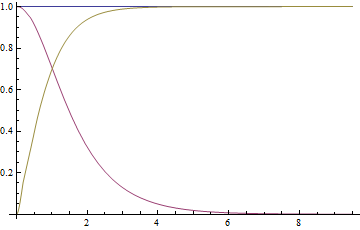

"Shooting"method and adjust"StartingInitialConditions". You can use a nearby solution for them. A lambda that works may be increased a little and a new solution produced. Little by little you might be able to push lambda up to1. $\endgroup$