How to draw a certain region and calculate its area and perimeter?

Question

How can I draw the figure shown above in rectangular coordinates, calculate the area and perimeter of the shaded region as a function of radius r of the outer circle, and find the points of intersection of the inner circles.

Answer 1

Show it:

RegionPlot[
 region = RegionUnion[
   Sequence @@ 
    RegionIntersection @@@ 
     Subsets[{Disk[{-1, 0}], Disk[{0, -1}], Disk[{1, 0}], 
       Disk[{0, 1}]}, {2}], 
   Fold[RegionDifference, {Disk[{0, 0}, 2], Disk[{-1, 0}], 
     Disk[{0, -1}], Disk[{1, 0}], Disk[{0, 1}]}]], Frame -> False]

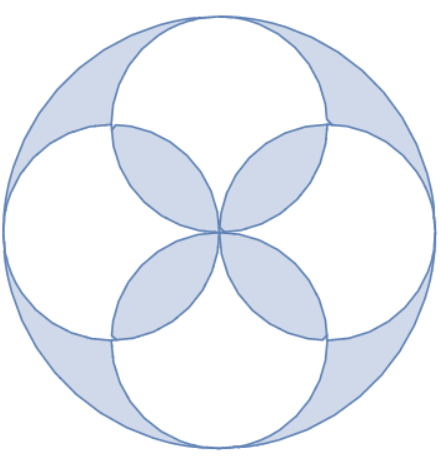
Area

Area[region]

4 (-2 + π)

Perimeter

ArcLength@RegionBoundary[region]

12 π

Answer 2

I would use BooleanRegion:

reg = BooleanRegion[
    Xor,
    {Disk[{-1,0},1], Disk[{0,1},1], Disk[{1,0},1], Disk[{0,-1},1], Disk[{0,0},2]}
];

RegionMeasure @ reg

RegionMeasure @ RegionBoundary @ reg

4 (-2 + Pi)

12 Pi

Answer 3

Your question wants a relationship for r which I assume is the radius of the larger circle. You can get it like this:

c1 = ImplicitRegion[(x - r)^2 + y^2 <= r^2, {x, y}];
c2 = ImplicitRegion[x^2 + (y - r)^2 <= r^2, {x, y}];
Assuming[r > 0, Area[RegionIntersection[c1, c2]]]

yields

$\frac{1}{2} (\pi -2) r^2$

All the shaded areas in terms of r:

FullSimplify[\[Pi]*r^2 - 4 \[Pi] (r/2)^2 + 8 1/8 (-2 + \[Pi]) r^2]

$\left ( \pi -2\right )r^2$

Testing for the particular answer given by yode:

(-2 + \[Pi]) r^2 /. r -> 2

yields

$4\pi - 8$

Answer 4

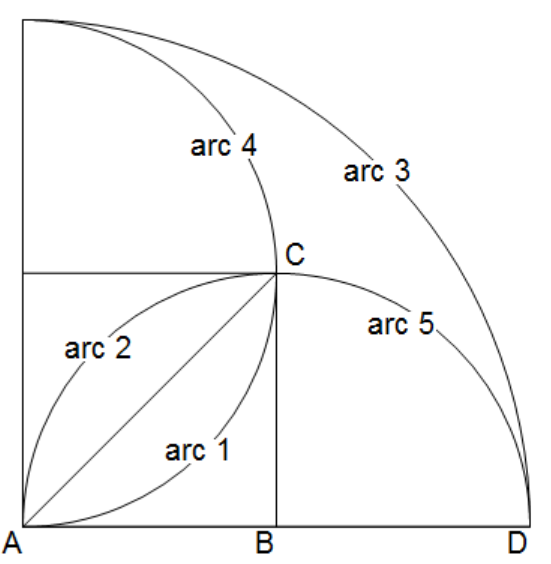
Just for fun. By symmetry we need only consider the first quadrant.

Graphics[{EdgeForm[Black], FaceForm[None], Disk[{0, 0}, 2, {0, Pi/2}],
   Disk[{1, 0}, 1, {0, Pi}], Disk[{0, 1}, 1, {-Pi/2, Pi/2}], 
  Line[{{1, 0}, {1, 1}}], Line[{{0, 0}, {1, 1}}], 
  Line[{{0, 1}, {1, 1}}],
  Text[Style["A", 20], {0, 0}, {1, 1}],
  Text[Style["B", 20], {1, 0}, {1, 1}],
  Text[Style["C", 20], {1, 1}, {-2, -1}],
  Text[Style["D", 20], {2, 0}, {1, 1}],
  Text[Style["arc 1", 20, Background -> White], {0.7, 0.3}, {0, 0}],
  Text[Style["arc 2", 20, Background -> White], {0.3, 0.7}, {0, 0}],
  Text[Style["arc 3", 20, Background -> White], {1.4, 1.4}, {0, 0}],
  Text[Style["arc 4", 20, Background -> White], {0.8, 1.5}, {0, 0}],
  Text[Style["arc 5", 20, Background -> White], {1.5, 0.8}, {0, 0}]
  }]

Let the radius of the small circle be 1 (hence the radius of the large circle is 2).

So the perimeter can be seen to be length of arc1+ arc2+arc3+arc4+arc5:

Let $p_i$ represent arc i length. Now $p_1=p_2=p_4=p_5= \pi/2$ and arc length $p3= \pi/2 \times 2$. Hence total perimeter:

perimeter = 4 (4 Pi/2 + Pi/2 2)

i.e. $12\pi$

For the area: area bounded by arc1 and arc 2 is 2 x (area of sector-area of triangle ABC):

area1 = 2 (Pi/4 - 1/2)

The area bounded by arcs 3,4 and 5= area of quarter circle -area of 2 semicircles+ area of overlap:

area2 = (Pi/2) 2^2/2 - Pi + area1

Note area1=area2=$\pi/2-1$, so the total area is

total = Simplify[4 (area1 + area2)]

yielding:

4 (-2 + \[Pi])

Answer 5

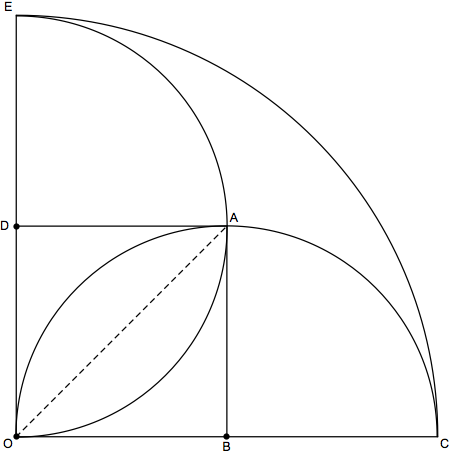
This isn't really a Mathematica problem. It is a Euclidean geometry problem and can be solve by a little classic geometry reasoning. Like ubpdqn I will work in the 1st quadrant and invoke symmetry.

By construction

$\qquad$OC = OE = R
$\qquad$OB = OD = DA = BA = R/2

By observation

$\qquad$Quadrant perimeter = EA + AO + OA + BA + EC
$\qquad$OBAD is a square

Arcs EA + OA and AO, + BA are one half the circumference of the equal circles centered at B and D, which have diameters R/2, so EA + OA + AO, + BA = circumference of an inner circle = π R. EC is one quarter of the circumference of the outer circle, so EC = (2 π R)/4 = π R/2. The quadrant perimeter is therefore π R + π R/2 = 3 π R/2.

It follows that the full perimeter, 4 x (quadrant perimeter), is 6 π R.

Point A is one of the points where the inner circles intersect and it clearly lies at {R/2, R/2}. By symmetry, the four points of intersection are

$\qquad${{R/2, R/2}, {-R/2, R/2}, {-R/2, -R/2}, {R/2, -R/2}}.

Finding the area is a little more complicated, but not much.

The area, a1, between the two arcs ending at points O and A is clearly twice the difference of the area between the arc OA and the dashed line OA. This in turn is the area of a quadrant of inner circle centered at B less the half the square OBAD. Thus,

$\qquad$a1 = 2 ((π (R/2)^2)/4 - ((R/2)^2)/2) = 1/8 (π - 2) R^2

The area, a2, bordered by the arcs EC, EA and AC is the area of the quadrant less the area of 2 quadrants of an inner circle less the area of the square OBAD. This is given by

$\qquad$a2 = (π R^2)/4 - (π (R/2)^2)/2 - (R/2)^2 = 1/8 (π - 2) R^2

Note that a1 = a2 (which I find an interesting result in itself). Therefore, the full area is

$\qquad$4 (2 a1) = (π - 2) R^2