6
$\begingroup$

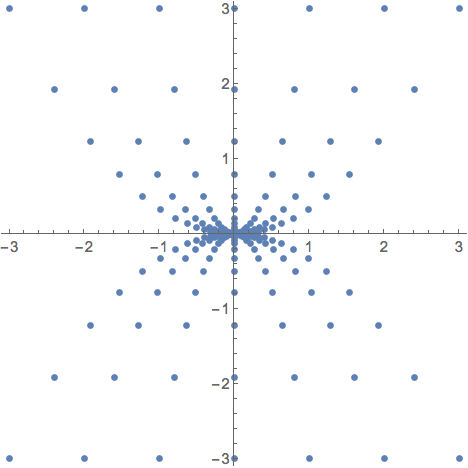
The following image contains solution trajectories of the system:

$$\vec x_{k+1}= \begin{bmatrix} 0.80 & 0\\ 0 & 0.64 \end{bmatrix}\,\vec x_k $$ I've managed to do this:

Clear[A, pts1, pts2, pts]
A = {{.80, 0}, {0, .64}}; 
pts1 = Table[NestList[A.# &, {k, 3}, 20], {k, -3, 3}];
pts2 = Table[NestList[A.# &, {k, -3}, 20], {k, -3, 3}];
pts = Flatten[Join[pts1, pts2], 1];
ListPlot[pts,
 PlotStyle -> PointSize[Medium],
 PlotRange -> {{-3.1, 3.1}, {-3.1, 3.1}},
 AspectRatio -> Automatic]

Which gives this image:

enter image description here

What I would like to learn how to do is to include a smooth curve for each trajectory, points of the trajectory lying on the curve, and a little arrow on each curve pointing in the direction of the origin.

I'd appreciate any help.

Thanks.

Update: Thanks to some nice help from cyrille.piatecki and Sumit, I was able to produce this:

Clear[A, pts1, pts2, pts]
A = {{.80, 0}, {0, .64}};
pts1 = Table[NestList[A.# &, {k, 3}, 10], {k, -3, 3}];
pts2 = Table[NestList[A.# &, {k, -3}, 10], {k, -3, 3}];
pts = Join[pts1, pts2];
Show[
 ListLinePlot[pts,
   InterpolationOrder -> 2,
   AxesLabel -> {"\!\(\*SubscriptBox[\(x\), \(1\)]\)", 
     "\!\(\*SubscriptBox[\(x\), \(2\)]\)"},
   PlotRange -> {{-3.3, 3.3}, {-3.1, 3.1}},
   AspectRatio -> Automatic,
   Epilog -> {
     Text[
      Style["\!\(\*SubscriptBox[\(x\), \(0\)]\)", 12, Black], {3, 
       3}, {-1, 1}],
     Text[
      Style["\!\(\*SubscriptBox[\(x\), \(1\)]\)", 12, Black], {2.4, 
       1.92}, {-1, 1}],
     Text[
      Style["\!\(\*SubscriptBox[\(x\), \(2\)]\)", 12, Black], {1.92, 
       1.23}, {-1, 1}],
     Text[
      Style["\!\(\*SubscriptBox[\(x\), \(0\)]\)", 12, Black], {-3, 
       3}, {1, 1}],
     Text[
      Style["\!\(\*SubscriptBox[\(x\), \(1\)]\)", 12, Black], {-2.4, 
       1.92}, {1, 1}],
     Text[
      Style["\!\(\*SubscriptBox[\(x\), \(2\)]\)", 12, Black], {-1.92, 
       1.23}, {1, 1}]
     }] /. Line[x_] -> {Arrowheads[{0., 0.04, 0.}], Arrow[x]},
 ListPlot[pts,
  PlotStyle -> PointSize[0.015]]
 ]

Which produced this image:

enter image description here

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ What is the initial conditions of the system? i.e. what is x at time zero? $\endgroup$
    – Nasser
    Commented Jul 9, 2016 at 7:03
  • $\begingroup$ @nasser I believe so. This is from Lay's Linear Algebra and its applications book. I started 7 trajectories at the top edge and 7 I started from the bottom edge. $\endgroup$
    – David
    Commented Jul 9, 2016 at 16:43

2 Answers 2

Reset to default
9
$\begingroup$

Avoid the Flatten. It will preserve the distinct lines.

pts = Join[pts1, pts2];
ListLinePlot[Evaluate@pts] /. Line[x__] :> {Arrowheads[{0., 0.05, 0.}], Arrow[x]}

enter image description here

For smootness you can use InterpolationOrder with ListLinePlot.

Improve this answer
$\endgroup$
7
$\begingroup$

I hope this 

Clear[A, pts1, pts2, pts]
A = {{.80, 0}, {0, .64}};
pts1 = Table[NestList[A.# &, {k, 3}, 20], {k, -3, 3}];
pts2 = Table[NestList[A.# &, {k, -3}, 20], {k, -3, 3}];
p1 = ListLinePlot[pts1, PlotStyle -> PointSize[Medium], 
PlotRange -> {{-3.1, 3.1}, {-3.1, 3.1}}, 
AspectRatio -> Automatic] /. 
Line[x_] :> {Arrowheads[{0, 0.04, 0}], Arrow[x]};
p2 = ListLinePlot[pts2, PlotStyle -> PointSize[Medium], 
PlotRange -> {{-3.1, 3.1}, {-3.1, 3.1}}, 
AspectRatio -> Automatic] /. 
Line[x_] :> {Arrowheads[{0, 0.04, 0}], Arrow[x]};
Show[p1, p2]

Which gives this

enter image description here

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.