0
$\begingroup$

I'm integrating a product of two functions which is bounded on the interval from -Inf to 0, and diverges from 0 to +Inf. The error that I get says that the integral diverges on {0,+Inf}... How did those bounds get included?

The result[t] function includes a sum of well-defined Hypergeometric functions which are also part of the error message. The approx[t] has some symbolic code in it but it hasn't caused any problems with Integrate in the past.

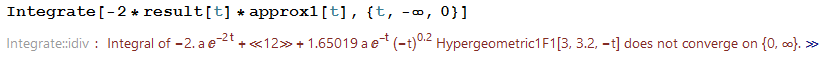
enter image description here

The integrand looks like this: enter image description here

The "a" that you see throughout is a symbolic constant. It's got no value associated with it right now.

I see how the integrand should technically diverge when t approaches zero because of the inverse powers, but if we take a look at what the function actually looks like on a plot there doesn't seem to be a problem at t = 0 (that's why I'm hoping the integral will work):

enter image description here

Thanks for any help!

Improve this question
$\endgroup$
3
  • $\begingroup$ Welcome to Mathematica.SE! I hope you will become a regular contributor. To get started, 1) take the introductory Tour now, 2) when you see good questions and answers, vote them up by clicking the gray triangles, because the credibility of the system is based on the reputation gained by users sharing their knowledge, 3) remember to accept the answer, if any, that solves your problem, by clicking the checkmark sign, and 4) give help too, by answering questions in your areas of expertise. $\endgroup$
    – bbgodfrey
    Commented Jul 10, 2015 at 19:03
  • $\begingroup$ Please provide the integrand, so that readers can experiment with it. $\endgroup$
    – bbgodfrey
    Commented Jul 10, 2015 at 19:11
  • $\begingroup$ People here generally like users to post code as Mathematica code instead of images or TeX, so they can copy-paste it. It makes it convenient for them and more likely you will get someone to help you. You may this this meta Q&A helpful $\endgroup$
    – Michael E2
    Commented Jul 11, 2015 at 4:24

1 Answer 1

Reset to default
0
$\begingroup$

When evaluating an integral, Mathematica applies a series of change-of-variables substitutions in attempt to put this integral in a standard from. Likely ehat has happened here is that Mathematica has performed a substitution like $t\to -t$ which has the effect of changing the bounds of integration. The resulting integral does not converge which almost certainly means that your original integral doe snot converge either. If you have reason to believe that your integral does converge, you could try integrating term by term.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Welcome to Mathematica.SE! I hope you will become a regular contributor. To get started, 1) take the introductory Tour now, 2) when you see good questions and answers, vote them up by clicking the gray triangles, because the credibility of the system is based on the reputation gained by users sharing their knowledge, 3) remember to accept the answer, if any, that solves your problem, by clicking the checkmark sign, and 4) give help too, by answering questions in your areas of expertise. $\endgroup$
    – bbgodfrey
    Commented Jul 10, 2015 at 19:07
  • 1
    $\begingroup$ Please provide more detail to substantiate how you arrived at this conclusion. What the OP might do to work around this issue also would be helpful. $\endgroup$
    – bbgodfrey
    Commented Jul 10, 2015 at 19:08
  • 1
    $\begingroup$ "integrating term by term" - not always a good idea; consider $$\int_0^\infty \frac{1-\cos x}{x^2} \mathrm dx$$ $\endgroup$
    – J. M.'s missing motivation ♦
    Commented Jul 11, 2015 at 1:38
  • $\begingroup$ Alex S, is there a way of avoiding this change of variables? $\endgroup$
    – Buddhapus
    Commented Jul 13, 2015 at 17:36

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.