5
$\begingroup$

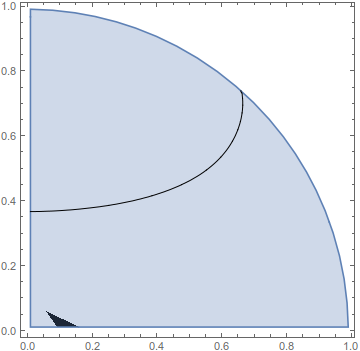
I would like to define a region in (x,y) by combining the region

RegionPlot[
 ImplicitRegion[
  Sqrt[x^2 + y^2] <= 0.99, {{x, 0.01, 0.99}, {y, 0.01, 0.99}}]]

with a curve that I obtain in the form of following list of data points

{{0.01, 0.365965}, {0.0213949, 0.366105}, {0.040625, 
  0.366414}, {0.0608803, 0.36713}, {0.07125, 0.367416}, {0.0809226, 
  0.367827}, {0.101875, 0.368985}, {0.125746, 0.370746}, {0.1325, 
  0.371144}, {0.138417, 0.371583}, {0.163125, 0.373914}, {0.193536, 
  0.377286}, {0.19375, 0.377306}, {0.193925, 0.377325}, {0.195267, 
  0.3775}, {0.221223, 0.380652}, {0.224375, 0.380995}, {0.228669, 
  0.381794}, {0.248015, 0.384485}, {0.255, 0.385685}, {0.264962, 
  0.387462}, {0.270313, 0.38829}, {0.274217, 0.388908}, {0.285625, 
  0.391134}, {0.294023, 0.392813}, {0.299857, 0.393893}, {0.302655, 
  0.39453}, {0.31625, 0.397271}, {0.325268, 0.399107}, {0.342134, 
  0.403384}, {0.346875, 0.404435}, {0.3499, 0.4051}, {0.360713, 
  0.408125}, {0.362188, 0.408504}, {0.374082, 0.411543}, {0.3775, 
  0.412638}, {0.384084, 0.414709}, {0.385761, 0.415176}, {0.392813, 
  0.417185}, {0.397525, 0.418725}, {0.406377, 0.42169}, {0.408125, 
  0.422209}, {0.409043, 0.422519}, {0.411678, 0.423437}, {0.420492, 
  0.426383}, {0.423437, 0.427397}, {0.42989, 0.42989}, {0.431678, 
  0.43051}, {0.43875, 0.433049}, {0.442822, 0.434678}, {0.452636, 
  0.43875}, {0.454063, 0.439324}, {0.455115, 0.439802}, {0.464461, 
  0.443664}, {0.469375, 0.445785}, {0.483029, 0.452404}, {0.484688, 
  0.453122}, {0.48536, 0.45339}, {0.486568, 0.454063}, {0.5, 
  0.460777}, {0.50554, 0.463835}, {0.515072, 0.469375}, {0.515226, 
  0.469462}, {0.515313, 0.469513}, {0.515682, 0.469745}, {0.524883, 
  0.475117}, {0.530625, 0.478686}, {0.534272, 0.481041}, {0.539593, 
  0.484688}, {0.54339, 0.487235}, {0.545938, 0.489192}, {0.550279, 
  0.492344}, {0.552195, 0.493743}, {0.553594, 0.494799}, {0.560303, 
  0.5}, {0.560834, 0.500416}, {0.56125, 0.500753}, {0.565057, 
  0.503849}, {0.565319, 0.504069}, {0.568906, 0.507039}, {0.569244, 
  0.507319}, {0.569639, 0.507656}, {0.576563, 0.513701}, {0.577418, 
  0.514457}, {0.578351, 0.515313}, {0.584219, 0.520827}, {0.585319, 
  0.521869}, {0.58644, 0.522969}, {0.591875, 0.528476}, {0.592941, 
  0.529559}, {0.593956, 0.530625}, {0.599531, 0.536731}, {0.600273, 
  0.537539}, {0.600932, 0.538281}, {0.607188, 0.545704}, {0.607295, 
  0.54583}, {0.607384, 0.545938}, {0.608063, 0.546813}, {0.614109, 
  0.554328}, {0.614844, 0.555443}, {0.619151, 0.56125}, {0.620514, 
  0.563236}, {0.6225, 0.566235}, {0.624335, 0.568906}, {0.626568, 
  0.572494}, {0.627296, 0.573702}, {0.629129, 0.576563}, {0.630156, 
  0.578344}, {0.632369, 0.582006}, {0.63354, 0.584219}, {0.633984, 
  0.585035}, {0.635102, 0.586929}, {0.63745, 0.591513}, {0.637653, 
  0.591875}, {0.637706, 0.591982}, {0.637813, 0.592202}, {0.641602, 
  0.599531}, {0.642769, 0.602231}, {0.644448, 0.606167}, {0.644918, 
  0.607188}, {0.645079, 0.607577}, {0.645469, 0.608516}, {0.646554, 
  0.611016}, {0.647236, 0.612783}, {0.647323, 0.61299}, {0.648103, 
  0.614844}, {0.649297, 0.618047}, {0.649473, 0.618495}, {0.649543, 
  0.618672}, {0.649671, 0.619046}, {0.650517, 0.62128}, {0.650985, 
  0.6225}, {0.651513, 0.624112}, {0.651813, 0.625016}, {0.652298, 
  0.626328}, {0.653125, 0.628936}, {0.653433, 0.629848}, {0.65354, 
  0.630156}, {0.653709, 0.63074}, {0.654768, 0.633984}, {0.65531, 
  0.635627}, {0.65588, 0.637813}, {0.656856, 0.641543}, {0.656901, 
  0.641693}, {0.656953, 0.641915}, {0.65792, 0.645469}, {0.658532, 
  0.647718}, {0.659354, 0.651698}, {0.659704, 0.653125}, {0.659915, 
  0.653992}, {0.660453, 0.656953}, {0.660499, 0.657235}, {0.660781, 
  0.658789}, {0.660797, 0.658867}, {0.661108, 0.660455}, {0.661163, 
  0.660781}, {0.661238, 0.661238}, {0.661689, 0.663702}, {0.661828, 
  0.664609}, {0.662013, 0.665841}, {0.66221, 0.667009}, {0.662409, 
  0.668438}, {0.662664, 0.67032}, {0.662672, 0.670374}, {0.663022, 
  0.672266}, {0.663131, 0.673744}, {0.663237, 0.674721}, {0.663407, 
  0.676094}, {0.663534, 0.677169}, {0.663705, 0.679017}, {0.663802, 
  0.679922}, {0.663877, 0.680654}, {0.664074, 0.683215}, {0.664123, 
  0.68375}, {0.664161, 0.684198}, {0.664353, 0.687322}, {0.664372, 
  0.687578}, {0.664387, 0.6878}, {0.664547, 0.691344}, {0.664555, 
  0.691406}, {0.664609, 0.693416}, {0.664668, 0.695175}, {0.664669, 
  0.695234}, {0.66467, 0.695295}, {0.66472, 0.698952}, {0.66472, 
  0.699063}, {0.664719, 0.699172}, {0.664693, 0.702807}, {0.664691, 
  0.702891}, {0.664689, 0.702971}, {0.664609, 0.705682}, {0.664585, 
  0.706694}, {0.664587, 0.706719}, {0.664584, 0.706745}, {0.664427, 
  0.710364}, {0.664418, 0.710547}, {0.664406, 0.71075}, {0.664196, 
  0.713962}, {0.664165, 0.714375}, {0.664121, 0.714864}, {0.663883, 
  0.717477}, {0.663808, 0.718203}, {0.663706, 0.719107}, {0.663521, 
  0.720943}, {0.663396, 0.722031}, {0.663258, 0.723383}, {0.663224, 
  0.724474}, {0.663097, 0.725859}, {0.662973, 0.727496}, {0.663114, 
  0.728192}, {0.66275, 0.729688}, {0.662729, 0.729721}, {0.662695, 
  0.729774}, {0.662198, 0.731105}, {0.662026, 0.731602}, {0.661415, 
  0.732236}, {0.661104, 0.732559}, {0.660946, 0.732723}, {0.660781, 
  0.732894}, {0.66058, 0.733315}, {0.660503, 0.733516}, {0.659971, 
  0.734326}, {0.659875, 0.734473}, {0.659855, 0.734504}, {0.659824, 
  0.73459}, {0.659524, 0.73543}, {0.659284, 0.735847}, {0.658972, 
  0.737239}, {0.658946, 0.737344}, {0.65893, 0.737407}, {0.658959, 
  0.738209}, {0.658962, 0.738301}, {0.658966, 0.738399}, {0.65898, 
  0.738813}}

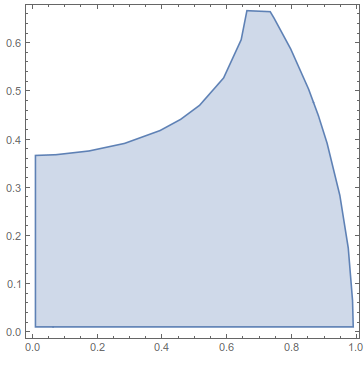
So at the end I want to define a region similar to this 

\[ScriptCapitalR] = 
 ImplicitRegion[
  Sqrt[x^2 + y^2] radius <= 0.99 radius && (y - 0.365) < 0.4 x, {{x, 
    0.01, 0.99}, {y, 0.01, 0.99}}]

where I used for simplicity the curve y = 0.4x+0.365, while I need the boundary defined by curve described by the aforementioned datapoints. I need it as a region over which I want to integrate a function f[x,y] later on.

Improve this question
$\endgroup$
6
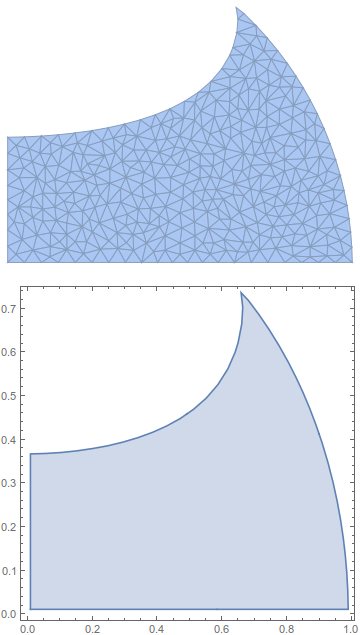
  • $\begingroup$ Why not construct an interpolating function out of your points and then use ParametricRegion[]? $\endgroup$
    – J. M.'s missing motivation ♦
    Commented Jun 9, 2015 at 13:07
  • $\begingroup$ The standard interpolation function doesn't work on such a list of data. I have tried to workaround with descriptions over here mathematica.stackexchange.com/questions/47332/… but I am stuck. $\endgroup$
    – Vaclav
    Commented Jun 9, 2015 at 13:27
  • $\begingroup$ How did you obtain the list of data points you have? I.e. where do they come from? $\endgroup$
    – MarcoB
    Commented Jun 9, 2015 at 17:43
  • $\begingroup$ Briefly I extracted that from a contour plot of a function f[x,y] and it in principal represents the boundary where the value of the function is zero. In other words it is a zero value contour and afterwords I want to integrate the f[x,y] over the region where the function is nonnegative. Just a small notice that the function I speak about is complicated and contains summations with 1000 terms with legendre polynomials. But maybe there is another way to do that. I just thought that if I find the region over which I want to integrate it will be easier but maybe I am wrong. $\endgroup$
    – Vaclav
    Commented Jun 10, 2015 at 10:26
  • $\begingroup$ So have you tried the straightforward way: NIntegrate[ f[x, y] Boole[f[x, y] < 0], {x, 0.01, 0.99}, {y, 0.01, 0.99}] ? $\endgroup$
    – BlacKow
    Commented Jun 10, 2015 at 15:28

1 Answer 1

Reset to default
2
$\begingroup$

One big obstacle with your data is that it can't be defined as a single-valued function of x - it curves backward at the end. If I take your data and define it as pts, then we can see what we are going for here

Show[
 RegionPlot[
  ImplicitRegion[
   Sqrt[x^2 + y^2] == 0.99, {{x, 0.01, 0.99}, {y, 0.01, 0.99}}]],
 Graphics@Line@pts]

Mathematica graphics

The trick I'll use is to take y as the independent variable, and so we can make a single-valued function from it. Then we can use ImplicitRegion and RegionPlot

RegionPlot[
 ImplicitRegion[
  Sqrt[x^2 + y^2] <= 0.99 && 
   x >= curve[y], {{x, 0.01, 0.99}, {y, 0.01, 0.99}}]]

enter image description here

and be once-again totally dissappointed with the combination of these two functions. But there is hope, 

DiscretizeRegion[
 ImplicitRegion[
  Sqrt[x^2 + y^2] <= 0.99 && 
   x >= curve[y], {{x, 0.01, 0.99}, {y, 0.01, 0.99}}], 
 MaxCellMeasure -> .001]
RegionPlot@%

Mathematica graphics

which is the sought-after region.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.