3D plot of two spheres with constraint

Question

I intended to plot two 3D volumes of two spheres, each centered around $(x_0,y_0,z_0)$ and $(x_1,y_1,z_1)$, respectively. Here the reference points must be selected as $x_0+y_0+z_0=1$ and $x_1+y_1+z_1=1$ and $x_1,x_0,y_1,y_0,z_1,z_0>0$. For example $x_0=0.5$, $y_0=0.3$, $z_0=0.2$. The radiuses of each shperes must be selected such that the shperes dont overlap.

Then in the same plot I need to apply the constraint that the points must add upto $1$, i.e. $x+y+z=1$ and all these points $(x,y,z)$ must also be positive. This constraint will give triangle surfaces (if not volumes) in the spheres.

I want to use Opacity so that I can see both the spheres as well as the surfaces inside the spheres.

Lastly, an arrow from the center point of each sphere to its boundary labeled by $\epsilon_0$ and $\epsilon_1$.

I have an unsuccesful attempt as follows.

x0 = 0.5;
y0 = 0.3;
z0 = 0.2;
eps0 = .2;
mySphere = Graphics3D[{Opacity[0.5], Sphere[{x0, y0, z0}, .2]}, PlotRange -> {{-1, 1}, {-1, 1}, {-1, 1}}];
g1 = ContourPlot3D[x + y + z == 1, {x, 0, 1}, {y, 0, 1}, {z, 0, 1}, Mesh -> None]

x1 = 0.1;
y1 = 0.3;
z1 = 0.6;
eps1 = .3;

mySphere2 = Graphics3D[{Opacity[0.5], Sphere[{x1, y1, z1}, .3]}, PlotRange -> {{-1, 1}, {-1, 1}, {-1, 1}}];
Show[mySphere, g1, mySphere2, Axes -> True, AxesOrigin -> {0, 0, 0}, PlotRange -> {{-.5, 1}, {-.5, 1}, {-.5, 1}}, ViewPoint -> {1.55, -1.94, 2}]

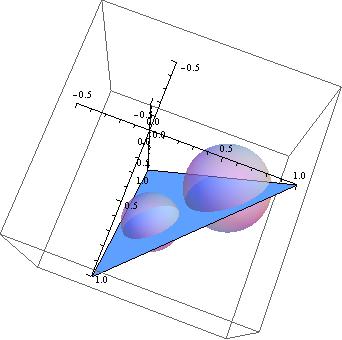
After Updating the question with the given answer. What remains is that myplane is I think correct for g1 as above (the triangle) but it must be restricted to each sphere. Namely myplane1 needs to stay in the first sphere and myplane2 needs to stay in the second sphere. There musnt be any plane outside the shperes. One last thing, if it could be possible to put an arrow?

The problem is that RegionPlot3D is very ugly. I dont wanna mesh grids on the shpere and Opacity is of no help. Not the whole trianglelike surface must be plotted. Only the part which lies in the sphere.

Can you help me to do this?

Answer 1

I think your question/request is:

Plot two non-intersecting semi-transparent spheres whose centers lie on the plane P defined by x + y + z = 1, as well as P restricted to the interiors of the spheres, all in the positive octant. Also add a labeled arrow from the center to the surface of each sphere.

If so, here's your solution:

center1 = {.5, .3, .2};
radius1 = .3;
center2 = {.1, .2, .7};
radius2 = .2;

mySphere1 = 
  Graphics3D[{Opacity[0.5], Sphere[center1, radius1]}, 
   PlotRange -> {{-.2, 1}, {-.2, 1}, {-.2, 1}}];
mySphere2 = 
  Graphics3D[{Opacity[0.5], Sphere[center2, radius2]}, 
   PlotRange -> {{-.2, 1}, {-.2, 1}, {-.2, 1}}];

myPlane = 
  Plot3D[1 - x - y, {x, 0, 1}, {y, 0, 1}, 
   RegionFunction -> 
    Function[{x, y, z}, If[x > 0 \[And] y > 0 \[And] z > 0 \[And]
       (Norm[{x, y, z} - center1] < radius1 \[Or] 
         Norm[{x, y, z} - center2] < radius2), True, False]],
   Mesh -> None,
   PlotStyle -> Opacity[0.9],
   PlotRange -> {{-.2, 1.5}, {-.2, 1.5}, {-.2, 1.5}},
   ClippingStyle -> None];

myArrow1 = Graphics3D[
   {Red, Arrow[{center1, center1 + radius1 Normalize[{1, 1, 1}]}]}];
myArrow2 = Graphics3D[
   {Red, Arrow[{center2, center2 + radius2 Normalize[{1, 1, 1}]}]}];

myLabel1 = 
  Graphics3D[
   Text[Style["\!\(\*SubscriptBox[\(\[Epsilon]\), \(1\)]\)", 16, Red],
     center1 + 1.2 radius1 Normalize[{1, 1, 1}] ]];
myLabel2 = 
  Graphics3D[
   Text[Style["\!\(\*SubscriptBox[\(\[Epsilon]\), \(2\)]\)", 16, Red],
     center2 + 1.3 radius2 Normalize[{1, 1, 1}] ]];

myfig = Show[mySphere1, mySphere2, myPlane, myArrow1, myArrow2, 
  myLabel1, myLabel2,
  Axes -> True, AxesOrigin -> {0, 0, 0}, 
  PlotRange -> {{-.2, 1}, {-.2, 1}, {-.2, 1}}, 
  ViewPoint -> {2.8, -2.8, 2}] 

Answer 2

Using

{c0, c1} = {{.5, .3, .2}, {.1, .2, .7}};
{r0, r1} = {.3, .2};
{l0, l1} = Style[Subscript["\[Epsilon]", #],Red,16] & /@ {"0", "1"};
s = {{c0, r0, l0}, {c1, r1, l1}};

(a) You can use RegionFunction in your g1 to incorporate the constraints:

g1 = ContourPlot3D[x + y + z == 1, {x, 0, 1}, {y, 0, 1}, {z, 0, 1}, 
   ContourStyle -> Directive[Opacity[.7], Orange], Mesh -> None,
   RegionFunction -> Function[{x, y, z}, Or@@(Total[({x, y, z} - #)^2] <= #2^2 & @@@ s)]];

(b) You can have all your graphics primitives in a single Graphics3D:

Graphics3D[{g1[[1]], {Opacity[.3], LightBlue, Sphere[#, #2],
   Opacity[1], Red, Arrow[{#, # + #2/Sqrt[3]}], Text[#3, 0.05 {1, 1, 1} + # + #2/Sqrt[3]]}&@@@ s},
  PlotRange -> {{-.5, 1}, {-.5, 1}, {-.5, 1}}, ViewPoint -> {1.55, -1.94, 2}]