How to generate the truth table to prove logical equivalence?

Question

How to generate the truth table to show that $p \implies (q \vee r)$ is equivalent to $(p \wedge \neg q ) \implies r$ ? Can I use BooleanTable?

Answer 1

(from the comments of belisarius & Daniel Lichtblau)

Equal[
  BooleanTable[Implies[p, q || r], {p, q, r}],
  BooleanTable[Implies[p && Not[q], r], {p, q, r}]
]

(* ==> True *)

Or without truth tables:

Reduce[Equivalent[Implies[p, q || r], Implies[p && Not[q], r]]]

(* ==> True *)

Answer 2

Here is a Rube Goldberg Machine that handles sequences of formulae.

truthTableFormattor[rawData_] := Insert[Insert[
Grid[rawData /. {0 -> 0, 
   1 -> Item[1, Background -> Lighter[Magenta]]}, 
 FrameStyle -> Gray, 
 Frame -> All], {Background -> {None, {GrayLevel[0.7], {White}}}, 
 Dividers -> {Black, {2 -> Black}}, Frame -> True, 
 Spacings -> {2, {2, {0.7}, 2}}}, 2], {Dividers -> All, 
Spacings -> .7 {1, 1}}, 2];


truthTable[f__] :=  Module[{}, atoms = Cases[Most[{f}],
 (a_ /;Length[a] == 0 \[And] Not[StringQ[a]])];heads = 
 ToString[TraditionalForm@#] & /@ {f};
  rawData = Transpose@Boole[BooleanTable[#, atoms] & /@ {f}];
  If[Last[{f}] === 1, 
   Transpose@Boole[BooleanTable[#, atoms] & /@ Most[{f}]], 
    If[Last[{f}] === "rev", 
    truthTableFormattor[{ToString[
       TraditionalForm@#] & /@ (Most@{f})}~Join~
      Transpose[(Reverse /@ 
      Boole[BooleanTable[#, atoms] & /@ (Most@{f})])]], 
     truthTableFormattor[{heads}~Join~rawData]]]];


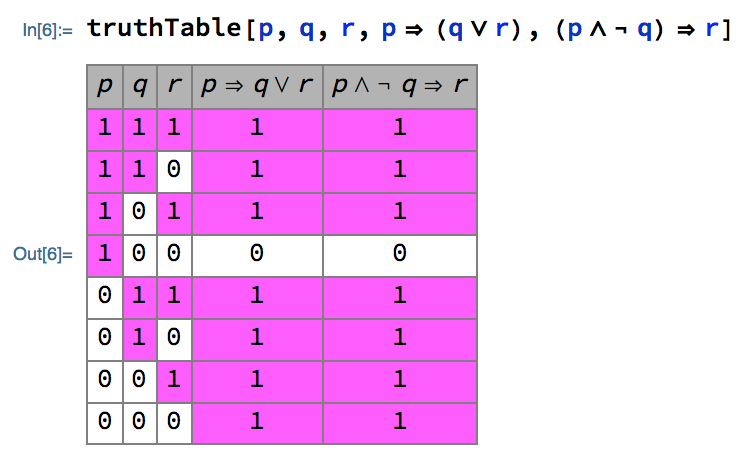
truthTable[p, q, r, p \[Implies] (q \[Or] r), (p \[And] \[Not] q) \[Implies] r]