Ellipse counting from image

Question

I have hundreds of images similar to this image:

I'm looking to count the number of ellipses as well as their major and minor diameters. The ellipses are touching, hence,

img = Import["https://i.sstatic.net/lkToU.png"]
EdgeDetect[img]

doesn't return fillable ellipses so using something like

WatershedComponents[%]

returns useless information.

I'm very new to this type of image analysis using Mathematica so any help would be greatly appreciated.

Answer 1

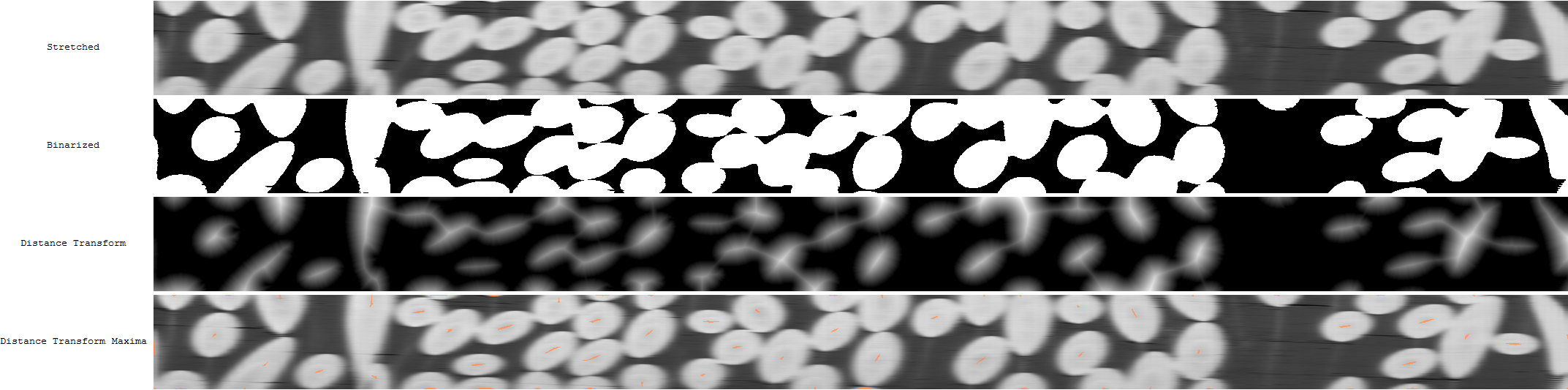
One standard way to detect circular shapes is to binarize the image and apply a distance transform: The maxima locations of the distance transform are the centers of the circles.

To make this work on your ellipses, I first have to stretch them to be (roughly) circular, as @Rahul Narain suggested in a comment:

img = ColorConvert[Import["http://i.imgur.com/oNrJq0j.png"], 
  "Grayscale"]
{w, h} = ImageDimensions[img];
ir = ImageResize[img, {w/5, h*5}]
stretched = ImageRotate[ImageResize[img, {w/5, h*5}], 90 \[Degree]]

(The rotation is just for display. If I don't rotate the image by 90°, this post will be very long. It has no influence on the ellipse detection.)

Calculating the distance transform and finding the maxima is easy:

distTransform = DistanceTransform[Binarize[stretched]];    
maxima = MaxDetect[distTransform, 2];

Here's a display of the result so far:

Grid[
 Transpose[{
   {"Stretched", "Binarized", "Distance Transform", 
    "Distance Transform Maxima"},
   Image[#, ImageSize -> All] & /@ {
     stretched,
     Binarize[stretched],
     ImageAdjust[distTransform],
     HighlightImage[stretched, maxima]}
   }]]

Now I can use ComponentMeasuements to find connected maxima locations, their orientation, shape and the max. value in the distance transform image:

components = 
  ComponentMeasurements[{MorphologicalComponents[maxima], 
    distTransform}, {"Centroid", "SemiAxes", "Orientation", "Max"}];

Show[stretched, Graphics[
  {
   Red,
   components[[All, 2]] /.
    {
     {centroid_, semiAxes_, orientation_, maxR_} :> 
      Rotate[Circle[
        centroid, {maxR*Sqrt[semiAxes[[1]]/semiAxes[[2]]], maxR}], 
       orientation, centroid]
     }
   }]]

As you can see:

• the centroids are quite good, except for the ellipses near the border, because the border changes the distance transform
• the minor radius of the ellipses is ok (it's just the distance from the center to the nearest background point)
• the estimated orientation is ok,
• but the semi-axis length ratio of the maximum is only a rough estimate.
• You could probably apply smoothing before binarizing, use MorphologicalBinarize and hand-tune the filter/binarization parameters to improve the result

ADD:

I can improve the shape estimate a bit further using WatershedComponents, with the distance transform maxima as seed points:

watershedComponents = 
  WatershedComponents[ColorNegate[distTransform], maxima] *       
    ImageData[Binarize[stretched]];
Colorize[watershedComponents]

Estimated Ellipses:

components = 
  ComponentMeasurements[
   watershedComponents, {"Centroid", "SemiAxes", "Orientation"}];

Show[stretched, Graphics[
  {
   Red,
   components /.
    {
     (n_ -> {centroid_, semiAxes_, orientation_}) :>
      {
       Rotate[Circle[centroid, semiAxes], orientation, centroid],
       Text[n, centroid]
       }
     }
   }]]