Creating a table/Matrix during a For loop

Question

I have a For loop (with i as a counter), which calculates different values (y1, y2, y3,...) as a function of the variable x. How can I create a table/matrix which contains i lines, each line containing the variable x_i and the values (y1_i, y2_i, y3_i,...)?

Answer 1

Typically the best way to accumulate results from an arbitrary process is to use Sow and Reap.

I picked four functions of i as an example. Since there are four, I Partition at the end into subsets of four:

Reap[
  For[i = 1, i < 10, i++, Sow[i]; Sow[i^2]; Sow[i!]; Sow[N@Log@i]];
][[2, 1]] ~Partition~ 4

{{1, 1, 1, 0.},
 {2, 4, 2, 0.693147},
 {3, 9, 6, 1.09861},
 {4, 16, 24, 1.38629},
 {5, 25, 120, 1.60944},
 {6, 36, 720, 1.79176},
 {7, 49, 5040, 1.94591},
 {8, 64, 40320, 2.07944},
 {9, 81, 362880, 2.19722}}

If you can formulate your code to do a single Sow for each row it will be cleaner:

Reap[
  For[i = 1, i < 10, i++, Sow[{i, i^2, i!, N@Log@i}]];
][[2, 1]]

---

Brett Champion recommended that I show the two argument from of Sow, which groups results according to explicit tags.

Sow[e, tag]
specifies that e should be collected by the nearest enclosing Reap whose pattern matches tag.

Sow[e, {tag₁, tag₂, ...}]
specifies that e should be collected once for each pattern that matches a tag_i.

(See this answer for a powerful use of the multiple tag form.)

Here is an example using this in place of Partition in the case of separate Sow expressions per loop.

Reap[
 For[i = 1, i < 10, i++, Sow[i, i]; Sow[i^2, i]; Sow[i!, i]; Sow[N@Log@i, i]];
][[2]]

---

Also, with a few exceptions it is better to avoid For in Mathematica and use constructs such as Table, Do, Array, Map, NestWhile, FixedPointList and others. I chose to answer your direct question rather than to answer with what I think you should use instead. If you are interested in alternative ways to write your program you should post a new question to that effect with an example For loop you wish to optimize.

Answer 2

Try using Table, assuming x is an array of values you could try:

matrix = Table[{xi, y1[xi], y2[xi], ..}, {xi, x}]

Table will "do the For loop" while building matrix, in this case xi will take each value of x.

And if y functions is also an array, you can use a nested Table:

matrix = Table[{xi, Sequence@@Table[y[xi,j], {j, n}]}, {xi, x}]

Here j is the index for the second table, which will run from 1 to n.

Answer 3

Now this is the kind of question that can be answered in a million ways:

yRange = 4;
iRange = 6;

(* using Table & Map *)
Table[{Subscript[x, i], 
   Sequence @@ Map[Subscript[y, #, i] &, Range[yRange]]}, {i, 
   6}] // Column

(* using Map & Table *)
Map[Prepend[Table[Subscript[y, j, #], {j, yRange}], 
    Subscript[x, #]] &, Range[iRange]] // Column

(* using MapThread & Array *)
MapThread[
  Prepend,
  {Transpose@Array[Subscript[y, ##] &, {yRange, iRange}], 
   Array[Subscript[x, ##] &, {iRange}]}] // Column

All returning:

Answer 4

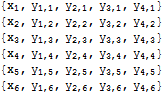
Without having your for loop in hand, you could also do something like the following:

myMatrix = {}; (* Initialize the list *)

For[i=0, i<Something, i++, 
  (* ... you do something here *)
  AppendTo[myMatrix,{xi, y1[xi], y2[xi], ..}]

  (* ... do other things .. *)
]

Or you could combine the a call to Table as @FJRA suggests for the "inner loop" j with the For loop like above.