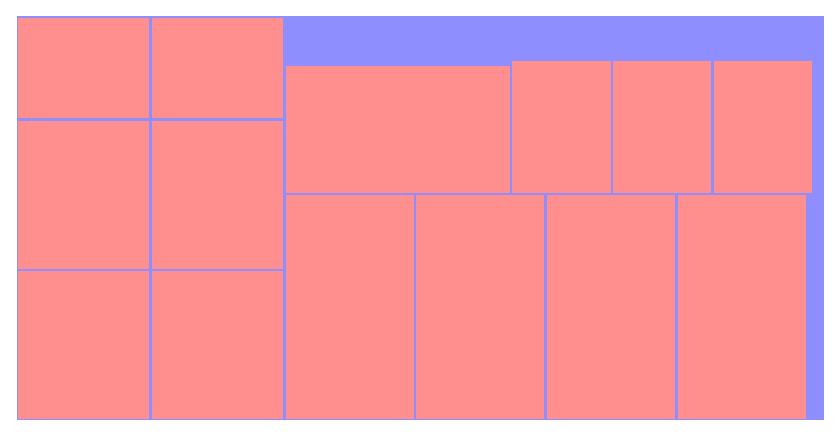
I have rectangles of a given size to fit into a larger rectangle of a fixed size, with a specified gap between the packed rectangles. I would like to write some code that optimises the number of rectangles of each given size that I can pack.
For example, say I have a large rectangle of $8' \times 4'\ \text{(}\approx 2438 \times 1219 \text{ mm)}$, I would like to know the optimal placement and orientation of, say $x\ \text{(}400 \times 450 \text{ mm)}$ rectangles , $y\ \text{(}300 \times 400 \text{ mm)}$ rectangles and $z\ \text{(}680 \times 390 \text{ mm)}$ rectangles.
This is for a real life application (cutting ply into smaller boards), so ideally I would like to optimise for least number of cuts as they charge for each cut).
$eg$
(code standard manual Graphics manual placement:
Module[{s1, s2, s3}, s1 = {400, 450}; s2 = {300, 400}; s3 = {680, 390};
Graphics[{ EdgeForm[{Nest[Lighter, Blue, 2], Thin}], Nest[Lighter, Blue, 2],
Rectangle[{0, 0}, {#, #2}] & @@ {2438, 1219},
Nest[Lighter, Red, 2],
Rectangle[{0, 0}, {s1[[1]], s1[[2]]}],
Rectangle[{0, s1[[2]] + 5}, {s1[[1]], 2 s1[[2]] + 5}],
Rectangle[{s1[[1]] + 5, 0}, {2 s1[[1]] + 5, s1[[2]]}],
Rectangle[{s1[[1]] + 5, s1[[2]] + 5}, {2 s1[[1]] + 5,
2 s1[[2]] + 5}],
Rectangle[{s2[[2]] + 5, 2 s1[[2]] + 10}, {5 + 2 s2[[2]],
2 s1[[2]] + 15 + s2[[1]]}],
Rectangle[{0, 2 s1[[2]] + 10}, {s2[[2]], 2 s1[[2]] + 15 + s2[[1]]}],
Rectangle[{2 s1[[1]] + 10, 0}, {2 s1[[1]] + 10 + s3[[2]], s3[[1]]}],
Rectangle[{2 s1[[1]] + s3[[2]] + 15,
0}, {2 s1[[1]] + 15 + 2 s3[[2]], s3[[1]]}],
Rectangle[{2 s1[[1]] + 2 s3[[2]] + 20,
0}, {2 s1[[1]] + 20 + 3 s3[[2]], s3[[1]]}],
Rectangle[{2 s1[[1]] + 3 s3[[2]] + 25,
0}, {2 s1[[1]] + 25 + 4 s3[[2]], s3[[1]]}],
Rectangle[{2 s1[[1]] + 10, s3[[1]] + 5}, {2 s1[[1]] + 10 + s3[[1]],
s3[[2]] + s3[[1]]}],
Rectangle[{2 s1[[1]] + 15 + s3[[1]],
s3[[1]] + 5}, {2 s1[[1]] + 15 + s3[[1]] + s2[[1]],
s3[[1]] + 5 + s2[[2]]}],
Rectangle[{2 s1[[1]] + 20 + s3[[1]] + s2[[1]],
s3[[1]] + 5}, {2 s1[[1]] + 20 + s3[[1]] + 2 s2[[1]],
s3[[1]] + 5 + s2[[2]]}],
Rectangle[{2 s1[[1]] + 25 + s3[[1]] + 2 s2[[1]],
s3[[1]] + 5}, {2 s1[[1]] + 25 + s3[[1]] + 3 s2[[1]],
s3[[1]] + 5 + s2[[2]]}] }]]
Have searched for rectangle packing algorithm, but can't find any with these constraints.
Added
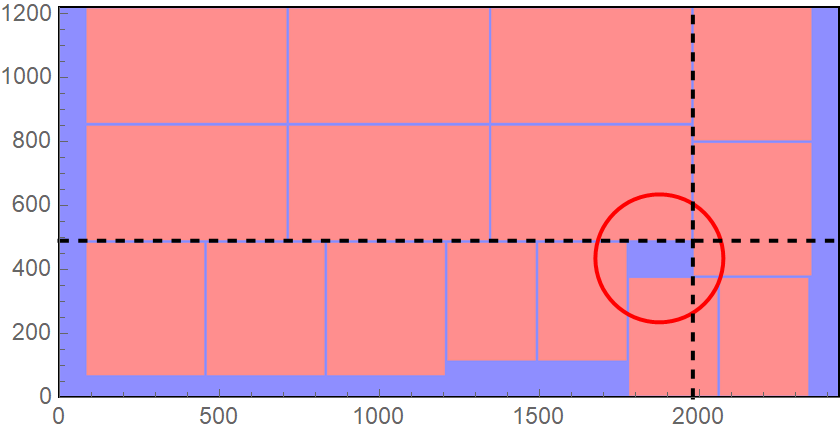
Using @GeorgeVarnavides ' code below, (though the Fitting option presents problems as outlined in comments below his answer), the method will also present practical problems as highlighted here
images = ConstantImage[Nest[Lighter, Red, 2], #] & /@
Join[ConstantArray[#, 5], ConstantArray[#2, 4],
ConstantArray[#3, 6]] & @@ {{400, 450}, {300, 400}, {680, 390}};
Show[ImageCollage[images, Automatic, {2438, 1219},
Method -> "ClosestPacking", Background -> Nest[Lighter, Blue, 2], ImagePadding -> 5],
Graphics[{Opacity[0], EdgeForm[Black], Rectangle[{0, 0}, {2438, 1219}], EdgeForm[{Red, Thick}],
Disk[{1875, 435}, 200],
Opacity[1], Thick, Dashed, Line[{{#, 0}, {#, 1219}} &[1980]],
Line[{{0, #}, {2438, #}} &[490]]}], Axes -> True]
as the guys at the timber yard will cut along the dashed lines since they can't cut part way accurately on a table saw. So noting @Syed 's comment, either strip-packing or bin-packing looks like the way to go. No idea how to implement this in MMA though.
Another issue is that the "ClosestPacking" method doesn't rotate automatically to optimise packing.
Having said that, @GeorgeVarnavides ' answer does give me a great starting point to then adjust manually (+1).