I just simulate a system and use an Extended Kalman Filter to estimate the state. Everything works, but I don't understand why I need to execute the Ndsolve command two times before it can run without error.
Here is the code (maybe it can seem to be intricate but there is no need to understand it, however, is just a simulation of a dynamical system and a filter):
t0 = 0;
tfin = 200;
SeedRandom[1234];
pstl = {xstl, ystl};
R0l[\[Theta]_] = {{Cos[\[Theta]], -Sin[\[Theta]]} , {Sin[\[Theta]],
Cos[\[Theta]]}};
pmk1 = {xmk1, ymk1};
pmk2 = {xmk2, ymk2};
numericalvalues =
Thread[{xstl, ystl, xmk1, ymk1, xmk2, ymk2} -> { 3, -2, 32, 16, -15,
2}];
(*Configuration variables*)
qalist = {x, y, \[Theta], xstls, ystls};
qa[t_] = (ToString[#] <> "[t]") & /@ qalist // ToExpression;
qad[t_] = D[qa[t], t];
qadd[t_] = D[qad[t], t];
input[t_] = {v[t], \[Omega][t]};
output[t_] = {y1[t], y2[t], yn1[t], yn2[t]};
(*Desired Trajectory*)
dgl[t_] = {-15 Cos[t] + 4, 15 Sin[t] + 4};
Rgl[t_] = {{Cos[-t], -Sin[-t]}, {Sin[-t], Cos[-t]}};
pl[t_] = {Sin[7 t], 0};
tr = dgl[t/2] + Rgl[t/2].pl[t/2];
vdes = D[tr, t];
ades = D[vdes, t];
(*System Dynamics*)
g1 = {Cos[#], Sin[#], 0, 0, 0} & @(#[[3]]) &;
g2 = {0, 0, 1, 0, 0};
dyn = (g1@#*v[t] + g2*\[Omega][t]) &;
(*Output functions*)
pstf = ({#1, #2} + R0l[#3 ].{#4, #5}) &;
h1 = (Sqrt[#.#] &[pmk1 - pstf @@ #]) &;
h2 = (Sqrt[#.#] &[pmk2 - pstf @@ # ]) &;
(*Just create white noise continuous time sample*)
CreateWhiteNoise[\[Mu]_, s_, t0_, tfin_, dt_, p_] :=
Module[{distr, n = Length[\[Mu]], listt, nc, listrn},
listt = Range[t0, tfin, dt];
nc = Length[listt];
distr = MultinormalDistribution[\[Mu], s];
listrn = RandomVariate[distr, nc];
(Interpolation[Thread[Join[{listt, listrn[[All, #]]}]]][p]) & /@
Range@n
]
(*Noise*)
cov = {{0.008, 0}, {0, 0.008}};
media = {0, 0};
noise = CreateWhiteNoise[media, cov, t0, tfin + 0.1, 0.2, t ];
(*Output functins with additive noise*)
h = {h1@#, h2@#} & ;
hn = (h@# + noise) &;
output[t_] = Join[h@qa[t], hn@qa[t]] /. numericalvalues;
(*Controller*)
pos = qa[t][[1 ;; 2]];
vel = qad[t][[1 ;; 2]];
acc = qadd[t][[1 ;; 2]];
Kv = 3*IdentityMatrix[2];
Kp = 3*IdentityMatrix[2];
ev = vdes - vel;
ep = tr - pos;
\[Nu] = ades + Kv.ev + Kp.ep;
monitor = {qa[t], input[t], output[t]};
v[t_] = Sqrt[x'[t]^2 + y'[t]^2];
\[Omega][t_] = (y''[t] * x'[t] - x''[t]*y'[t]) / (x'[t]^2 + y'[t]^2);
{y1[t_], y2[t_], yn1[t_], yn[t_]} =
Join[h@qa[t], hn@qa[t]] /. numericalvalues;
eqcontrollo = acc == \[Nu] ;
\[Theta]d = D[ ArcTan[x'[t], y'[t]], t];
eqtheta = \[Theta]'[t] == \[Theta]d;
eqsens = {xstls'[t], ystls'[t]} == {0, 0};
(*Dynamic Equations*)
eqns = {eqcontrollo, qad[t][[3 ;; 5]] == {\[Theta]d, 0, 0}} /.
numericalvalues;
(*Initial conditions*)
qa0 = {-2, 1, \[Pi]/3, 3, -2};
eqin = {x[0], y[0], \[Theta][0], xstls[0], ystls[0],
Derivative[1][x][0], Derivative[1][y][0]} == {-2, 1, \[Pi]/3,
3, -2, 1/20, Sqrt[3]/20};
{stato, ingressi, uscite} =
NDSolveValue[{eqns, eqin}, monitor, {t, t0, tfin}];
ParametricPlot[stato[[1 ;; 2]], {t, t0, tfin}, ImageSize -> Tiny];
(*FILTER*)
(*Auxiliary functions*)
ListForm[mat_] :=
DeleteCases[(Thread@# & /@ Thread@mat // Flatten ), True]
SymRed[mat_] := Module[{i, j, temp},
temp = Thread@# & /@ Thread@mat;
Normal@
SparseArray[{{i_, j_} /; i >= j :>
temp[[i, j]], {i_, j_} /; i < j :> True}, Dimensions@temp] //
ListForm
]
qlisthat = (ToString@# <> "hat" // ToExpression) & /@ qalist ;
qahat[t_] = (ToString@# <> "[t]" // ToExpression) & /@ qlisthat;
qahatd[t_] = D[qahat[t], t];
nstate = Length@qlisthat;
Pmat = Normal@
SparseArray[{{i_, j_} /; i >= j :>
ToExpression["p" <> ToString@i <> ToString@j],
{i_, j_} /; i < j :>
ToExpression["p" <> ToString@j <> ToString@i ]},
nstate*{1, 1}];
P[t_] = Array[(ToString@Pmat[[#1, #2]] <> "[t]") &,
Dimensions[Pmat]] // ToExpression;
Pd[t_] = D[P[t], t];
P0 = IdentityMatrix[nstate]*{0.005, 0.005, 0.3 Degree, 0.005,
0.005 } // Chop;
stima0 = RandomVariate[MultinormalDistribution[ qa0, P0]];
initstima = qahat[0] == stima0;
initcov = P[0] == P0 ;
Rinv = Inverse[cov] // Chop;
predoutput = h@qahat[t] /. numericalvalues;
errstima = qa[t] - qahat[t];
A = D[dyn@qahat[t], {qahat[t]}];
Ci = D[h@qahat[t], {qahat[t]}] /. numericalvalues;
K = P[t].Ci\[Transpose].Rinv;
errpred = hn@qa[t] - predoutput /. numericalvalues;
initEKF = {initstima, initcov};
eqStima = qahatd[t] == dyn@qahat[t] + K.errpred;
eqP = SymRed[
Pd[t] ==
A.P[t] + P[t].A\[Transpose] - P[t].Ci\[Transpose].Rinv.Ci.P[t]];
eqEKF = {eqStima, eqP} /. numericalvalues;
last part of code:
errstimaEKF =
NDSolveValue[{eqns, eqin, eqEKF, initEKF}, errstima, {t, t0, tfin},
WorkingPrecision -> 12, MaxStepSize -> 0.001, MaxSteps -> 10^6];
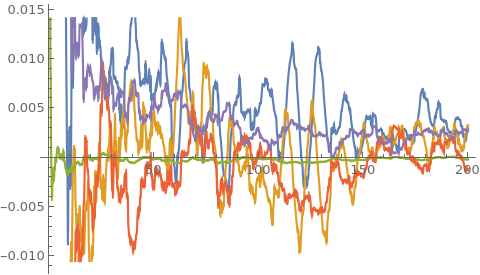
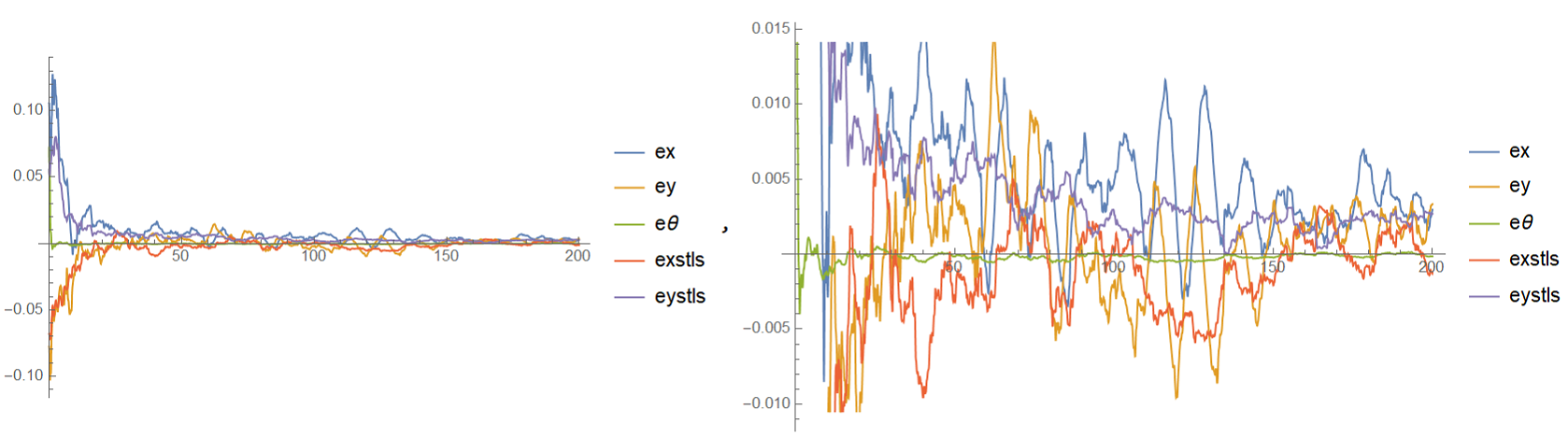
Plot[errstimaEKF, {t, t0, tfin},
PlotLegends -> ("e" <> ToString@# & /@ qalist), PlotRange -> All]
This should be the result:
It happens only if I specify MaxStepSize option in Ndsolve or if tfin is high.
QUESTION 2
The code is terribly slow,someone knows if is there a way to manipulate the Ndsolve input (ODE) to speed up the code ?
Let's say for example using := instead of = or using functions instead of expressions, maybe with the pattern test _?NumericQ in the arguments.
In the code above is there a way to manipulate the ODE in a way that they contain functions evaluated only numerically instead of expressions? Maybe using something the numeric pattern test only on the time variable t