Extrude a parametric plot along a straight line obtainning a closed surface

Question

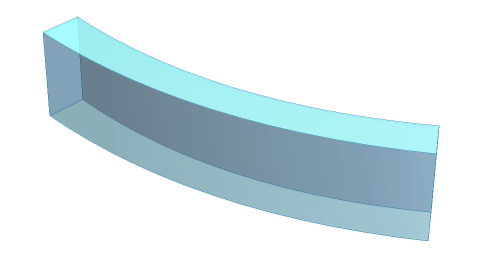
I made this parametric plot

ParametricPlot[
 {x1 - 0.035 x1 x2, 0.0175 x1^2 + (1. + 0.0175 x2) x2}, {x1, -b2, b2}, {x2, -a2, a2},
 Frame -> False,
 Axes -> False,
 Mesh -> None,
 BoundaryStyle -> {{RGBColor["#2980b9"], 
    Opacity[0.9]}, {RGBColor["#2980b9"]}},
 PlotStyle -> {{RGBColor["#2980b9"], 
    Opacity[0.4]}, {RGBColor["#2980b9"], Opacity[0.8]}},
 PlotRange -> All
 ]

and this is the output

The thing that I wanna do now is to "extrude" this surface along a straight line, have some shadow depth if it is possible, and keep the same color of the 2D surface for the 3D external surface which has to be closed on both ends.

I already found something here (Extruding along a path) but I am not able to change the colours and I was hoping that, since I want to extrude my surface along a line, perhaps there was a simpler method.

I thank everyone for the answers in advance.

Answer 1

To get the mesh you can extract the shape from plot and discretize it, then use RegionProduct to extrude along a 1D line. Note that there's a bug in RegionProduct where for meshes the line has to have machine precision numbers for the line coordinates. There is no easy way to do shadows unfortunately, so you'll have to make do with bog-standard diffuse lighting.

{a2, b2} = {1, 10};
plot = ParametricPlot[{x1 - 0.035 x1 x2, 
   0.0175 x1^2 + (1. + 0.0175 x2) x2}, {x1, -b2, b2}, {x2, -a2, a2}, 
  Frame -> False, Axes -> False, Mesh -> None, 
  BoundaryStyle -> {{RGBColor["#2980b9"], 
     Opacity[0.9]}, {RGBColor["#2980b9"]}}, 
  PlotStyle -> {{RGBColor["#2980b9"], 
     Opacity[0.4]}, {RGBColor["#2980b9"], Opacity[0.8]}}, 
  PlotRange -> All
  ]
mesh = DiscretizeGraphics@plot;
(* you need the N[...] because region code is a cruel joke *)
reg = RegionProduct[mesh, Line[{{0}, {N[5]}}]];
Graphics3D[{EdgeForm[None], Lighter[RGBColor["#2980b9"]], reg}, 
 Lighting -> "Neutral", Boxed -> False]

But if you need more fancy effects, you can always Export["mesh.obj", mesh] and throw it into Blender or any 3D software of your choosing:

Answer 2

Clear["`*"];
f[x1_, x2_, t_] = {x1 - 0.035 x1 x2, 0.0175 x1^2 + (1. + 0.0175 x2) x2, 0} + 
   t {0, 0, 1};
{c1, c2} = {10, 1};
h = 5;
SetOptions[ParametricPlot3D, Axes -> False, Mesh -> None, 
  BoundaryStyle -> {{RGBColor["#2980b9"], 
     Opacity[0.9]}, {RGBColor["#2980b9"]}}, 
  PlotStyle -> {{RGBColor["#2980b9"], 
     Opacity[0.4]}, {RGBColor["#2980b9"], Opacity[0.8]}}, 
  PlotRange -> All];
Show[ParametricPlot3D[{f[x1, x2, 0], f[x1, x2, h]}, {x1, -c1, 
   c1}, {x2, -c2, c2}], 
 ParametricPlot3D[f[x1, c2, t], {x1, -c1, c1}, {t, 0, h}], 
 ParametricPlot3D[f[x1, -c2, t], {x1, -c1, c1}, {t, 0, h}], 
 ParametricPlot3D[f[c1, x2, t], {x2, -c2, c2}, {t, 0, h}], 
 ParametricPlot3D[f[-c1, x2, t], {x2, -c2, c2}, {t, 0, h}]]