I was trying to verify my hand solution for this PDE using Mathematica.
Is a trick to help Mathematica obtain solution to $u_{t}+u=u_{xx}$ with initial conditions $u(x,0)=f(x)$ and boundary conditions $u(0,t)=u(L,t)=0$ ?
I tried changing $L$ to $1$, and tried using explicit $f(x)$, but Mathematica still can't solve it. This is a basic separation of variables PDE which I think Mathematica 11.2 should have been able to solve it. So I thought may be some one can have a trick to help Mathematica with it.
Hand solution
Using separation of variables, let $u\left( x,t\right) =X\left( x\right) T\left( t\right) $. Substituting this back into the PDE gives \begin{align*} T^{\prime}X+TX & =X^{\prime\prime}T\\ \frac{T^{\prime}}{T}+1 & =\frac{X^{\prime\prime}}{X}=-\lambda \end{align*} Where the separation constant is some real value $-\lambda$. This gives the following two ODE's to solve \begin{align} T^{\prime}+(1+\lambda)T & =0\tag{1}\\ X^{\prime\prime}+\lambda X & =0\tag{2} \end{align} Starting with the spatial ODE in order to obtain the eigenvalues. The boundary conditions on the spatial ODE become \begin{align*} X\left( 0\right) & =0\\ X\left( 1\right) & =0 \end{align*}
The above boundary value ODE is standard one and its eigenvalues are $$ \lambda_{n}=\left( \frac{n\pi}{L}\right) ^{2}\qquad n=1,2,3,\cdots $$ The corresponding eigenfunctions are $$ X_{n}\left( x\right) =c_{n}\sin\left( \sqrt{\lambda_{n}}x\right) $$
The solution to the time ODE (1) is, using integrating factor method
$$ T\left( t\right) =e^{-\left( 1+\lambda_{n}\right) t} $$
Therefore \begin{align} u\left( x,t\right) & =\sum_{n=1}^{\infty}u_{n}\nonumber\\ & =\sum_{n=1}^{\infty}T_{n}\left( t\right) X_{n}\left( x\right) \nonumber\\ & =\sum_{n=1}^{\infty}c_{n}e^{-\left( 1+\lambda_{n}\right) t}\sin\left( \sqrt{\lambda_{n}}x\right) \tag{3} \end{align}
In order to determine $c_{n}$, the initial condition is now applied. At $t=0$, $u\left( x,0\right) =f\left( x\right) $ and the above becomes $$ f\left( x\right) =\sum_{n=1}^{\infty}c_{n}\sin\left( \sqrt{\lambda_{n} }x\right) $$ Multiplying both sides by $\sin\left( \sqrt{\lambda_{m}}x\right) $ and integrating over the domain of $f\left( x\right) $ gives $$ \int_{0}^{L}f\left( x\right) \sin\left( \sqrt{\lambda_{m}}x\right) dx=\int_{0}^{L}\sum_{n=1}^{\infty}c_{n}\sin\left( \sqrt{\lambda_{n}}x\right) \sin\left( \sqrt{\lambda_{m}}x\right) dx $$ Interchanging the order of summation and integrating gives $$ \int_{0}^{L}f\left( x\right) \sin\left( \sqrt{\lambda_{m}}x\right) dx=\sum_{n=1}^{\infty}c_{n}\int_{0}^{L}\sin\left( \sqrt{\lambda_{n}}x\right) \sin\left( \sqrt{\lambda_{m}}x\right) dx $$ By orthogonality of $sin$ functions, all terms in the right side vanish except when $n=m$, leading to \begin{align*} \int_{0}^{L}f\left( x\right) \sin\left( \sqrt{\lambda_{m}}x\right) dx & =c_{m}\int_{0}^{L}\sin^{2}\left( \sqrt{\lambda_{m}}x\right) dx\\ & =c_{m}\frac{L}{2} \end{align*} Therefore $$ c_{m}=\frac{2}{L}\int_{0}^{L}f\left( x\right) \sin\left( \sqrt{\lambda_{m} }x\right) dx $$ Since $m$ is an arbitrary number, replacing it back to $n$ \begin{equation} c_{n}=\frac{2}{L}\int_{0}^{L}f\left( x\right) \sin\left( \sqrt{\lambda_{n} }x\right) dx\qquad n=1,2,3,\cdots\tag{4} \end{equation}
But $\sqrt{\lambda_{n}}=\frac{n\pi}{L}$, therefore $$ c_{n}=\frac{2}{L}\int_{0}^{L}f\left( x\right) \sin\left( \frac{n\pi} {L}x\right) dx\qquad n=1,2,3,\cdots $$
The above shows that $c_{n}$ is the Fourier sine series of $f\left( x\right) $. Since $f\left( x\right) $ is not given, explicit solution for $c_{n}$ can not be found. Therefore the final solution is
\begin{align*} u\left( x,t\right) & =\sum_{n=1}^{\infty}c_{n}e^{-\left( 1+\lambda _{n}\right) t}\sin\left( \sqrt{\lambda_{n}}x\right) \\ & =\sum_{n=1}^{\infty}\left( \frac{2}{L}\int_{0}^{L}f\left( x\right) \sin\left( \sqrt{\lambda_{n}}x\right) dx\right) e^{-\left( 1+\lambda _{n}\right) t}\sin\left( \sqrt{\lambda_{n}}x\right) \end{align*}
With $\lambda_{n}=\left( \frac{n\pi}{L}\right) ^{2}$.
Mathematica attempts
ClearAll[x, t, u, f];
pde = D[u[x, t], t] + u[x, t] == D[u[x, t], {x, 2}];
ic = u[x, 0] == f[x];
bc = {u[0, t] == 0, u[L, t] == 0};
DSolve[{pde, ic, bc}, u[x, t], x, t]

ClearAll[x, t, u, f];
pde = D[u[x, t], t] + u[x, t] == D[u[x, t], {x, 2}];
ic = u[x, 0] == x (1 - x);
bc = {u[0, t] == 0, u[L, t] == 0};
DSolve[{pde, ic, bc}, u[x, t], x, t]
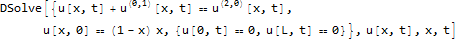
ClearAll[x, t, u, f];
pde = D[u[x, t], t] + u[x, t] == D[u[x, t], {x, 2}];
ic = u[x, 0] == x (1 - x);
bc = {u[0, t] == 0, u[1, t] == 0};
DSolve[{pde, ic, bc}, u[x, t], x, t]
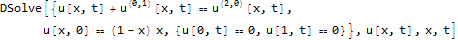
Maple 2017.3 is able to solve this
pde:=diff(u(x,t),t)+u(x,t)=diff(u(x,t),x$2);
ic:=u(x,0)=f(x);
bc:=u(0,t)=0,u(L,t)=0;
pdsolve({pde,ic,bc},u(x,t)) assuming L>0;
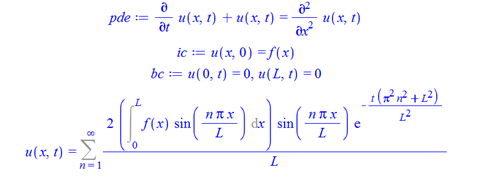
May be next version of Mathematica can solve this?

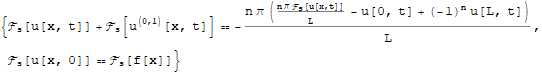
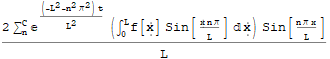
thiswhen you say "this only works"? $\endgroup$