I'm new to Mathematica (installed couple of hours ago) and I need to compute a few Lie brackets between two vector fields $f$ and $g$. $$ f\left(\mathbf{x}\right) = \left( \begin{array}{c} x_3\\ x_4\\ \frac{-2m_2x_2x_3x_4 -g\cos\!\left(x_1\right)\left(m_2x_2 + m_1r\right)}{m_2{x_2}^2 + m_1r^2 + J_1 + J_2}\\ x_2{x_3}^2 - g\sin\!\left(x_1\right)\\ \end{array} \right)$$ $$g\left(\mathbf{x}\right) = \left( \begin{array}{c} 0\\ 0\\ \frac{1}{m_2{x_2}^2 + m_1r^2 + J_1 + J_2}\\ 0\\ \end{array} \right) $$ Where the vector for variables is $\mathbf{x}=\left(x_1,x_2,x_3,x_4\right)^T$. To compute the Lie bracket of $f$ along $g$ I need to do the following: $$\left[f,g\right] = \frac{\partial g}{\partial x} f - \frac{\partial f}{\partial x} g $$ And to do that I need the Jacobian of $f$ and $g$ which seems I can't do in Mathematica. I used the following code for $f$:
f = {{x3}, {x4}, {-(2 m2 x2 x3 x4 + g Cos[x1] (m2 x2 + m1 r))/ (m2 x2^2 + m1 r^2 + J1 + J2)}, {x2 x3^2 - g Sin[x1]}}
x = {{x1}, {x2}, {x3}, {x4}}
jf = D[f, {x}]
jf // MatrixForm
This code while it gives the numerical values somehow correct, it kinda mess up the dimensions of the results. It should be a $4 \times 4$ matrix, but it gives me a $4 \times 1 \times 4 \times 1$ tensor. What am I doing wrong here ?
The output of jf:
{{{{0}, {0}, {1}, {0}}}, {{{0}, {0}, {0}, {1}}}, {{{(g (m1 r + m2 x2) Sin[x1])/(J1 + J2 + m1 r^2 + m2 x2^2)}, {(-2 m2 x3 x4 - g m2 Cos[x1])/(J1 + J2 + m1 r^2 + m2 x2^2) - (m2 x2 (-2 m2 x2 x3 x4 - g (m1 r + m2 x2) Cos[x1]))/(J1 + J2 + m1 r^2 + m2 x2^2)^2}, {-((2 m2 x2 x4)/(J1 + J2 + m1 r^2 + m2 x2^2))}, {-((2 m2 x2 x3)/(J1 + J2 + m1 r^2 + m2 x2^2))}}}, {{{-g Cos[x1]}, {x3^2}, {2 x2 x3},0}}}}
Dimensions[jf]
{4, 1, 4, 1}

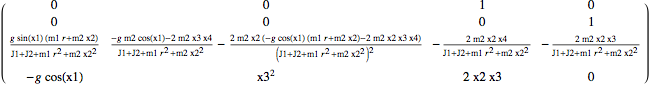
Lists are treated as general tensors. Therefore, if you don't want the extra dimensions in the output, you can feel free to omit them in the input. $\endgroup$Dimensions[{{a},{b}}]vs.Dimensions[{a,b}]. The first is interpreted as a matrix, and is transposable, the second is a vector and is not internally transposable. This is partially due to no distinctions existing between a vector and its dual in a Cartesian space. That, and internally they're just arrays of memory. $\endgroup$