DensityPlot needed

Question

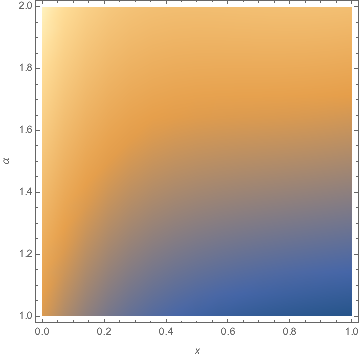
I want to make a DensityPlot showing $y(x)$ plotted against $x$ and $\alpha$, with the following differential equations

α = 1
NDSolve[{α*y'''[x] + 8 y''[x] + 17 y'[x] + 10 y[x] == 0, 
   y[0] == 6*α, y'[0] == -20, y''[0] == 84}, y, {x, 0, 1}];
Plot[Evaluate[y[x] /. %], {x, 0, 1}]

where $\alpha$ is varied between 1 and 2.

Answer 1

You want the fantastic function ParametricNDSolve:

Clear[α]

sol = ParametricNDSolve[{
   α y'''[x] + 8 y''[x] + 17 y'[x] + 10 y[x] == 0,
   y[0] == 6 α,
   y'[0] == -20,
   y''[0] == 84}, 
  y, {x, 0, 1}, {α}]

DensityPlot[Evaluate[y[α][x] /. sol], {x, 0, 1}, {α, 1, 2}, 
 FrameLabel -> {x, α}]

Answer 2

This differential equation is quite simple. So you can solve it analytically.

y[x_, α_] = FullSimplify[y[x] /. First@
DSolve[{α*y'''[x] + 8 y''[x] + 17 y'[x] + 10 y[x] == 0, 
        y[0] == 6*α, y'[0] == -20, y''[0] == 84}, y, x], 0 >= x <= 1 && 1 <= α <= 2]

The is result not shown here.

And plot the DensityPlot

DensityPlot[y[x, α], {x, 0, 1}, {α, 1, 2}]