I have run into some weird behaviour on the part of NDSolve which I find pretty bizarre and which I would like to understand better.
Suppose, for the sake of argument, that I want to study the ODE system
$$
\left\{\begin{array}{l}
y'(x)=\cos(x)\phantom{y(x)}\\
z'(x)=y(x),\phantom{\cos(x)}
\end{array}\right.
$$
and that I want to do it sequentially. This is pretty easy: I begin by solving the first equation and setting the resulting InterpolatingFunction to a placeholder f,
f = y /. First[NDSolve[{y'[x] == Cos[x], y[0] == 0}, y, {x, 0, 20}]]
and then I use this f inside the second NDSolve,
NDSolve[{z'[x] == f[x], z[0] == 0}, z, {x, 0, 20}]
and everything is dandy.
Going a bit further down For The Sake Of Argument alley, suppose that for whatever reason I want my forcing functions and dependent variables to have vector values (with a single entry). I need to adjust the initial conditions to match, but otherwise everything works fine:
g = y /. First[NDSolve[{y'[x] == {Cos[x]}, y[0] == {0}}, y, {x, 0, 20}]]
NDSolve[{z'[x] == g[x], z[0] == {0}}, z, {x, 0, 20}]
Now finally, suppose that some devious, argumentative trickster decides to sneak in to change the g[x] and add a zero of appropriate dimensions, changing that right-hand side to {0}+g[x], so the command will look like
NDSolve[{z'[x] == {0} + g[x], z[0] == {0}}, z, {x, 0, 20}]
"That's not a problem!", I hear you say, "surely that's not something that will cause Mathematica to just suddenly throw its hands up in the air and give up, right?", and I wholeheartedly agree with you.
Unfortunately, that's not really the case. For some reason, that last bit seems to simply be too much, and Mathematica returns the whole NDSolve construct undigested:

Now this is pretty weird. Watering down the NDSolve commands to non-evaluating ndsolves throws some light into what's going on: when the arguments to the solver are processed, adding the explicit {0} to the symbolic g[x] results in the equation z'[x]=={g[x]}, where the right-hand side is one level too deep:

As such, putting in an explicit part statement on the g, or making the initial condition for z one level deeper will produce mostly acceptable results (or at least, it will produce results, but the second option gives the wrong shape). Thus
NDSolve[{z'[x] == {0} + g[x][[1]], z[0] == {0}}, z, {x, 0, 20}]
NDSolve[{z'[x] == {0} + g[x], z[0] == {{0}}}, z, {x, 0, 20}]
produces
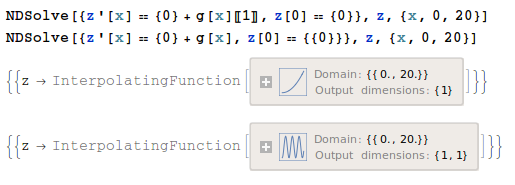
Now this is sort of acceptable (at least it sort of kind of fixes the problem) but it feels like a horrible kludge to me.
Mostly, though, I'm deeply uncomfortable with the fact that expressions which look equivalent (g[x] and {0}+g[x]) produce wildly different results, in an unstable, you-can't-know-what-you'll-get sort of way. Is there a unified way to ensure a more uniform output in this sort of situation?


{a} + b == {a + b}, wherebis yourInterpolatingFunctionobject. IfListis not theHeadofb, thenPlus, beingListablewill absorb thatbintoList[a]. I'm betting there is probably no getting around this. $\endgroup${0} + g[x]will evaluate to{g[x]}beforeNDSolveexecute. I think you'll find this post interesting: mathematica.stackexchange.com/a/97006/1871 $\endgroup$