I am processing SNe Ia data and using FindMinimum to try and extract two values: t1 and a0. The FindMinimum value works only if I supply values that are close to the answer. If I provide no starting values or arbitrary starting values (e.g. t1=1, a0=1), then the function doesn't converge. If I give it values close to the answer (e.g. t1=1*^18, a0=1*^-14), then I get an answer. Here's the code:
c = 2.99792*^5;
megaParsec = 3.08567758*^19;
alpha = 0.147;
beta = 3.13;
mag = -19.34;
chiSquared[t1_, a0_] :=
Sum[residual[i, t1, a0]^2/error[i]^2, {i, 1, Length[data]}]
error[i_] := (distance = observedDistance[i];
distanceModuli =
Log10[distance/megaParsec]*5 + 25; (data[[i, 6]]/distanceModuli)*
distance)
luminousDistance[z_, t1_, a0_] := -((a0*t1^2*z +
2*c*((-t1)*(1 + z) + Sqrt[t1^2*(1 + z)]))/(2 + z))
residual[i_, t1_, a0_] := (z = data[[i, 2]];
observedDistance[i] - luminousDistance[z, t1, a0])
observedDistance[i_] := (magnitude =
data[[i, 3]] + alpha*(data[[i, 4]] - 1) - beta*data[[i, 5]] - mag;
10^((magnitude - 25)/5)*megaParsec)
And here is the data:
data = {{"sn2004s", 0.01, 14.183, 0.973, 0.035, 0.213}, {"sn1999ac",
0.01, 14.13, 0.987, 0.056, 0.177}, {"sn1997do", 0.011, 14.317,
0.983, 0.056, 0.204}, {"sn2006bh", 0.011, 14.347, 0.814, -0.045,
0.168}, {"sn2002dp", 0.011, 14.597, 0.973, 0.113,
0.203}, {"sn2005al", 0.012, 14.843, 0.871, -0.073,
0.179}, {"sn2001ep", 0.013, 14.904, 0.903, 0.088,
0.189}, {"sn1997e", 0.014, 15.118, 0.819, 0.036,
0.2}, {"sn2001fe", 0.015, 14.685, 1.077, -0.002,
0.194}, {"sn2005bo", 0.014, 15.646, 0.867, 0.236,
0.186}, {"sn2002ha", 0.014, 14.703, 0.867, -0.056,
0.202}, {"sn2006n", 0.015, 15.09, 0.787, -0.023,
0.196}, {"sn1999dq", 0.014, 14.409, 1.103, 0.075,
0.183}, {"sn1999aa", 0.016, 14.728, 1.12, -0.052,
0.167}, {"sn1992al", 0.014, 14.499, 0.959, -0.087,
0.187}, {"sn2001bt", 0.014, 15.317, 0.899, 0.18,
0.181}, {"sn2005el", 0.015, 14.842, 0.838, -0.08,
0.19}, {"sn1999dk", 0.014, 14.881, 0.991, 0.086,
0.198}, {"sn2001v", 0.016, 14.596, 1.111, 0.025,
0.176}, {"sn2005kc", 0.015, 15.502, 0.933, 0.176,
0.192}, {"sn1994s", 0.015, 14.801, 1.031, -0.037,
0.208}, {"sn2001cz", 0.016, 15.083, 1.007, 0.071,
0.19}, {"sn2001cn", 0.015, 15.306, 0.933, 0.145,
0.191}, {"sn2001bf", 0.015, 14.719, 1.1, 0, 0.215}, {"sn2004eo",
0.015, 15.104, 0.88, 0.058, 0.18}, {"sn2004ey", 0.016, 14.676,
1.001, -0.107, 0.201}, {"sn2001en", 0.015, 15.095, 0.877, 0.038,
0.309}, {"sn2006td", 0.016, 15.735, 0.841, 0.123,
0.208}, {"sn1996bv", 0.017, 15.353, 1.064, 0.162,
0.234}, {"sn2006ax", 0.017, 14.984, 1.001, -0.091,
0.174}, {"sn2001da", 0.017, 15.464, 0.778, 0.078,
0.412}, {"sn2000dk", 0.018, 15.361, 0.768, -0.001,
0.184}, {"sn1998v", 0.017, 15.105, 0.983, 0.004,
0.243}, {"sn1998ef", 0.018, 14.832, 0.892, -0.068,
0.203}, {"sn2007ci", 0.019, 15.909, 0.729, 0.066,
0.188}, {"sn1992bo", 0.019, 15.79, 0.771, -0.03,
0.182}, {"sn2002kf", 0.02, 15.664, 0.862, -0.032,
0.222}, {"sn2005ki", 0.02, 15.536, 0.844, -0.067,
0.171}, {"sn2003w", 0.021, 15.89, 0.993, 0.134,
0.178}, {"sn1992bc", 0.021, 15.145, 1.081, -0.086,
0.173}, {"sn2006ej", 0.02, 15.779, 0.853, 0.026,
0.204}, {"sn2007bc", 0.022, 15.912, 0.852, 0.011,
0.191}, {"sn2002jy", 0.022, 15.758, 1.109, -0.008,
0.2}, {"sn2008bf", 0.022, 15.739, 1.034, 0.013,
0.178}, {"sn2006bq", 0.022, 16.191, 0.848, 0.079,
0.191}, {"sn2006et", 0.022, 16.003, 1.11, 0.166,
0.215}, {"sn2006cp", 0.023, 16.015, 1.052, 0.099,
0.188}, {"sn2006ar", 0.023, 16.486, 0.903, 0.128,
0.198}, {"sn1995ak", 0.022, 15.982, 0.85, 0.011,
0.278}, {"sn2006mp", 0.023, 16.009, 1.092, 0.036,
0.188}, {"sn2005bg", 0.025, 15.833, 1.044, -0.003,
0.185}, {"sn2006ac", 0.023, 16.193, 0.895, 0.08,
0.174}, {"sn1994m", 0.025, 16.278, 0.83, 0.043,
0.208}, {"sn2000cn", 0.024, 16.554, 0.755, 0.115,
0.189}, {"sn2007f", 0.024, 15.914, 1.059, -0.02,
0.187}, {"sn2000ca", 0.023, 15.606, 1.062, -0.073,
0.194}, {"sn2007qe", 0.024, 16.074, 1.059, 0.067,
0.171}, {"sn2006sr", 0.024, 16.157, 0.852, 0.011,
0.195}, {"sn1993h", 0.024, 16.766, 0.726, 0.179,
0.193}, {"sn2002bf", 0.025, 16.358, 0.936, 0.169,
0.232}, {"sn2002he", 0.025, 16.271, 0.82, -0.012,
0.214}, {"sn1992ag", 0.025, 16.355, 0.95, 0.18,
0.231}, {"sn2005ms", 0.027, 16.18, 1.045, -0.01,
0.181}, {"sn1992p", 0.028, 16.097, 1.079, -0.063,
0.271}, {"sn2007cq", 0.025, 15.85, 0.938, 0.003,
0.198}, {"sn2005na", 0.027, 15.934, 0.95, -0.078,
0.183}, {"sn2004gs", 0.027, 17.146, 0.768, 0.167,
0.172}, {"sn1999gp", 0.027, 16.044, 1.182, 0.029,
0.186}, {"sn2007co", 0.027, 16.491, 0.964, 0.098,
0.186}, {"sn1998ab", 0.028, 16.089, 0.982, 0.066,
0.194}, {"sn2002de", 0.028, 16.699, 1.062, 0.139,
0.223}, {"sn2003u", 0.029, 16.521, 0.791, 0.003,
0.227}, {"sn2005eq", 0.029, 16.322, 1.159, 0.026,
0.184}, {"sn2001ba", 0.03, 16.244, 1.008, -0.095,
0.196}, {"sn1996c", 0.031, 16.654, 1.073, 0.087,
0.208}, {"sn2006qo", 0.03, 16.865, 1.048, 0.192,
0.18}, {"sn2003ch", 0.03, 16.725, 0.842, -0.001, 0.2}, {"sn1990o",
0.03, 16.267, 1.047, -0.018, 0.229}, {"sn1997dg", 0.031, 16.84,
0.941, -0.024, 0.215}, {"sn2006az", 0.031, 16.517, 0.858, -0.064,
0.168}, {"sn2004as", 0.033, 17.011, 1.049, 0.077,
0.2}, {"sn2007bd", 0.032, 16.614, 0.844, -0.021,
0.18}, {"sn1999cc", 0.032, 16.783, 0.812, 0.015,
0.174}, {"sn2006s", 0.033, 16.898, 1.112, 0.074,
0.172}, {"sn2006bt", 0.031, 16.971, 1.011, 0.13,
0.181}, {"sn2004l", 0.033, 17.385, 0.925, 0.192,
0.234}, {"sn2005iq", 0.034, 16.76, 0.878, -0.085,
0.17}, {"sn2003iv", 0.035, 17.03, 0.741, -0.04,
0.25}, {"sn2006gr", 0.034, 17.009, 1.103, 0.1,
0.184}, {"sn2005eu", 0.035, 16.521, 1.101, -0.027,
0.213}, {"sn2002hd", 0.036, 16.867, 0.858, 0.081,
0.377}, {"sn1992bg", 0.035, 16.749, 0.956, -0.037,
0.281}, {"sn1996bl", 0.035, 16.677, 0.979, 0.006,
0.206}, {"sn2000cf", 0.037, 17.05, 0.916, -0.023,
0.193}, {"sn2006mo", 0.037, 17.486, 0.759, 0.065,
0.207}, {"sn2001eh", 0.037, 16.667, 1.185, -0.004,
0.198}, {"sn1999aw", 0.039, 16.791, 1.234, -0.032,
0.182}, {"sn2002hu", 0.038, 16.69, 1.045, -0.058,
0.183}, {"sn2003fa", 0.039, 16.763, 1.152, -0.01,
0.182}, {"sn2001az", 0.04, 16.986, 1.108, -0.049,
0.259}, {"sn2005lz", 0.041, 17.674, 0.844, 0.093,
0.201}, {"sn1992bl", 0.042, 17.345, 0.815, -0.035,
0.24}, {"sn1992bh", 0.042, 17.649, 0.99, 0.065,
0.215}, {"sn2004gu", 0.047, 17.439, 1.141, 0.101,
0.178}, {"sn2005hc", 0.045, 17.302, 1.078, -0.006,
0.18}, {"sn1993ag", 0.049, 17.865, 0.884, 0.085,
0.241}, {"sn1995ac", 0.049, 17.091, 1.085, -0.012,
0.184}, {"sn1990af", 0.05, 17.796, 0.741, -0.006,
0.198}, {"sn1993o", 0.053, 17.656, 0.906, -0.073,
0.196}, {"sn1999ao", 0.055, 17.906, 0.95, -0.017,
0.212}, {"sn1998dx", 0.054, 17.546, 0.844, -0.088,
0.24}, {"sn2006ob", 0.059, 18.302, 0.741, 0.022,
0.187}, {"sn2006oa", 0.058, 17.955, 1.131, 0.023,
0.196}, {"SDSS3901", 0.063, 18.015, 1.117, 0.051,
0.18}, {"sn1992bs", 0.063, 18.317, 0.966, -0.018,
0.237}, {"sn2006an", 0.065, 18.195, 1.061, 0.016,
0.222}, {"sn2007ae", 0.063, 17.832, 1.198, 0.002,
0.234}, {"SDSS10028", 0.065, 18.373, 0.891, 0.054,
0.2}, {"SDSS6057", 0.067, 18.641, 0.944, 0.129,
0.206}, {"sn2006al", 0.069, 18.485, 0.809, -0.063,
0.248}, {"sn1993b", 0.07, 18.497, 0.914, 0.057,
0.273}, {"sn2006on", 0.068, 18.494, 1.038, 0.104,
0.31}, {"sn1992ae", 0.075, 18.448, 0.944, -0.027,
0.266}, {"sn2005ir", 0.075, 18.4, 1.043, 0.014,
0.17}, {"sn1999bp", 0.078, 18.422, 1.065, -0.037,
0.198}, {"sn1992bp", 0.079, 18.335, 0.877, -0.075,
0.212}, {"sn2005ag", 0.08, 18.44, 1.029, -0.014,
0.179}, {"SDSS1241", 0.087, 19.103, 0.929, 0.072,
0.189}, {"SDSS3592", 0.087, 18.751, 0.975, -0.04,
0.178}, {"SDSS6773", 0.09, 18.663, 0.979, -0.011,
0.207}, {"SDSS2102", 0.095, 18.634, 1.133, -0.094,
0.217}, {"SDSS10434", 0.104, 19.185, 1.01, -0.053,
0.224}, {"SDSS3256", 0.108, 19.495, 0.942, -0.029,
0.222}, {"SDSS7147", 0.11, 19.516, 0.796, -0.034,
0.195}, {"SDSS8719", 0.116, 19.392, 0.992, -0.059,
0.199}, {"SDSS5395", 0.117, 19.459, 1.11, 0.002,
0.188}, {"SDSS2561", 0.118, 19.813, 0.993, 0.086,
0.193}, {"SDSS1371", 0.119, 19.073, 1.072, -0.076,
0.187}, {"SDSS5549", 0.121, 19.654, 1.02, 0.033,
0.182}, {"SDSS2916", 0.124, 19.937, 0.875, 0.066,
0.248}, {"06D2fb", 0.124, 19.772, 0.964, -0.004,
0.181}, {"SDSS6406", 0.125, 19.616, 1, 0.026, 0.188}, {"SDSS2992",
0.127, 20.034, 0.889, 0.127, 0.21}, {"SDSS744", 0.128, 19.793,
1.149, 0.08, 0.25}, {"SDSS5751", 0.13, 20.136, 1.068, 0.191,
0.179}, {"SDSS1032", 0.13, 20.326, 0.717, 0.088,
0.219}, {"SDSS2635", 0.143, 19.83, 1.092, -0.015,
0.202}, {"SDSS1794", 0.143, 20.058, 1.136, 0.018,
0.244}, {"SDSS8921", 0.145, 19.961, 1.104, 0.007,
0.231}, {"SDSS5103", 0.146, 20.377, 0.963, 0.055,
0.191}, {"SDSS11300", 0.147, 20.309, 0.862, 0.11,
0.243}, {"SDSS10106", 0.147, 20.948, 0.99, 0.2,
0.233}, {"SDSS2308", 0.148, 19.587, 1.069, -0.164,
0.189}, {"SDSS2031", 0.153, 19.703, 1.049, -0.091,
0.195}, {"SDSS5550", 0.156, 19.844, 1.202, -0.055,
0.19}, {"SDSS2689", 0.162, 20.254, 1.165, 0.095,
0.202}, {"SDSS3087", 0.165, 20.266, 1.056, 0.025,
0.196}, {"05D3ne", 0.169, 20.251, 0.809, -0.147,
0.218}, {"SDSS5916", 0.172, 20.439, 0.922, 0.014,
0.206}, {"SDSS3080", 0.174, 20.236, 0.999, -0.038,
0.195}, {"SDSS5350", 0.175, 20.323, 0.913, -0.057,
0.248}, {"SDSS5635", 0.179, 20.923, 1.011, 0.002,
0.233}, {"SDSS2372", 0.181, 20.58, 1.032, 0.045,
0.21}, {"SDSS6936", 0.181, 20.575, 1.003, -0.007,
0.208}, {"SDSS1580", 0.183, 20.291, 1.099, -0.014,
0.215}, {"05D2ah", 0.184, 20.765, 0.991, 0.019,
0.184}, {"SDSS6422", 0.184, 20.274, 1.08, -0.097,
0.193}, {"SDSS8213", 0.185, 21.133, 0.923, 0.179,
0.226}, {"SDSS5994", 0.187, 20.476, 1.074, -0.041,
0.218}, {"SDSS6304", 0.19, 20.952, 0.927, 0.095,
0.207}, {"SDSS762", 0.191, 20.657, 1.102, 0.009,
0.211}, {"SDSS2440", 0.193, 20.653, 1.051, -0.062,
0.212}, {"SDSS7335", 0.198, 21.265, 0.781, 0.067,
0.243}, {"SDSS6780", 0.202, 20.947, 0.789, -0.004,
0.246}, {"SDSS7243", 0.204, 20.789, 1.079, 0.002,
0.237}, {"SDSS3331", 0.206, 21.089, 0.95, 0.076,
0.225}, {"04D1dc", 0.211, 21.084, 0.856, 0.023,
0.191}, {"SDSS7847", 0.212, 21.225, 1.017, 0.155,
0.224}, {"SDSS6933", 0.213, 20.832, 0.995, 0.002,
0.202}, {"SDSS8495", 0.214, 20.811, 1.098, -0.001,
0.246}, {"SDSS1316", 0.217, 20.907, 1.058, -0.073,
0.374}, {"SDSS9467", 0.218, 21.057, 0.83, -0.118,
0.269}, {"05D3kx", 0.219, 20.867, 1.069, -0.016,
0.179}, {"SDSS7512", 0.219, 21.104, 1.055, 0.027,
0.233}, {"SDSS5533", 0.22, 21.173, 0.976, 0.046,
0.21}, {"SDSS3452", 0.23, 20.799, 1.092, -0.068,
0.211}, {"SDSS10449", 0.244, 20.995, 1.109, 0.039,
0.285}, {"SDSS3377", 0.245, 20.791, 1.12, -0.06,
0.219}, {"05D3mq", 0.246, 21.521, 0.912, 0.034,
0.204}, {"SDSS3451", 0.25, 20.958, 1.055, -0.038,
0.22}, {"06D3gn", 0.25, 21.892, 0.949, 0.16, 0.186}, {"SDSS3199",
0.251, 21.539, 1.164, 0.03, 0.224}, {"SDSS5717", 0.252, 21.355,
1.175, -0.012, 0.211}, {"SDSS5736", 0.253, 21.421, 0.949, 0.011,
0.211}, {"SDSS9032", 0.254, 21.345, 1.075, 0.041,
0.271}, {"SDSS9457", 0.257, 21.453, 0.978, 0.022,
0.302}, {"SDSS1112", 0.258, 21.563, 0.918, 0.022,
0.302}, {"SDSS8046", 0.259, 21.633, 1.049, 0.077,
0.249}, {"SDSS6108", 0.259, 21.537, 1.065, 0.063,
0.246}, {"SDSS3241", 0.259, 21.01, 1.057, -0.168,
0.251}, {"SDSS1253", 0.262, 21.23, 0.871, -0.09,
0.253}, {"SDSS2017", 0.262, 21.286, 1.148, -0.085,
0.26}, {"04D3ez", 0.263, 21.697, 0.891, 0.089, 0.184}, {"05D1hk",
0.263, 21.184, 1.168, -0.006, 0.192}, {"SDSS2422", 0.265, 21.144,
1.112, -0.156, 0.215}, {"SDSS2943", 0.265, 21.372, 1.055, 0.007,
0.24}, {"SDSS6315", 0.267, 20.919, 0.971, -0.166,
0.233}, {"06D3fp", 0.268, 21.748, 0.999, 0.104, 0.18}, {"03D4cj",
0.27, 21.052, 1.124, -0.063, 0.184}, {"SDSS6192", 0.272, 21.698,
0.826, -0.018, 0.268}, {"SDSS5957", 0.28, 21.453, 0.983, -0.089,
0.245}, {"06D3dt", 0.282, 22.168, 0.986, 0.117, 0.192}, {"03D4ag",
0.285, 21.277, 1.111, -0.043, 0.19}, {"SDSS2165", 0.288, 21.604,
1.063, -0.096, 0.245}, {"SDSS2789", 0.29, 21.576, 0.901, -0.077,
0.27}, {"03D3ba", 0.291, 21.984, 1.084, 0.146,
0.238}, {"SDSS6249", 0.294, 21.821, 1.086, 0.064,
0.256}, {"SDSS10550", 0.3, 22.02, 1.162, 0.105, 0.372}, {"06D4dh",
0.303, 21.449, 1.052, -0.126, 0.185}, {"SDSS11864", 0.303,
22.299, 1.015, 0.07, 0.432}, {"SDSS5966", 0.31, 21.798, 1.02,
0.002, 0.315}, {"SDSS6699", 0.311, 21.796, 0.872, -0.126,
0.277}, {"SDSS5844", 0.311, 21.571, 1.015, -0.099,
0.254}, {"SDSS6649", 0.314, 21.598, 1.09, -0.057,
0.251}, {"SDSS7475", 0.322, 21.535, 1.025, -0.123,
0.251}, {"05D2ab", 0.323, 22.001, 0.987, -0.013,
0.191}, {"SDSS6924", 0.328, 21.633, 1.076, -0.041,
0.265}, {"03D1fc", 0.332, 21.866, 1.048, 0.016, 0.194}, {"04D3kr",
0.337, 21.957, 1.127, -0.004, 0.184}, {"SDSS2533", 0.34, 21.79,
1.191, -0.04, 0.278}, {"04D3nh", 0.34, 22.142, 1.059, 0.009,
0.184}, {"03D1bp", 0.347, 22.421, 0.88, 0.002, 0.192}, {"04D2mc",
0.348, 22.58, 0.845, 0.142, 0.205}, {"05D2ie", 0.348, 22.249,
0.988, -0.046, 0.198}, {"SDSS9207", 0.35, 22.062, 1.126, 0.022,
0.322}, {"05D2hc", 0.35, 22.693, 0.931, 0.057, 0.194}, {"05D2mp",
0.354, 22.417, 1.138, 0.058, 0.208}, {"03D3bl", 0.355, 22.951,
1.002, 0.241, 0.211}, {"04D2fs", 0.357, 22.437, 1.01, 0.081,
0.191}, {"04D3fk", 0.358, 22.537, 0.96, 0.11, 0.184}, {"04D1hd",
0.369, 22.166, 1.071, -0.06, 0.179}, {"04D2cf", 0.369, 22.491,
0.882, 0.015, 0.265}, {"05D3jr", 0.37, 22.663, 0.902, 0.096,
0.189}, {"03D3ay", 0.371, 22.293, 1.054, -0.018,
0.234}, {"05D4bm", 0.372, 22.22, 1.02, -0.041, 0.183}, {"05D4fo",
0.373, 22.463, 0.924, -0.022, 0.183}, {"05D4cw", 0.375, 22.145,
0.911, -0.12, 0.187}, {"SDSS7779", 0.381, 21.943, 1.124, -0.049,
0.25}, {"SDSS5737", 0.393, 22.439, 1.27, 0.144,
0.339}, {"SDSS8707", 0.395, 22.272, 1.11, -0.088,
0.26}, {"05D4ff", 0.402, 22.615, 0.932, 0.028, 0.192}, {"06D3ed",
0.404, 22.615, 0.963, -0.036, 0.182}, {"05D4dt", 0.407, 22.808,
0.891, -0.023, 0.186}, {"06D4cq", 0.411, 22.562, 1.04, -0.005,
0.184}, {"04D2fp", 0.415, 22.559, 1.034, -0.012,
0.197}, {"05D2dw", 0.417, 22.488, 1.125, 0.021, 0.198}, {"05D3cf",
0.419, 22.965, 0.97, 0.045, 0.203}, {"04D4gg", 0.424, 22.753,
1.131, 0.124, 0.207}, {"05D2cb", 0.427, 23.407, 1.1, 0.193,
0.206}, {"04D1rh", 0.435, 22.582, 1.085, -0.015,
0.203}, {"06D4co", 0.437, 22.521, 0.96, -0.027, 0.183}, {"06D2gb",
0.442, 23.008, 0.829, 0.03, 0.23}, {"06D3df", 0.442, 22.685,
1.122, 0.021, 0.195}, {"03D3aw", 0.449, 22.654, 1.066, -0.053,
0.241}, {"04D2gb", 0.45, 22.916, 0.831, 0.042, 0.21}, {"04D3gt",
0.451, 23.259, 0.976, 0.223, 0.192}, {"03D3cd", 0.461, 22.593,
1.208, 0.012, 0.293}, {"05D3lc", 0.461, 22.982, 0.913, -0.021,
0.187}, {"03D4au", 0.468, 23.817, 1.048, 0.158, 0.239}, {"05D3mx",
0.47, 23.043, 0.832, -0.057, 0.202}, {"04D4jr", 0.47, 22.642,
1.16, -0.026, 0.195}, {"04D3df", 0.47, 23.521, 0.787, 0.108,
0.199}, {"04D4ju", 0.472, 23.771, 1.045, 0.184, 0.214}, {"05D2bv",
0.474, 22.719, 0.989, -0.096, 0.197}, {"05D2ac", 0.479, 22.677,
1.133, -0.012, 0.191}, {"05D3dd", 0.48, 22.941, 0.985, -0.015,
0.203}, {"05D1ix", 0.49, 22.879, 1.054, -0.034, 0.198}, {"03D1ax",
0.496, 22.992, 0.925, -0.062, 0.196}, {"05D4af", 0.499, 23.108,
1.01, -0.013, 0.222}, {"06D2bk", 0.499, 23.273, 1.054, 0.036,
0.234}, {"03D1au", 0.504, 23.012, 1.137, 0.017, 0.203}, {"05D4av",
0.509, 23.558, 1.095, 0.185, 0.204}, {"05D2dy", 0.51, 22.913,
1.099, -0.1, 0.211}, {"04D2mj", 0.513, 23.783, 1.154, 0.171,
0.216}, {"04D1pg", 0.515, 23.57, 1.092, 0.119, 0.218}, {"05D3ci",
0.515, 23.564, 1.166, 0.16, 0.278}, {"04D4in", 0.516, 22.902,
1.169, -0.034, 0.201}, {"06D3el", 0.519, 22.913, 1.08, -0.082,
0.196}, {"04D2gc", 0.522, 23.327, 1.133, 0.037, 0.219}, {"06D2ca",
0.531, 23.301, 1.099, 0.06, 0.249}, {"06D2cc", 0.532, 23.468,
0.944, 0.089, 0.256}, {"05D2eb", 0.534, 23.006, 1.113, -0.036,
0.233}, {"05D4ek", 0.536, 23.303, 1.059, 0.081, 0.21}, {"05D4be",
0.537, 22.916, 1.1, -0.114, 0.199}, {"04D4bq", 0.55, 23.347,
1.095, 0.134, 0.23}, {"04D3hn", 0.552, 23.503, 0.935, 0.096,
0.21}, {"06D2ck", 0.552, 23.447, 1.05, -0.002, 0.249}, {"06D4bo",
0.552, 23.231, 1.098, -0.028, 0.227}, {"05D1ee", 0.559, 23.556,
0.953, 0.023, 0.222}, {"04D1hx", 0.56, 23.715, 1.042, 0.143,
0.215}, {"05D1kl", 0.56, 24.154, 1.075, 0.164, 0.246}, {"05D1cc",
0.563, 23.496, 0.981, -0.005, 0.211}, {"05D1dn", 0.566, 23.317,
1.129, 0.018, 0.222}, {"03D4gl", 0.571, 23.314, 1.238, 0.039,
0.831}, {"05D2dt", 0.574, 23.656, 1.024, 0.045, 0.249}, {"06D3et",
0.576, 23.512, 0.861, -0.039, 0.213}, {"05D3jq", 0.579, 23.322,
1.161, 0.035, 0.209}, {"05D3gp", 0.58, 23.521, 0.946, -0.054,
0.286}, {"03D4gf", 0.58, 23.336, 1.095, 0.01, 0.236}, {"05D1dx",
0.58, 23.304, 1.078, -0.027, 0.205}, {"03D1aw", 0.582, 23.584,
1.098, 0.001, 0.235}, {"04D1jg", 0.584, 23.272, 1.028, -0.079,
0.219}, {"04D1kj", 0.585, 23.345, 1.034, -0.052,
0.211}, {"05D4ej", 0.585, 23.746, 1.034, 0.02, 0.222}, {"04D1sa",
0.585, 23.559, 0.94, -0.064, 0.237}, {"05D1hm", 0.587, 24.102,
1.126, 0.155, 0.244}, {"05D4bf", 0.589, 23.627, 1.029, 0.018,
0.235}, {"04D2mh", 0.59, 23.403, 1.163, 0.041, 0.215}, {"04D1oh",
0.59, 23.388, 1.01, -0.048, 0.227}, {"03D4gg", 0.592, 23.413,
1.098, 0.046, 0.264}, {"05D3lr", 0.6, 23.854, 1.02, 0.096,
0.251}, {"05D4ef", 0.605, 23.832, 0.839, -0.054,
0.225}, {"05D2he", 0.608, 23.953, 1.044, 0.073, 0.244}, {"03D4dy",
0.61, 23.268, 1.127, -0.06, 0.231}, {"04D3do", 0.61, 23.577,
0.906, -0.097, 0.213}, {"03D1dt", 0.612, 23.3, 1.048, -0.054,
0.273}, {"04D4an", 0.613, 24.046, 0.987, 0.025, 0.27}, {"05D1ck",
0.617, 24.074, 1.007, 0.111, 0.231}, {"04D2an", 0.62, 23.597,
0.991, -0.019, 0.295}, {"04D3co", 0.62, 23.757, 0.936, 0.019,
0.245}, {"03D4dh", 0.627, 23.39, 1.13, -0.045, 0.225}, {"04D4fx",
0.629, 23.501, 1.115, 0.01, 0.23}, {"05D2ci", 0.63, 23.612, 0.901,
0.045, 0.263}, {"05D1cb", 0.632, 23.715, 0.967, -0.001,
0.222}, {"03D4at", 0.634, 23.733, 1.019, -0.008,
0.261}, {"04D1pu", 0.639, 24.024, 0.843, 0.094, 0.291}, {"05D2ec",
0.64, 23.672, 0.994, -0.063, 0.248}, {"05D4ag", 0.64, 23.895,
1.055, 0.068, 0.287}, {"05D3ax", 0.643, 23.62, 1.071, -0.034,
0.28}, {"04D3cy", 0.643, 23.8, 0.978, -0.011, 0.257}, {"05D3lb",
0.647, 23.896, 1.04, 0.035, 0.224}, {"05D3kt", 0.648, 23.965,
0.979, 0.085, 0.23}, {"04D1sk", 0.663, 24.058, 1.028, 0.1,
0.251}, {"05D3hs", 0.664, 23.501, 1.045, -0.141,
0.234}, {"05D3mh", 0.67, 24.106, 1.084, 0.053, 0.272}, {"03D1co",
0.679, 24.088, 1.084, -0.019, 0.266}, {"05D2bt", 0.68, 23.521,
1.067, -0.113, 0.239}, {"06D3cc", 0.683, 24.067, 1.082, 0.006,
0.312}, {"04D4ic", 0.687, 24.121, 0.9, -0.01, 0.276}, {"06D3em",
0.69, 24.377, 1.006, 0.149, 0.259}, {"05D1ke", 0.69, 23.611,
1.035, -0.077, 0.23}, {"03D4cz", 0.695, 24.045, 0.818, -0.071,
0.292}, {"05D2ck", 0.698, 24.474, 0.733, -0.015,
0.277}, {"04D4ib", 0.699, 23.595, 1.101, -0.093,
0.233}, {"04D2iu", 0.7, 24.246, 0.806, 0.021, 0.288}, {"06D4ba",
0.7, 23.761, 1.065, -0.081, 0.28}, {"05D2le", 0.7, 23.961,
1.054, -0.012, 0.247}, {"05D4cq", 0.701, 23.73, 1.077, -0.058,
0.24}, {"05D4bj", 0.701, 24.103, 1.012, 0.059, 0.249}, {"04D1si",
0.702, 23.867, 0.994, -0.003, 0.244}, {"04D4hu", 0.703, 23.92,
1.005, -0.078, 0.241}, {"05D3gv", 0.715, 24.001, 0.911, -0.043,
0.254}, {"05D3jh", 0.718, 23.753, 0.927, -0.096, 0.23}, {"06D3gh",
0.72, 23.926, 1.034, -0.021, 0.27}, {"04D1aj", 0.721, 23.904,
1.03, -0.004, 0.266}, {"05D4ev", 0.722, 24.259, 0.896, -0.014,
0.259}, {"06D3do", 0.725, 23.898, 1.096, -0.044,
0.281}, {"06D3bz", 0.727, 23.959, 0.923, -0.057,
0.268}, {"04D2gp", 0.732, 24.219, 0.849, -0.091, 0.29}, {"06D4bw",
0.732, 23.904, 1.066, 0.005, 0.25}, {"05D2fq", 0.733, 23.997,
1.084, -0.033, 0.249}, {"05D2ct", 0.734, 24.385, 1.042, 0.093,
0.293}, {"04D1pp", 0.735, 23.998, 0.865, -0.064,
0.236}, {"05D3jk", 0.736, 23.717, 1.082, -0.079,
0.227}, {"05D1eo", 0.737, 24.316, 0.854, -0.038,
0.255}, {"04D2ja", 0.74, 24.129, 1.04, -0.131, 0.281}, {"04D3fq",
0.742, 24.116, 0.956, -0.03, 0.264}, {"04D2kr", 0.744, 23.865,
1.057, -0.02, 0.247}, {"05D3jb", 0.745, 23.939, 1.102, -0.025,
0.235}, {"04D3ks", 0.75, 23.855, 1.075, -0.03, 0.257}, {"04D4im",
0.751, 23.852, 1.148, 0.043, 0.245}, {"04D3oe", 0.756, 24.06,
0.908, -0.161, 0.258}, {"05D2nt", 0.757, 24.099, 1.156, -0.006,
0.247}, {"05D3mn", 0.76, 24.043, 0.987, -0.021, 0.244}, {"06D3gx",
0.76, 23.885, 1.043, -0.089, 0.296}, {"05D4cn", 0.763, 24.102,
1.111, 0.015, 0.243}, {"05D1if", 0.763, 24.059, 1.019, -0.035,
0.243}, {"05D3hh", 0.766, 24.319, 1.07, 0.018, 0.279}, {"04D1qd",
0.767, 24.228, 1.007, 0.012, 0.245}, {"04D1de", 0.768, 24.144,
1.101, -0.079, 0.241}, {"04D4id", 0.769, 24.212, 1.074, -0.1,
0.276}, {"04D1pc", 0.77, 24.553, 0.97, 0.067, 0.256}, {"05D4bi",
0.775, 24.051, 1.098, -0.094, 0.268}, {"04D1jd", 0.778, 24.425,
1.01, 0.051, 0.258}, {"05D4cs", 0.79, 23.967, 1.135, -0.061,
0.236}, {"03D4fd", 0.791, 24.232, 1.072, -0.024,
0.288}, {"04D1ks", 0.798, 24.145, 1.079, 0.076, 0.251}, {"05D3dh",
0.8, 24.203, 1.009, 0.078, 0.26}, {"03D1fq", 0.8, 24.512,
0.87, -0.045, 0.272}, {"05D3cx", 0.805, 23.913, 1.051, -0.11,
0.266}, {"05D3ha", 0.805, 24.388, 0.972, 0.09, 0.287}, {"05D4gw",
0.808, 24.481, 1.025, 0.047, 0.288}, {"05D4dy", 0.81, 24.617,
0.956, -0.083, 0.275}, {"04D3ny", 0.81, 24.262, 1.024, 0.011,
0.31}, {"04D4dm", 0.811, 24.402, 0.981, 0.021, 0.267}, {"04D3mk",
0.813, 24.294, 0.954, -0.104, 0.254}, {"04D3nc", 0.817, 24.293,
1.139, -0.022, 0.28}, {"06D2ce", 0.82, 24.215, 1.219, 0.018,
0.333}, {"04D3lu", 0.822, 24.377, 0.934, -0.084, 0.25}, {"05D1cl",
0.83, 24.353, 1.242, -0.018, 0.269}, {"04D3cp", 0.83, 24.111,
1.049, -0.18, 0.256}, {"04D2al", 0.836, 24.319, 0.933, -0.04,
0.372}, {"Elvis", 0.839, 24.397, 0.985, 0, 0.263}, {"05D4fg",
0.839, 24.195, 1.024, -0.102, 0.254}, {"06D2ga", 0.84, 24.29,
1.176, -0.001, 0.356}, {"05D1az", 0.842, 24.254, 1.184, 0.015,
0.269}, {"05D4hn", 0.842, 24.196, 1.122, 0.048, 0.319}, {"04D1hy",
0.85, 24.307, 1.11, -0.026, 0.254}, {"06D4ce", 0.85, 24.205,
1.168, -0.059, 0.303}, {"05D3kp", 0.85, 24.139, 1.131, -0.082,
0.243}, {"05D4dw", 0.855, 24.438, 1.046, 0.008, 0.278}, {"04D1ff",
0.86, 24.243, 1.08, 0.046, 0.257}, {"05D1iz", 0.86, 24.392,
1.095, -0.082, 0.336}, {"05D1er", 0.86, 24.618, 1.042, 0.062,
0.29}, {"03D1bk", 0.865, 24.345, 1.018, -0.167, 0.263}, {"05D1em",
0.866, 24.283, 1.017, -0.1, 0.26}, {"04D4ii", 0.866, 24.399,
1.165, 0.039, 0.284}, {"03D1ew", 0.868, 24.359, 1.036, -0.036,
0.284}, {"05D2nn", 0.87, 24.395, 0.879, -0.147, 0.321}, {"04D4bk",
0.88, 24.327, 1.181, -0.061, 0.28}, {"05D3cq", 0.89, 24.22,
0.96, -0.151, 0.278}, {"05D2by", 0.891, 24.568, 1.132, -0.008,
0.29}, {"03D4di", 0.899, 24.314, 1.146, -0.043, 0.29}, {"05D3ht",
0.901, 24.417, 1.118, -0.095, 0.282}, {"04D3gx", 0.91, 24.666,
0.94, -0.11, 0.293}, {"04D1ow", 0.915, 24.366, 1.004, -0.124,
0.268}, {"05D2bw", 0.92, 24.4, 0.972, -0.111, 0.319}, {"05D2ay",
0.92, 24.672, 0.983, 0.014, 0.349}, {"05D2ob", 0.924, 24.822,
1.103, 0.033, 0.318}, {"03D4cy", 0.927, 24.705, 1.103, -0.032,
0.346}, {"06D2cd", 0.93, 24.876, 1.166, 0.051, 0.49}, {"04D4jy",
0.93, 24.765, 1.022, -0.076, 0.345}, {"04D4ih", 0.934, 24.43,
1.03, -0.166, 0.276}, {"Vilas", 0.935, 24.473, 1.036, -0.028,
0.272}, {"04D4hf", 0.936, 24.811, 1.081, 0.028, 0.35}, {"05D3la",
0.936, 24.494, 0.967, -0.083, 0.267}, {"03D4cx", 0.949, 24.464,
0.947, 0.019, 0.331}, {"04D1pd", 0.95, 24.734, 1.039, 0.022,
0.298}, {"04D3ml", 0.95, 24.56, 1.117, -0.077, 0.29}, {"04D3nr",
0.96, 24.587, 0.99, 0.005, 0.299}, {"05D3km", 0.96, 24.773,
1.022, -0.123, 0.28}, {"04D4jw", 0.961, 24.848, 0.892, -0.173,
0.365}, {"Patuxent", 0.97, 25.026, 0.962, -0.129, 0.356}, {"Ombo",
0.975, 24.891, 1.208, 0.018, 0.271}, {"05D2my", 0.981, 24.688,
1.13, -0.026, 0.305}, {"04D3lp", 0.983, 25.023, 0.816, -0.044,
0.378}, {"04D1rx", 0.985, 24.77, 1.081, -0.062, 0.307}, {"04D1iv",
0.998, 24.624, 1.152, -0.074, 0.285}, {"06D4cl", 1, 24.578,
1.13, -0.065, 0.296}, {"04D3dd", 1.002, 25.234, 1.112, -0.016,
0.412}, {"Strolger", 1.01, 24.99, 1.195, -0.077, 0.433}, {"Eagle",
1.02, 24.968, 1.017, -0.061, 0.289}, {"Ferguson", 1.02, 24.867,
1.023, 0.007, 0.329}, {"04D4dw", 1.031, 24.546, 1.127, -0.08,
0.326}, {"06D3en", 1.06, 24.756, 0.858, -0.139, 0.358}, {"Gabi",
1.12, 25.121, 1.048, -0.037, 0.278}, {"Greenberg", 1.14, 24.727,
1.09, -0.055, 0.325}, {"Lancaster", 1.23, 26.054, 0.969, 0.073,
0.335}, {"Torngasek", 1.265, 25.757, 1.04, 0.028,
0.354}, {"Aphrodite", 1.3, 25.691, 1.058, 0.013, 0.284}, {"Borg",
1.34, 25.87, 1.192, 0.09, 0.389}, {"Sasquatch", 1.39, 25.956,
1.193, 0.112, 0.535}, {"Primo", 1.54992, 25.7576,
0.168369, -0.197134, 0.6329682}, {" GND13Sto ", 1.8, 26.1369,
0.527158, -0.0156538, 0.9455797}, {"SN UDS10Wil", 1.914,
26.2, -0.5, -0.071, 0.85}, {"GND12Col", 2.25, 26.791, 1.1517,
0.0421647, 1.381078}};
And here is the function that selects the minimum value:
FindMinimum[{chiSquared[t1, a0]}, {{t1, 1*^16}, {a0, 1}}]
This will return:
{111076., {t1 -> 6.65636*10^18, a0 -> 3.87928*10^-14}}
Which is pretty close, but if you remove the initializers, or set t1 to 1, then it's unable to find a minimum.
FindMinimum[{chiSquared[t1, a0]}, {t1, a0}]
{1.49713*10^7, {t1 -> 72.0749, a0 -> -2.21105*10^7}}
How do I get the FindMinimum function to work if I don't know the answer?

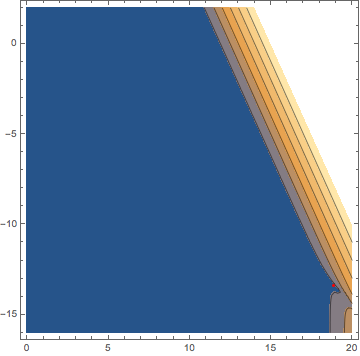

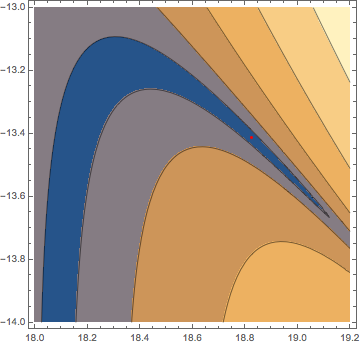
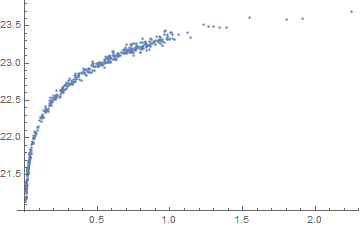
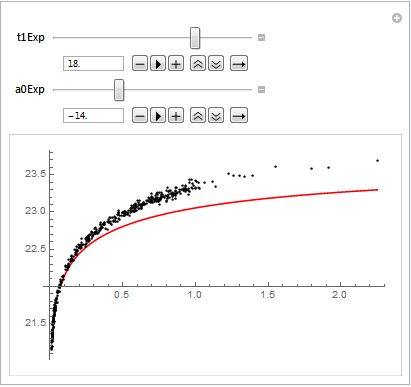
FindFit[]? That explicitly uses LM by default unless told otherwise. If you insist on usingFindMinimum[]then setMethod -> "LevenbergMarquardt"directly. But you still need to give good seeds, and that is something that you who (is supposed to) know the data should be able to supply. $\endgroup$