As in version 10.2, there is the NDEigensystem can be used to calculate the eigenstates and eigenvalues of a differential operator.
For example in the harmonic potential case, the even and odd eigen functions can be calculated seperatelyusing Neumann and Dirichlet boundary condition respectively:
{egnVal1, egnVec1} =
NDEigensystem[{-1/2 Laplacian[u[x], {x}] + 1/2 x^2 u[x]},
u[x], {x, 0, 10}, 2];
{egnVal2, egnVec2} =
NDEigensystem[{-1/2 Laplacian[u[x], {x}] + 1/2 x^2 u[x],
DirichletCondition[u[x] == 0, True]}, u[x], {x, 0, 10}, 2];
And the eigenenergies are:
egnVal = Riffle[egnVal1, egnVal2]
(* {0.500079, 1.50054, 2.5019, 3.5047} *)
And the eigenstates are:
egnVec = Riffle[egnVec1, egnVec2];
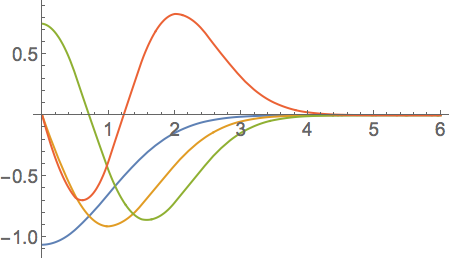
Plot[Evaluate[egnVec], {x, 0, 6}, PlotRange -> All]
which gives the correct wave functions apart from the arbitrary phases.