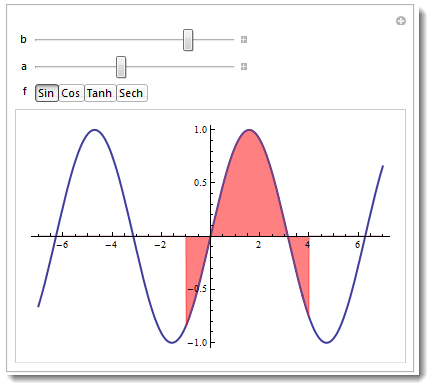
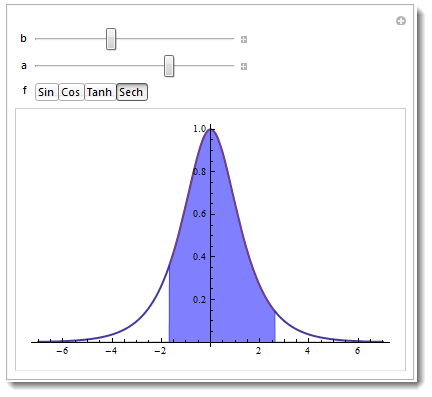
Area will be red or blue depending whether b > a$b > a$ or not.
Manipulate[
Plot[{f[x], UnitStep[Sign[b - a] (x - a)] UnitStep[Sign[b - a] (b - x)] f[x]},
{x, -7, 7}, PlotStyle -> {Thick, Thickness[0]}, Filling -> {2 -> 0},
FillingStyle -> Directive[Opacity[.5], If[b - a > 0, Red, Blue]],
PlotLabel -> "AREA = " <> ToString[NIntegrate[f[x], {x, a, b}]]], {{b, 4}, -7, 7},
{{a, -1}, -7, 7}, {f, {Sin, Cos, Tanh, Sech}}]
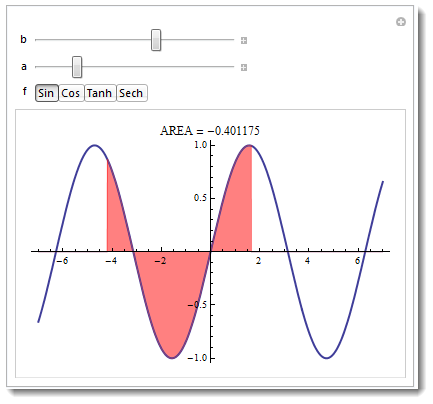
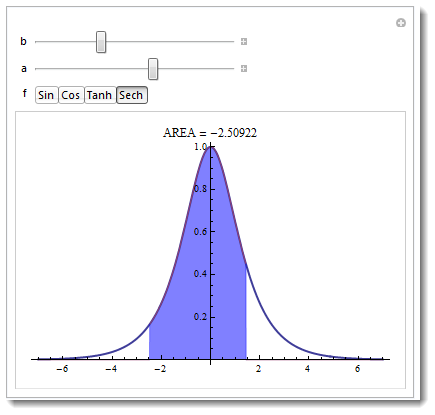
Also take a look at source code at the Wolfram Demonstration Project.