Yaroslav Bulatov posted a great answera great answer addressing this problem on StackOverflow.
Among several good illustrations the answer includes this undocumented option:
Method -> {Refinement -> {ControlValue -> (*radians*) }}
Alexey Popkov also gave an excellent analysis of the Plot algorithm.an excellent analysis of the Plot algorithm.
His answer includes the apparently equivalent but cleaner (in degrees rather than radians as above):
Method-> {MaxBend -> (*degrees*) }
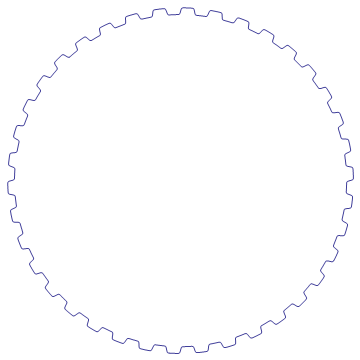
Example:
gearCurve[a_, b_, n_] :=
ParametricPlot[
{(a + 1/b Tanh[b Sin[n t]]) Cos[t],
(a + 1/b Tanh[b Sin[n t]]) Sin[t]},
{t, 0, 2 Pi},
Axes -> False,
Method-> {MaxBend -> 1}
];
gearCurve[10, 5, 38]