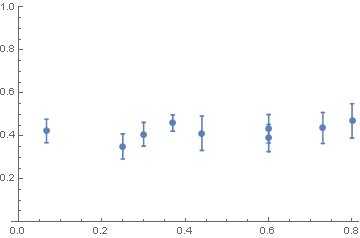
Your data:
data = {{0.067, 0.423}, {0.30, 0.408}, {0.60, 0.433}, {0.25, 0.3512}, {0.37, 0.4602}, {0.44, 0.413}, {0.60, 0.390}, {0.73, 0.437}, {0.8, 0.47}};
errors = {0.055, 0.0552, 0.0662, 0.0583, 0.0378, 0.080, 0.063, 0.072, 0.08};
ErrorListPlot[Transpose[{data, ErrorBar /@ errors}], PlotRange -> {0, 1}]
Assume that the errors are distributed Normally with standard deviation given by your errors. Then we can define
diffErr[y1_, s1_, x1_, y2_, s2_, x2_] := Block[{distr, slope, error},
distr =
TransformedDistribution[(v - u)/(
x2 - x1), {u \[Distributed] NormalDistribution[y1, s1],
v \[Distributed] NormalDistribution[y2, s2]}];
slope = Mean[distr];
error = StandardDeviation[distr];
{slope, error}
]
That is the calculation of the slope, provided the vertical values are normally distributed with StdDev given by your errors.
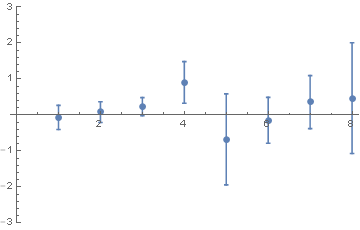
And now the slopes between points ie the numerical derivative isderivatives are then
slopes=Table[diffErr[data[[i, 2]], errors[[i]], data[[i, 1]], data[[i + 1, 2]], errors[[i + 1]], data[[i + 1, 1]]], {i, Length[data] - 1}]
{{-0.0643777, 0.334435}, {0.0833333, 0.287315}, {0.233714, 0.252034}, {0.908333, 0.579016}, {-0.674286, 1.26401}, {-0.14375, 0.636427}, {0.361538, 0.735933}, {0.471429, 1.53756}}
ErrorListPlot[slopes, PlotRange -> {-3, 3}]