Note: this is fixed in version 9.
My question concerns the usage of NExpectation and Expectation and why I see the behavior I see in the following example.
First take some data and derive an EmpiricalDistribution:
data = {4, 104, 96, 80, 22, 76, 106, 18, 98, 44, 112, 78, 50, 120, 2, 6,
100, 10, 68, 80, 42, 66, 100, 58, 4, 76, 18, 102, 6, 16, 52, 32, 62,
36, 18, 4, 54, 98, 38, 74, 16, 22, 102, 2, 2, 4, 22, 72, 100, 82, 48,
16, 34, 44, 130, 50, 48, 74, 60, 96, 8, 118, 30, 58, 84, 4, 70, 66,
40, 14, 92, 68, 42, 56, 56, 16, 40, 12, 22, 26, 98, 4, 80, 100, 36,
88, 48, 26, 28, 94, 22, 26, 78, 16, 52, 8, 10, 2};
dist = EmpiricalDistribution[data];
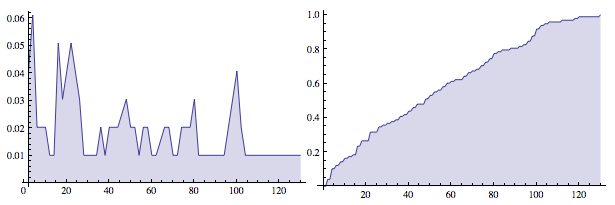
You can plot PDFs and CDFs of the distribution:
Row[{DiscretePlot[PDF[dist, x], {x, data}, Joined -> True, ImageSize -> 300],
DiscretePlot[CDF[dist, x], {x, 0, Max@data, 1}, Joined -> True, ImageSize -> 300]}]
They look like this:
That covers the background. Now execute the following and it gets a little odd:
Expectation[X \[Conditioned] X > 4, X \[Distributed] dist]
NExpectation[X \[Conditioned] X > 4, X \[Distributed] dist]
N[Expectation[X \[Conditioned] X > 4, X \[Distributed] dist]]
620/11
NExpectation[X \[Conditioned] X > 4, X \[Distributed]DataDistribution[<<"Empirical">>, {51}]]
56.3636
So, what gives?
How come NExpectation[...] doesn't calculate an answer, but N[Expectation[...]] does? Clearly, Expectation handles EmpiricalDistribution without a problem. One would think that NExpectation would as well. Doesn't this seem odd? Just hoping that understanding why Mathematica does this might yield additional insights into Mathematica itself.
