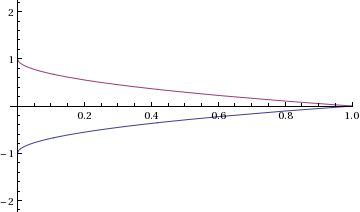
y1[x_] := Sqrt@x - 1
y2[x_] := -Sqrt@ x + 1
Plot[{y1[x], y2[x]}, {x, 0, x /. Last@Solve[y1[x] == y2[x]]}, PlotRange->{{0, 2}, {-1, 1}}]
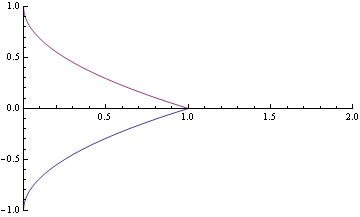
or you can play with PlotRange:
Plot[{y1[x], y2[x]}, {x, 0, 10},
PlotRange -> {{0, x /. Last@Solve[y1[x] == y2[x]]}, {-1, 1}}]