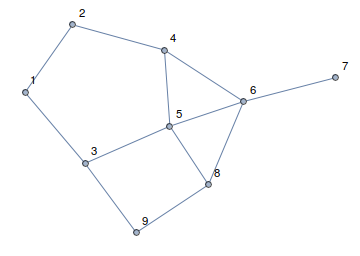
I am doing a research on networks which consists of polygons with different number of sides. I am trying to find all simple cycles in a network which are chordless. As an example, consider the following graph:
graph = Graph[
{
1 <-> 2, 2 <-> 3, 3 <-> 4, 4 <-> 5, 5 <-> 6, 1 <-> 6,
3 <-> 7, 7 <-> 8, 8 <-> 9, 9 <-> 10, 10 <-> 11, 11 <-> 3,
4 <-> 12, 12 <-> 13, 13 <-> 11
},
VertexLabels -> "Name"
]
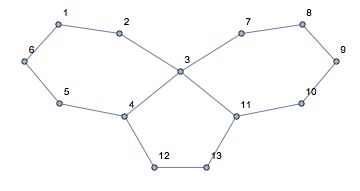
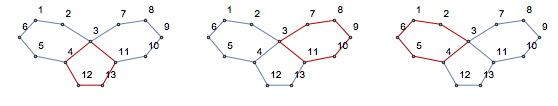
{1,2,3,4,5,6}, {3,4,11,12,13},{3,7,8,9,10,11} are rings and we can extract them by using:
cycles = FindFundamentalCycles[graph];
rings = Sort @* VertexList @* Graph /@ cycles
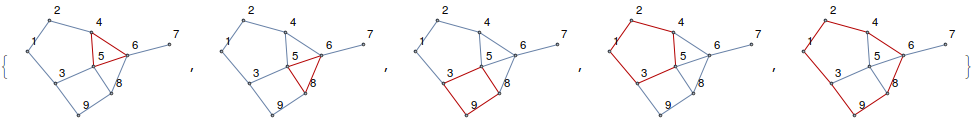
But the above solution doesn't always work as it might give non-chordless cycles. Consider the following example:
grapht = Graph[
{
1 <-> 2, 1 <-> 3, 2 <-> 4, 4 <-> 5, 5 <-> 6, 4 <-> 6,
6 <-> 7, 3 <-> 5, 3 <-> 9, 5 <-> 8, 8 <-> 9
},
VertexLabels -> "Name"];
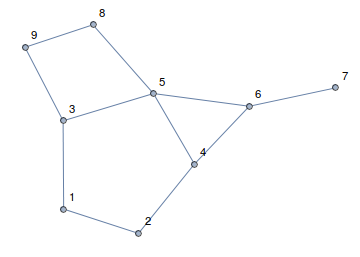
Rings (cycles) are:
cyclest = FindFundamentalCycles[grapht];
HighlightGraph[grapht, #] & /@ cyclest
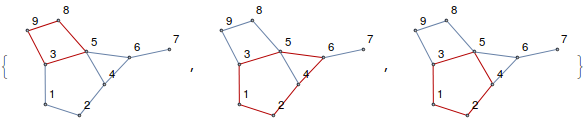
But I need to get {4,5,6} as a ring not {1,2,3,4,5,6} since there is an edge in the latter. Is there any way to filter out only chordless cycles?