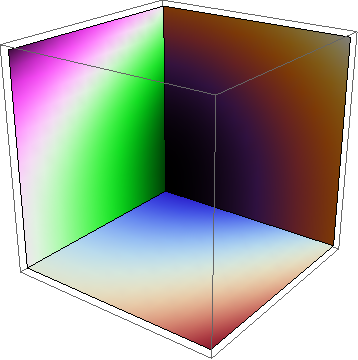
For example:
f[x_, y_, z_] := x + y y + z z z
data = Flatten[Table[{{x, y, z}, f[x, y, z]}, {x, 0, 1, .1}, {y, 0, 1, .1}, {z, 0, 1, .1}], 2];
fi = Interpolation@data;
dpx = Rasterize@ DensityPlot[fi[0, y, z], {y, 0, 1}, {z, 0, 1}, Frame -> False,
ColorFunction -> "SunsetColors"];"SunsetColors", PlotRangePadding -> 0];
dpy = Rasterize@ DensityPlot[fi[x, 0, z], {x, 0, 1}, {z, 0, 1}, Frame -> False,
ColorFunction -> "GreenPinkTones"];"GreenPinkTones", PlotRangePadding -> 0];
dpz = Rasterize@ DensityPlot[fi[x, y, 0], {x, 0, 1}, {y, 0, 1}, Frame -> False,
ColorFunction -> "ThermometerColors"];"ThermometerColors", PlotRangePadding -> 0];
Graphics3D[{
{Texture@dpx, Polygon[{{0, 0, 0}, {0, 1, 0}, {0, 1, 1}, {0, 0, 1}},
VertexTextureCoordinates -> {{0, 0}, {1, 0}, {1, 1}, {0, 1}}]},
{Texture@dpy, Polygon[{{0, 0, 0}, {1, 0, 0}, {1, 0, 1}, {0, 0, 1}},
VertexTextureCoordinates -> {{0, 0}, {1, 0}, {1, 1}, {0, 1}}]},
{Texture@dpz,
Polygon[{{0, 0, 0}, {1, 0, 0}, {1, 1, 0}, {0, 1, 0}},
VertexTextureCoordinates -> {{0, 0}, {1, 0}, {1, 1}, {0, 1}}]}},
Lighting -> "Neutral"]