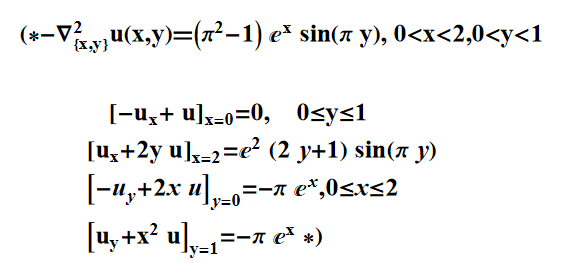equation is
and the symbol solution is $u[x,y]=e^x \sin(\pi y)$ mathematica code
sol = NDSolveValue[{-Inactive[Laplacian][
u[x, y], {x, y}] == (Pi^2 - 1)*E^x*Sin[Pi*y] -
NeumannValue[-u[x, y], x == 0] +
NeumannValue[E^2*(1 + 2*y)*Sin[Pi*y] - 2*y*u[x, y],
x == 2] - NeumannValue[(-Pi)*E^x - 2*x*u[x, y], y == 0] +
NeumannValue[(-Pi)*E^x - x^2*u[x, y], y == 1]},
u, {x, 0, 2}, {y, 0, 1}];
sol[x, y] /. {x -> 0.5, y -> 0.5}(*is negative, but the u[x,y]=E^x Sin[Pi y]=E^0.5*)
ContourPlot[sol[x, y], {x, 0, 2}, {y, 0, 1}, ContourStyle -> None,
PlotLegends -> Automatic];
but the numerical solution at $(0.5,0.5)$ is $u(0.5,0.5)=-3.24478$, is not equal $e^{0.5}$, and I don't know how wrong it is.