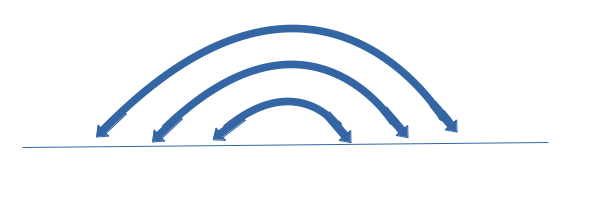
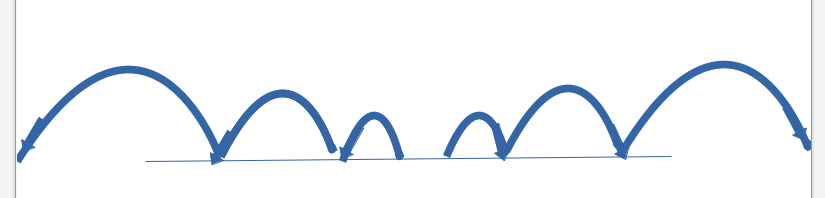
I am looking for a way to generate the phase portrait on the real line of the orbits of maps, such as
$$f(x)=-x$$
which orbit has the phase portrait :
Then another example:
$$g(x)=2x$$
has an orbit with the following phase portrait
This is explained in Devaney "Chaotic Dynamical Systems".
No such options are available for either Mathematica or MATLAB. Only the regular phase portrait for the solutions of ODEs. But this is phase portrait for maps and theirthe periods of their orbits.