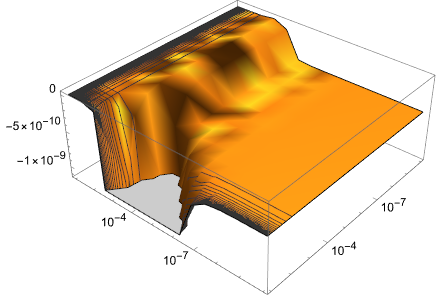
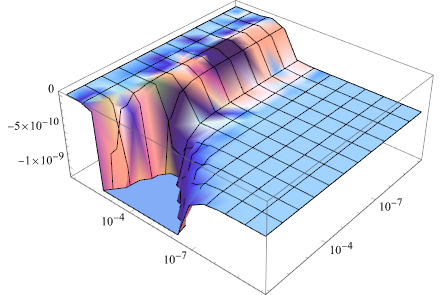
First plot
ClearAll["Global`*"]
c1 = 4631.0; c2 = 2280.1; d = 8066.8; e = 0.0168; k = 1;
eqn = (1 + (c2^2/c1^2)*w^2)*(1 +
k*taoT*c2*w + (c2*d/k)*
w*(1 + k*tauq*c2*w + (1/2)*k^2*tauq^2*c2^2*w^2)) + (e*c2*d/k)*
w*(1 + k*tauq*c2*w + (1/2)*k^2*tauq^2*c2^2*w^2) == 0;
sol=NSolveValues[eqn,w];

Plot[Re@sol[[2]]/.taoT->10^-1,{tauq,10^-10,10^-1},ScalingFunctions->{"Log",Identity},PerformanceGoal->"Quality"]
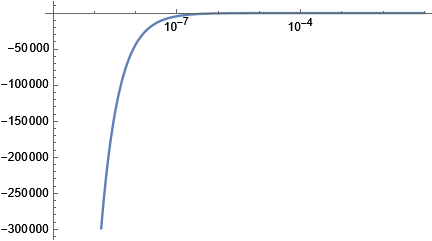
Second plot
Plot3D[Re@sol[[4]],{tauq,10^-10,10^-1},{taoT,10^-10,10^-1},
ScalingFunctions->{"Log","Log",Identity},PerformanceGoalMesh->"Quality"]>10,PlotTheme->"Classic"]