Another way is to use ParametricPlot (which will accomplish an equivalent thing via polygons). Here, thickness adds a multiple th of the unit normal to the curve. Just pass a thickness function as the parameter th.
thickness[f_, th_] := Block[{x}, {x, f} + Normalize[{-D[f, x], 1}] th];
ParametricPlot[
Evaluate@thickness[2 Sin[x], 0.075 (1 + Sin[x]^2) t],
{x, 0, 3 Pi}, {t, -1, 1},
Mesh -> None, BoundaryStyle -> None,
ColorFunction -> (ColorData["BlueGreenYellow"][#2] &)]

Update
This is a nicer interface. You pass the thickness function as an option. The function will have one parameter passed to it, namely the variable var of the plot.
ClearAll[thicknessPlot];
SetAttributes[thicknessPlot, HoldAll];
Options[thicknessPlot] = {thicknessFunction -> (0.1 &)} ~Join~ Options[ParametricPlot];
thicknessPlot[f_, {var_, v1_, v2_}, opts : OptionsPattern[]] :=
Module[{param},
With[{thicknessFn = OptionValue[thicknessFunction],
unitN = Block[{var}, Normalize[{-D[f, var], 1}]]},
ParametricPlot[{var, f} + thicknessFn[var] unitN param,
{var, v1, v2}, {param, -1, 1},
Mesh -> (OptionValue[Mesh] /. Automatic -> None),
BoundaryStyle -> (OptionValue[BoundaryStyle] /. Automatic -> None),
Evaluate @ FilterRules[FilterRules[{opts}, Options[ParametricPlot]],
Except[Mesh | BoundaryStyle]]
]]
]
Example:
thicknessPlot[2 Sin[x], {x, 0, 3 Pi},
thicknessFunction -> (0.01 + #/20 &),
ColorFunction -> (ColorData["BlueGreenYellow"][#2] &)]
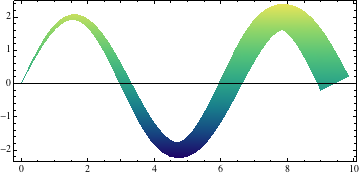
Note: Adding PlotPoints -> {15, 2} will speed things up, or if more points are needed for a complicated graph, then something like PlotPoints -> {50, 2}. Since the formula in ParametricPlot is a linear function of the thickness parameter param, two plot points for that dimension will usually be enough.
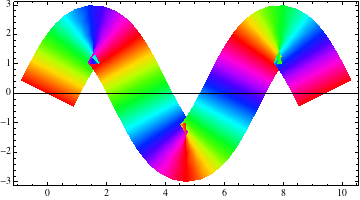
Also note that if half the thickness exceeds the radius of curvature, the curve will fold over itself. This is a problem with the mathematics, not the code (except that the code implements the mathematics).
thicknessPlot[2 Sin[x], {x, 0, 3 Pi}, thicknessFunction -> (1 &),
PlotPoints -> {15, 2}, ColorFunction -> (Hue[4 #3] &)]