For $n=\{{6,7,8}\}$, it take about $\{{1.43,\;19.69,\;860.85}\}$ seconds.
When $n=9$, it doesn't finished for $4$ hours, still very slow, I terminate it.
Still very slow, I tried to convert all the patterns to C code, it's not difficult, the generated C code is more than 5,700 lines, the efficiency improvement is very obvious.
n=9;
gps=Block[{i=1,j=1},Groupings[Table[x,n],f->2]/.
{f:>(F[#,Symbol["i"<>ToString@Mod[i++,n-1,1]],#2]&),x:>Symbol["n"<>ToString[Mod[j++,n,1]]]}];
gen=StringTemplate["if(check1(``)){
sprintf(buffer, \"``\\n\", ``);
if(check2(buffer)) printf(\"%s\", buffer);
}"][ToString@CForm@#,
StringReplace[ToString@#,{"F"|", "->"","["->"(","]"->")",
"n"~~DigitCharacter:>"%d","i"~~DigitCharacter:>"%c"}],
StringReplace[StringRiffle[#//.F[x___]:>{x}//Flatten,","],"i"->"o"]
]&/@gps//StringRiffle[#,"\n"]&;
src="#include <stdio.h>
#include <math.h>
#include <string.h>
#include <time.h>
#define NUM 100
#define N 6
#define EPS 1e-8
char ops[N] = {'+', '-', '*', '/', '#', '@'};
char buffer[128];
double F(double x, int op, double y) {
double arr[] = {x + y, x - y, x * y, x / y, 10 * x + y, -x + y};
return arr[op];
}
int check1(double x) {
return fabs(x - NUM) < EPS || fabs(x + NUM) < EPS;
}
int check2(char buffer[]) {
return (!strstr(buffer, \"#(\") && !strstr(buffer, \")#\"));
}
void calc(int a[]) {
int len = 0;
int n1 = a[0], n2 = a[1], n3 = a[2], n4 = a[3], n5 = a[4], n6 = a[5], n7 = a[6], n8 = a[7], n9 = a[8];
#pragma omp parallel for schedule(dynamic) reduction(+:buffer)
for (int i1 = 0; i1 < N; i1++) {
for (int i2 = 0; i2 < N; i2++)
for (int i3 = 0; i3 < N; i3++)
for (int i4 = 0; i4 < N; i4++)
for (int i5 = 0; i5 < N; i5++)
for (int i6 = 0; i6 < N; i6++)
for (int i7 = 0; i7 < N; i7++)
for (int i8 = 0; i8 < N; i8++) {
char o1 = ops[i1], o2 = ops[i2], o3 = ops[i3], o4 = ops[i4], o5 = ops[i5], o6 = ops[i6], o7 = ops[i7], o8 = ops[i8];
``
}
}
}
int main() {
clock_t t0 = clock();
freopen(\"output.txt\", \"w\", stdout);
int a[] = {1,2,3,4,5,6,7,8,9};
calc(a);
printf(\"Elapsed time: %g sec\\n\", (clock() - t0) / (double) CLOCKS_PER_SEC);
return 0;
}
";
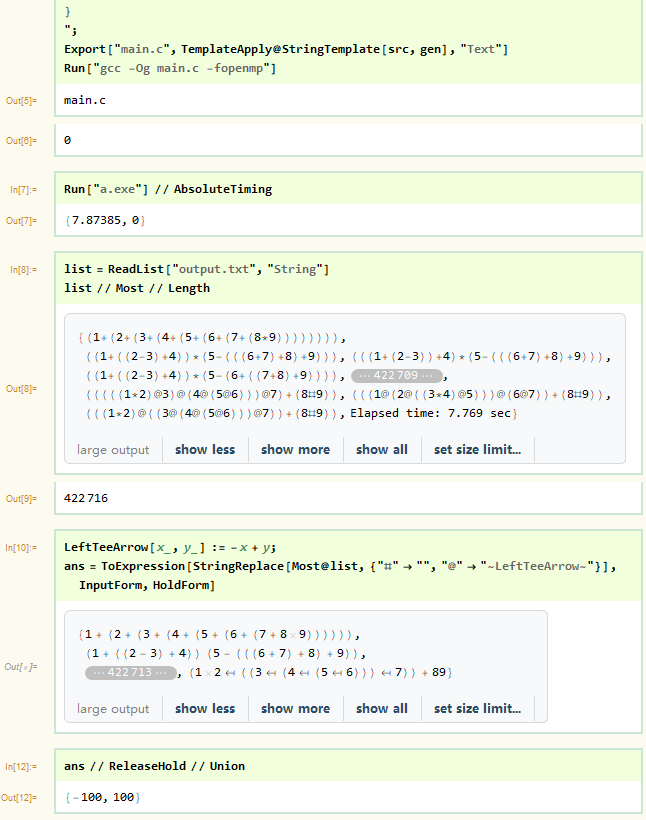
Export["main.c",TemplateApply@StringTemplate[src,gen],"Text"]
Run["gcc -Og main.c -fopenmp"]
Run["a.exe"] // AbsoluteTiming
list=ReadList["output.txt","String"]
list // Most // Length
LeftTeeArrow[x_,y_]:=-x+y;
ans=ToExpression[StringReplace[Most@list,{"#"->"","@"->"~LeftTeeArrow~"}],InputForm,HoldForm]
ReleaseHold[ans]//Union